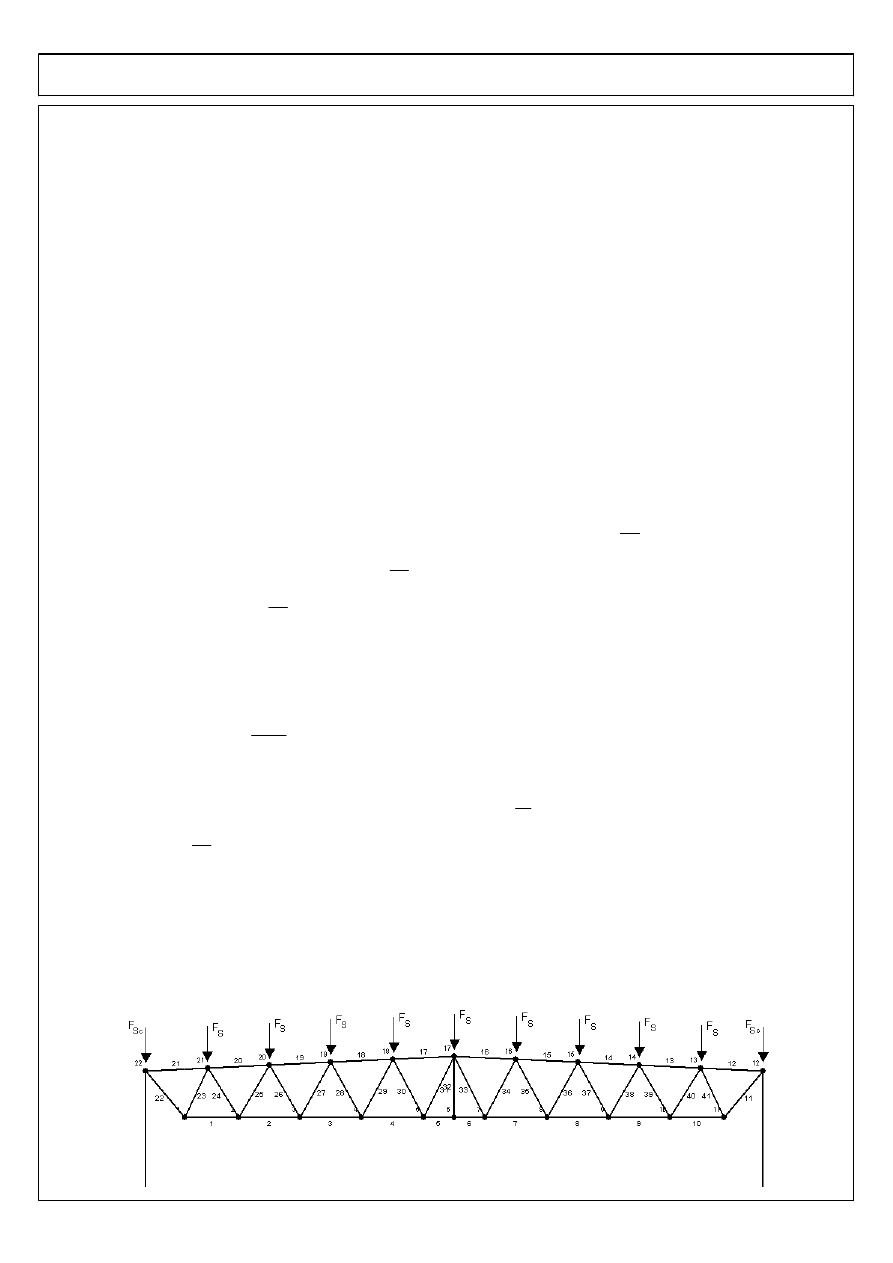
STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

A. Data:

span of the girder:

the level of the head of the column:

the spacing of main columns:

length of the hall:

the cover:

steel:

localization:

L

27m

:=

H

13.5m

:=

a

6m

:=

A

78m

:=

sandwich panel

S355

Warszawa

1. Composition of loads

1.1 Permanent actions - scheme 1

a) self-weight of girder and roof structure:

Gg

7.69kN

:=

(indirect node)

Ggc

4.7kN

:=

(extreme node)

b) self-weight of walls:

self-weight of sandwich panel

BALEXTHERM-PU-W-ST 100mm

gk

0.124

kN

m

2

:=

Z shape 20x20x2:

gz

0.003

kN

m

:=

G0

gk a

⋅

gz

+

0.747

kN

m

⋅

=

:=

eccentricity of the load of covering:

e0

0.5 100

⋅

mm

200mm

+

0.5 300

⋅

mm

+

0.4 m

=

:=

bending moment evenly distributed on the length of column:

M0

G0 e0

⋅

0.299

kN m

⋅

m

⋅

=

:=

c) self-weight of the column:

Initially was accepted I-section HEB300 with the self-weight

gs

117

kg

m

:=

Gs

1.17

kN

m

:=

1.2 Variable loads

1.2.1 Snow load - scheme 2

Fs

11.66kN

:=

(indirect node)

Fsc

7.13kN

:=

(extreme node)

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

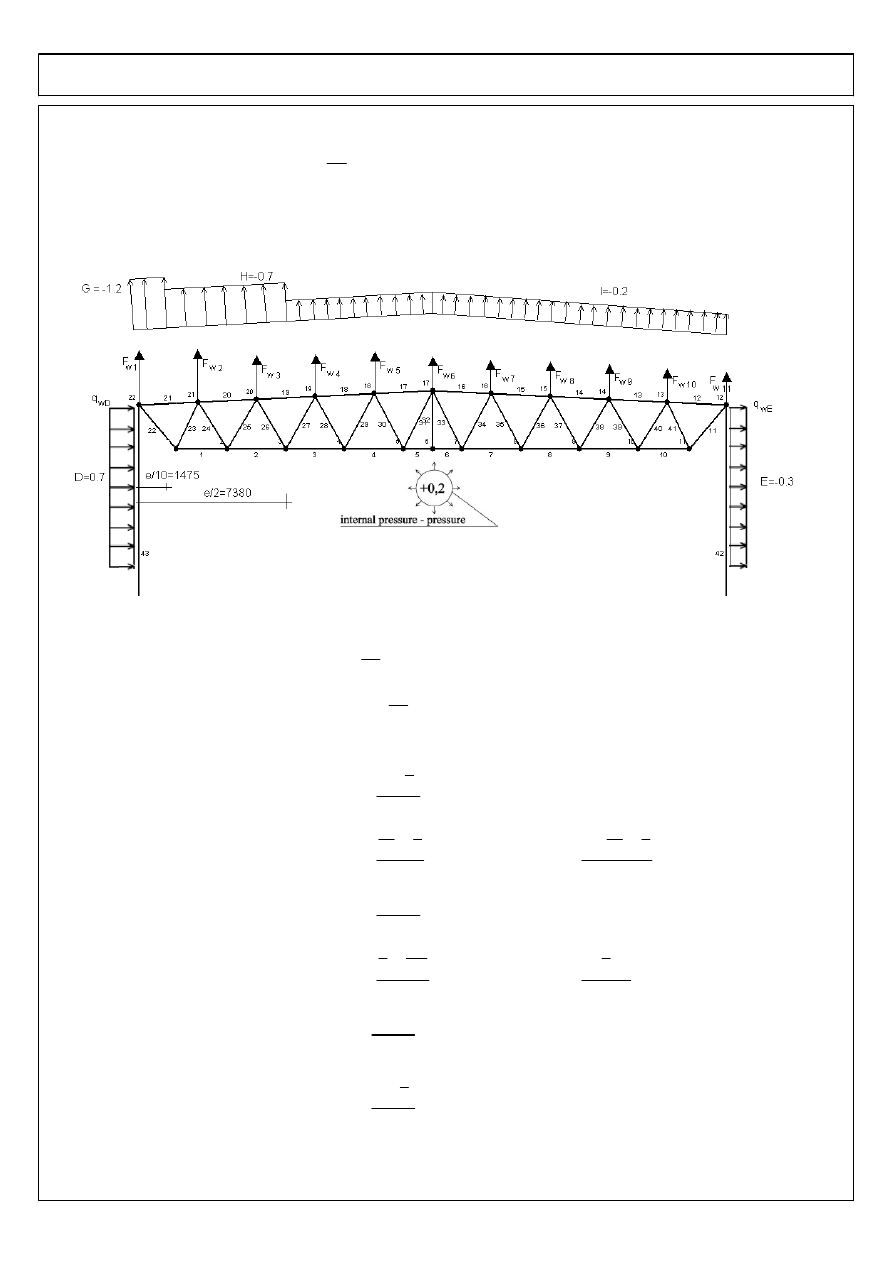
1.2.2 Wind load

peak velocity pressure:

qp

0.742

kN

m

2

:=

Systems of load

SYSTEM 1 - wind from the side

Scheme 3: wind from left side and internal pressure

Values of load distributed over walls:

qwD3

0.85 cs cd

⋅

qp

⋅

0.7

⋅

wiP

−

(

)

a

⋅

1.892

kN

m

⋅

=

:=

qwE3

0.85 cs cd

⋅

qp

⋅

0.3

−

(

)

⋅

wiP

−





a

⋅

1.892

−

kN

m

⋅

=

:=

Values of resultant forces concentrated in nodes of upper chord:

Fw13

cs cd

⋅

weG

⋅

wiP

−

(

)

c

b

2

+

cos

α

( )

l

⋅

















⋅

10.297

−

kN

⋅

=

:=

node 22

Fw23

cs cd

⋅

weG

⋅

wiP

−

(

)

e

10

b

2

−

cos

α

( )

l

⋅

















⋅

cs cd

⋅

weH

⋅

wiP

−

(

)

b

e

10

−

b

2

+

cos

α

( )

l

⋅

















⋅

+

11.11

−

kN

⋅

=

:=

node 21

Fw33

cs cd

⋅

weH

⋅

wiP

−

(

)

b

cos

α

( )

l

⋅













⋅

10.832

−

kN

⋅

=

:=

node 20

Fw43

cs cd

⋅

weH

⋅

wiP

−

(

)

e

2

5 b

⋅

2

−

cos

α

( )

l

⋅

















⋅

cs cd

⋅

weI

⋅

wiP

−

(

)

b

2

cos

α

( )

l

⋅

















⋅

+

4.914

−

kN

⋅

=

:=

node 19

Fw53

cs cd

⋅

weI

⋅

wiP

−

(

)

b

cos

α

( )

l

⋅













⋅

4.814

−

kN

⋅

=

:=

nodes 18 - 13

Fw63

cs cd

⋅

weI

⋅

wiP

−

(

)

c

b

2

+

cos

α

( )

l

⋅

















⋅

2.942

−

kN

⋅

=

:=

node 12

Scheme 4: wind from right side and internal pressure

Mirror of scheme 3

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

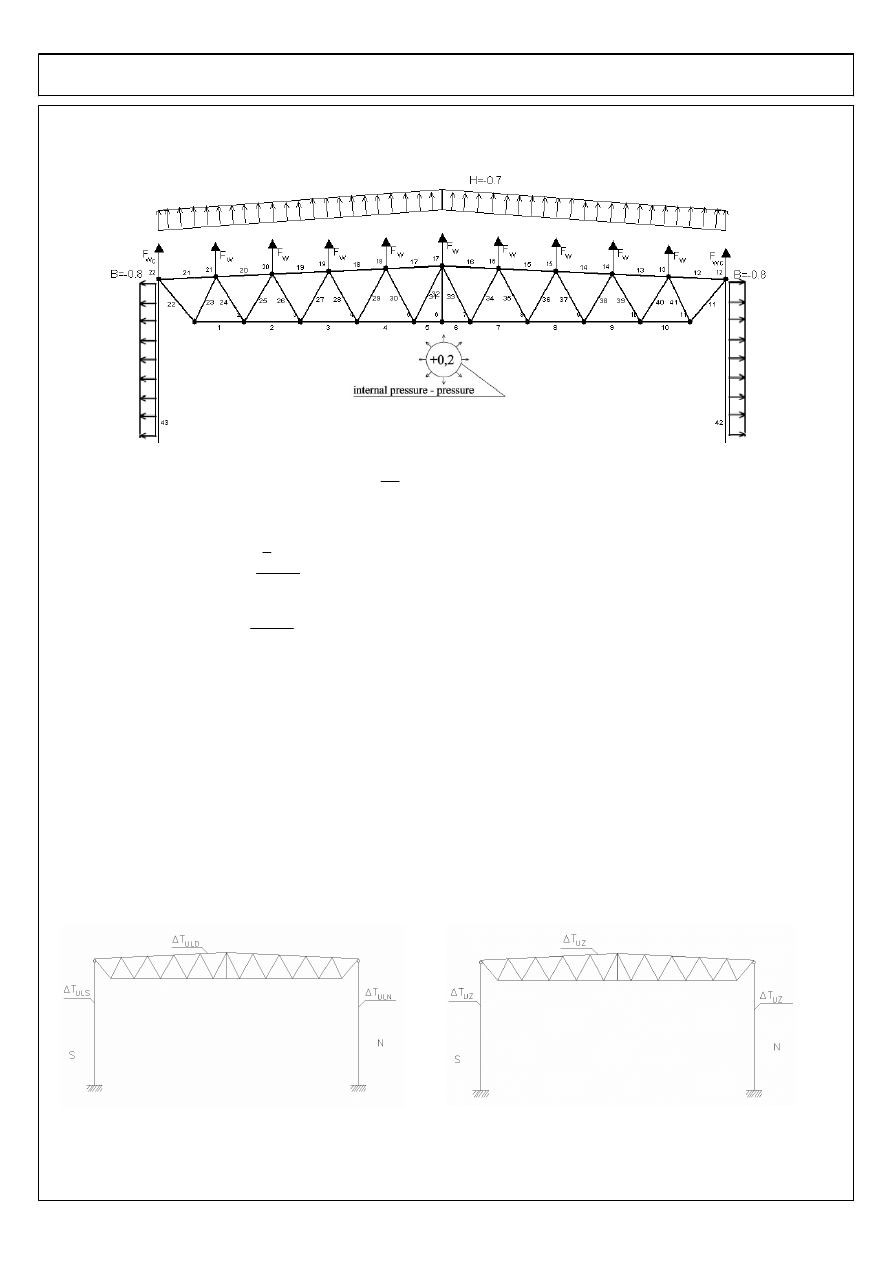
SYSTEM 2 - wind from the front

Scheme 5: wind from the front and internal pressure

Values of load distributed over walls:

qwB3

0.85 cs cd

⋅

qp

⋅

0.8

−

(

)

⋅

wiP

−





a

⋅

3.784

−

kN

m

⋅

=

:=

Resultant concentrated forces in nodes of upper chord:

Fwc5

cs cd

⋅

weH

⋅

wiP

−

(

)

b

2

c

+

cos

α

( )

l

⋅

















⋅

6.619

−

kN

⋅

=

:=

Fw5

cs cd

⋅

weH

⋅

wiP

−

(

)

b

cos

α

( )

l

⋅













⋅

10.832

−

kN

⋅

=

:=

1.2.3 Thermal load (first part of project)

The uniform component of the temperature:

- in summer for elements and surfaces situated from the north-east side

∆TULN

23deg

:=

- in summer for elements and surfaces situated from the south-west side

∆TULS

37deg

:=

- summer for the roof

∆TULD

43deg

:=

- in winter for the all construction

∆TUZ

9.5

−

deg

:=

Scheme 6: the thermal load in summer

Scheme 7: the thermal load in winter

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

RM WIN

2. Column

2.1 Combination of actions

COMBINATION 1 - dimensioning the column (dead load, wind action from right side, snow load)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

γQ2 ψQ2

⋅

Qk2

⋅

+

NEd

1.35 40.8

⋅

kN

1.5 26.4

⋅

kN

+

1.5 0.5

⋅

59.5

⋅

kN

+

139.31 kN

⋅

=

:=

MEd

1.35 7.5

⋅

kNm

1.5 43.1

⋅

kNm

+

1.5 0.5

⋅

0.4

⋅

kNm

+

75.08 kNm

⋅

=

:=

VEd

1.35 0.3

⋅

kN

1.5 12.8

⋅

kN

+

1.5 0.5

⋅

0

⋅

kN

+

19.61 kN

⋅

=

:=

COMBINATION 2 - maximum bending moment (dead load, wind action from left side, snow load, winter)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

γQi ψQi

⋅

Qki

⋅

(

)

∑

+

NEd

1.35 40.8

⋅

kN

1.5 26.4

⋅

kN

+

1.5 0.5

⋅

59.5

⋅

kN

+

1.5 0.6

⋅

0

⋅

kN

+

139.31 kN

⋅

=

:=

MEd

1.35 7.5

⋅

kNm

1.5 43.1

⋅

kNm

+

1.5 0.5

⋅

0.4

⋅

kNm

+

1.5 0.6

⋅

1.3

⋅

kNm

+

76.25 kNm

⋅

=

:=

VEd

1.35 0.3

⋅

kN

1.5 12.8

⋅

kN

+

1.5 0.5

⋅

0

⋅

kN

+

1.5 0.6

⋅

0.1

⋅

kN

+

19.7 kN

⋅

=

:=

COMBINATION 3 - maximum axial force (dead load, snow load)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

NEd

1.35 40.8

⋅

kN

1.5 59.5

⋅

kN

+

144.33 kN

⋅

=

:=

MEd

1.35 7.5

⋅

kNm

1.5 0.4

⋅

kNm

+

10.72 kNm

⋅

=

:=

VEd

1.35 0.3

⋅

kN

1.5 0

⋅

kN

+

0.41 kN

⋅

=

:=

COMBINATION 4 - maximum shearing force (dead load min, wind action from the front)

γGjinf Gkjinf

⋅

γQ1 Qk1

⋅

+

NEd

1.0 40.2

⋅

kN

1.5 55.3

⋅

kN

+

123.15 kN

⋅

=

:=

MEd

1.0 0.1

⋅

kNm

1.5 0

⋅

kNm

+

0.1 kNm

⋅

=

:=

VEd

1.0 0.3

⋅

kN

1.5 53.1

⋅

kN

+

79.95 kN

⋅

=

:=

ROBOT:

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

2. Column

2.1 Combination of actions

COMBINATION 1 - dimensioning the column (dead load, wind action from right side, snow load)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

γQ2 ψQ2

⋅

Qk2

⋅

+

NEd

1.35 54.26

⋅

kN

1.5 47.15

⋅

kN

+

1.5 0.5

⋅

59.6

⋅

kN

+

188.68 kN

⋅

=

:=

MEd

1.35 10.83

⋅

kNm

1.5 116.2

⋅

kNm

+

1.5 0.5

⋅

13.71

⋅

kNm

+

199.2 kNm

⋅

=

:=

VEd

1.35 8.04

⋅

kN

1.5 36.15

⋅

kN

+

1.5 0.5

⋅

10.83

⋅

kN

+

73.2 kN

⋅

=

:=

COMBINATION 2 - maximum bending moment (dead load, wind action from left side, snow load, winter)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

γQi ψQi

⋅

Qki

⋅

(

)

∑

+

NEd

1.35 54.26

⋅

kN

1.5 47.15

⋅

kN

+

1.5 0.5

⋅

59.6

⋅

kN

+

1.5 0.6

⋅

0.18

⋅

kN

+

188.84 kN

⋅

=

:=

MEd

1.35 10.83

⋅

kNm

1.5 116.2

⋅

kNm

+

1.5 0.5

⋅

13.7

⋅

kNm

+

1.5 0.6

⋅

4.1

⋅

kNm

+

202.89 kNm

⋅

=

:=

VEd

1.35 8.04

⋅

kN

1.5 36.15

⋅

kN

+

1.5 0.5

⋅

10.83

⋅

kN

+

1.5 0.6

⋅

4.09

⋅

kN

+

76.88 kN

⋅

=

:=

COMBINATION 3 - maximum axial force (dead load, snow load)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

NEd

1.35 54.26

⋅

kN

1.5 59.6

⋅

kN

+

162.65 kN

⋅

=

:=

MEd

1.35 10.83

⋅

kNm

1.5 13.71

⋅

kNm

+

35.19 kNm

⋅

=

:=

VEd

1.35 8.04

⋅

kN

1.5 10.83

⋅

kN

+

27.1 kN

⋅

=

:=

COMBINATION 4 - maximum shearing force (dead load min, wind action from the front)

γGjinf Gkjinf

⋅

γQ1 Qk1

⋅

+

NEd

1.0 54.26

⋅

kN

1.5 55.35

⋅

kN

+

137.28 kN

⋅

=

:=

MEd

1.0 10.83

⋅

kNm

1.5 36.71

⋅

kNm

+

65.89 kNm

⋅

=

:=

VEd

1.0 8.04

⋅

kN

1.5 20.65

⋅

kN

+

39.01 kN

⋅

=

:=

ROBOT COMB:

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

2. Column

2.1 Combination of actions

COMBINATION 1 - dimensioning the column (dead load, wind action from right side, snow load)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

γQ2 ψQ2

⋅

Qk2

⋅

+

=

1.35 Gkjsup

⋅

1.5 Qk1

⋅

+

1.5 0.5

⋅

Qk2

⋅

+

NEd1

84.82kN

:=

MEd1

173.81kNm

:=

VEd1

41.12kN

:=

COMBINATION 2 - maximum bending moment (dead load, wind action from left side, snow load, winter)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

γQi ψQi

⋅

Qki

⋅

(

)

∑

+

=

1.35 Gkjsup

⋅

1.5 Qk1

⋅

+

1.5 0.5

⋅

Qk1

⋅

+

1.5 0.6

⋅

Qk2

⋅

+

NEd2

85.26kN

:=

MEd2

182.5kNm

:=

VEd2

42.11kN

:=

COMBINATION 3 - maximum axial force (dead load, snow load)

γGjsup Gkjsup

⋅

γQ1 Qk1

⋅

+

=

1.35 Gkjsup

⋅

1.5 Qk1

⋅

+

NEd3

162.65kN

:=

MEd3

35.19kNm

:=

VEd3

7.64kN

:=

COMBINATION 4 - maximum shearing force (dead load min, wind action from the front)

γGjinf Gkjinf

⋅

γQ1 Qk1

⋅

+

=

1.0 Gkjinf

⋅

1.5 Qk1

⋅

+

NEd4

28.77kN

:=

MEd4

65.89kNm

:=

VEd4

33.07kN

:=

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

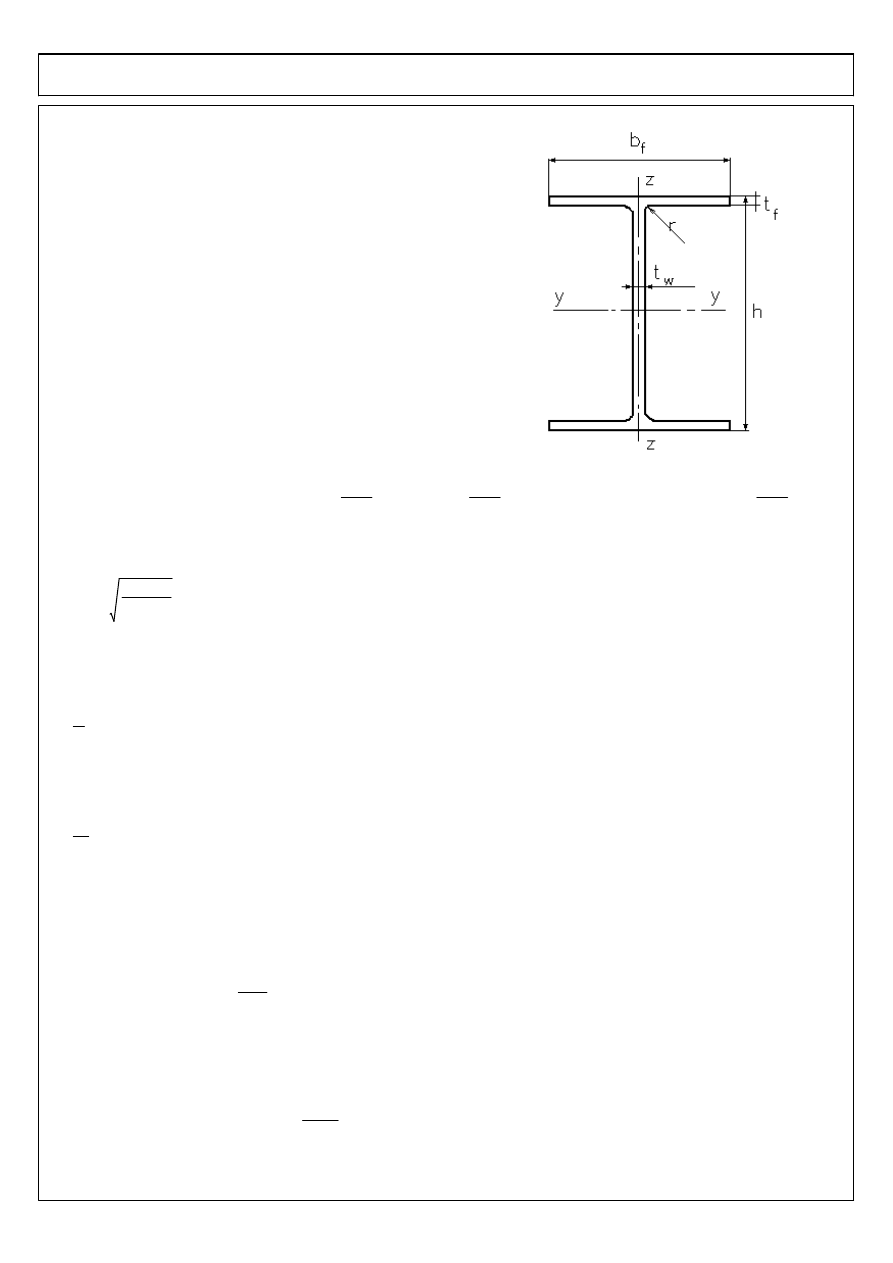
2.2 Design of the column

Initially accepted HEB 280

A

131cm

2

:=

Iz

6590cm

4

:=

iy

12.13cm

:=

h

280mm

:=

Iτ

144cm

4

:=

iz

7.09cm

:=

bf

280mm

:=

tf

18mm

:=

Iω

1130000cm

6

:=

tw

11mm

:=

Wply

1530.08cm

3

:=

r

24mm

:=

2.2.1 Class of section

Mechanical properties of the steel:

tf

18 mm

⋅

=

< 40mm

fy

355

N

mm

2

:=

fu

510

N

mm

2

:=

E

210GPa

:=

G

81000

N

mm

2

:=

The class of eccentrically compressed elements should be defined accordingly for the stress distribution arising at the
considered combination of loads - for this instance to the axial compression.

ε

235MPa

fy

0.814

=

:=

Flange in compression:

c

0.5 bf tw

−

(

)

⋅

r

−

:=

c

tf

6.139

=

<

9

ε

⋅

7.323

=

class 1

Web in bending and compression:

d

h

2 tf

⋅

−

2 r

⋅

−

:=

d

tw

17.818

=

<

38

ε

⋅

30.917

=

class 1

Section's class: 1

2.2.2 Resistance of cross section

a) compression

γM0

1.0

:=

NcRd

A fy

⋅

γM0

4.651

10

3

×

kN

⋅

=

:=

Resistance condition:

Combination of loads for N

max

(3):

Nmax

NEd3

162.65 kN

⋅

=

:=

Nmax
NcRd

0.035

=

<1

condition fulfilled

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

b) bending

The influence of the axial power on the plastic resistance of the section in bending.

- combination of loads for M

max

(2):

Mmax

MEd2

182.5 kNm

⋅

=

:=

NEd

NEd2

85.26 kN

⋅

=

:=

- the condition for the omission of the influence of the axial force on the plastic resistance of the section in bending in
relation to the y axis:

NEd

85.26 kN

⋅

=

<

0.25 NplRd

⋅

0.25 NcRd

⋅

1162.6 kN

⋅

=

:=

0.25

NEd

85.26 kN

⋅

=

<

0.5 d

⋅

tw

⋅

fy

⋅

γM0

382.69 kN

⋅

=

We can omit the influence of the axial force on the plastic resistance in bending.

The design bending resistance:

MplyRd

Wply fy

⋅

γM0

543.178 kNm

⋅

=

:=

Resistance condition:

Mmax

MplyRd

0.336

=

<1

condition fulfilled

c) shearing

The shearing resistance of the section

- area of flange:

Af

bf tf

⋅

5040 mm

2

⋅

=

:=

- area of the web:

Aw

d tw

⋅

2156 mm

2

⋅

=

:=

- effective shearing area:

Avz1

A

2 bf

⋅

tf

⋅

−

tw 2r

+

(

)

tf

⋅

+

4082 mm

2

⋅

=

:=

η

1

:=

Avz2

η Aw

⋅

2156 mm

2

⋅

=

:=

Avz

max Avz1 Avz2

,

(

)

4082 mm

2

⋅

=

:=

Af

Aw

2.338

=

>0.6

resistance of section:

VcRd

Aw

fy

3

γM0

⋅

⋅

441.892 kN

⋅

=

:=

resistance condition:

Combination of loads for N

max

(3):

Vmax

VEd4

33.07 kN

⋅

=

:=

Vmax
VcRd

0.075

=

<1

condition fulfilled

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

2.2.3 Buckling resistance of the column

Internal forces: design of column carried out for the combination of actions 2:

NEd

NEd2

85.26 kN

⋅

=

:=

MEd

MEd2

182.5 m kN

⋅

=

:=

VEd

VEd2

42.11 kN

⋅

=

:=

2.2.3.1 Buckling lengths

L - length of column in given plane of buckling

µ

- coefficient of buckling

a) length of the buckling in y axis:

Ly

H

13.5 m

=

:=

μy

1.5

:=

- partially fixed

Lcry

Ly μy

⋅

20.25 m

=

:=

b) length of the buckling in z axis:

Lz

H

2

6.75 m

=

:=

-spandrel beam

μz

1.0

:=

- pinned connection

Lcrz

Lz μz

⋅

6.75 m

=

:=

2.2.3.2 Buckling coefficient

a) length of the buckling in y axis (in plane of lateral system)

the reference value to appointing of the relative slenderness:

λ1

π

E

fy

⋅

:=

=

λ1

93.9

ε

⋅

76.399

=

:=

the relative slenderness in y axis:

λy

A fy

⋅

Ncr

:=

Ncr

=

λy

Lcry

iy

1

λ1

⋅

2.185

=

:=

imperfection factor:

αy

0.76

:=

parameter of curve of instability:

Φy

0.5 1

αy λy 0.2

−

(

)

⋅

+

λy

2

+









⋅

3.642

=

:=

buckling coefficient:

χy

1

Φy

Φy

2

λy

2

−

+

0.153

=

:=

b) length of the buckling in z axis (out of plane of lateral system)

the relative slenderness in y axis:

λz

A fy

⋅

Ncr

:=

Ncr

=

λz

Lcrz

iz

1

λ1

⋅

1.246

=

:=

imperfection factor:

αz

0.76

:=

parameter of curve of instability:

Φz

0.5 1

αz λz 0.2

−

(

)

⋅

+

λz

2

+









⋅

1.674

=

:=

buckling coefficient:

χz

1

Φz

Φz

2

λz

2

−

+

0.358

=

:=

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

2.2.3.3 Lateral buckling coefficient

The correction coefficient C

1

- accepted triangular distribution of bending moments

ψ

0

:=

k

1

:=

C1

1.879

:=

Critical moment M

cr

at the lateral buckling

Mcr

C1

π

2

E

⋅

Iz

⋅

Lz

2

⋅

Iω

Iz

Lz

2

G

⋅

Iτ

⋅

π

2

E

⋅

Iz

⋅

+

⋅

1333.6 kNm

⋅

=

:=

Relative slenderness at lateral buckling:

λLT

Wply fy

⋅

Mcr

0.638

=

:=

Parameter of curve of the lateral buckling:

λLT0

0.4

:=

β

0.75

:=

αLT

0.34

:=

ΦLT

0.5 1

αLT λLT λLT0

−

(

)

⋅

+

β λLT

( )

2

⋅

+









⋅

0.693

=

:=

The coefficient of lateral buckling:

χLT1

1

λLT

2

2.455

=

:=

χLT2

1

ΦLT

ΦLT

2

β λLT

2

⋅

−

+

0.9

=

:=

χLT

min

χLT1 χLT2

,

1

,

(

)

0.9

=

:=

2.2.3.4 Interaction factors

Coefficient of equivalent constant moment

Cmy

0.9

:=

- sway buckling mode

γM1

1

:=

NRk

A fy

⋅

4.651

10

3

×

kN

⋅

=

:=

kyy1

Cmy 1

λy 0.2

−

(

)

NEd

χy

NRk
γM1

⋅

⋅

+





















⋅

1.115

=

:=

kyy2

Cmy 1 0.8

NEd

χy

NRk
γM1

⋅

⋅

+





















⋅

0.987

=

:=

kyy

min kyy1 kyy2

,

(

)

0.987

=

:=

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

The coefficient C

mLT

at the triangular distribution of bending moments for

ψ

=0

CmLT

0.6

0.4

ψ

⋅

+

0.6

=

:=

>0.4

kzy1

1

0.1

λz

⋅

CmLT 0.25

−

NEd

χz

NRk
γM1

⋅

⋅

−

0.982

=

:=

kzy2

1

0.1

CmLT 0.25

−

NEd

χz

NRk
γM1

⋅

⋅

−

0.985

=

:=

kzy

max kzy1 kzy2

,

(

)

0.985

=

:=

2.2.3.5 Resistance check of column

∆MyEd

0

:=

MzEd

0

:=

∆MzEd

0

:=

MyRk

Wply fy

⋅

543.178 kNm

⋅

=

:=

MyEd

MEd

182.5 kNm

⋅

=

:=

NEd

χy NRk

⋅

γM1













kyy

MyEd

χLT

MyRk

γM1

⋅

⋅

+

0.489

=

< 1

NEd

χz NRk

⋅

γM1













kzy

MyEd

χLT

MyRk

γM1

⋅

⋅

+

0.419

=

< 1

conditions are fulfilled

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

2.2.4 Head of column

a) The load on the level of the head of the column:
The autorithative combination for the maximum value of the axial force in the top joint of the column
COMB 5 (dead load, snow load, winter):

Nmaxg

1.35 54.26

⋅

kN

1.5 59.6

⋅

kN

+

1.5 0.6

⋅

0.18

⋅

kN

+

162.81 kN

=

:=

b) assumptions:
Dimensions of the horizontal metal plate covering the column:

length:

lbg

h

2 20

⋅

mm

+

320 mm

=

:=

width:

bbg

max bf 2 20

⋅

mm

+

240mm

,

(

)

320 mm

=

:=

thickness:

tbg

16mm

:=

Dimensions of the rib:

thickness:

tg

16mm

:=

width:

bg

bbg

320 mm

=

:=

height:

hg

lbg

320 mm

=

:=

c) Welds connecting rib with the column

The thickness accepted from constructional conditions according to PN-90-03200:

anom

4mm

:=

>

0.2 tg

⋅

3.2 mm

=

anom

4mm

:=

<

0.7 tw

⋅

7.7 mm

=

anom

4mm

:=

>

2.5mm

anom

4mm

:=

<

16mm

The design resistance of the fillet weld

βw

0.8

:=

γM2

1.25

:=

fvwRd

fu

3

βw

⋅

γM2

⋅

294.449

N

mm

2

=

:=

The design resistance of fillet weld per unit length:

FwRd

fvwRd anom

⋅

1177.79

N

mm

=

:=

The required length of the weld:

lw.req

Nmaxg

4 FwRd

⋅

35 mm

=

:=

Accepted length of weld:

lw

max lw.req hg

,

(

)

320 mm

=

:=

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

c) The pressure to the web and rib

The range of pressure area:

width of bearing:

bc

80mm

:=

s

bc 2 tbg

⋅

+

112 mm

=

:=

The area of the pressure to web and rib:

Ag

bg tg

⋅

tw s tg

−

(

)

⋅

+

6176 mm

2

=

:=

The design resistance at load by axial force:

NcRd

Ag fy

⋅

γM0

2192.5 kN

=

:=

Resistance condition:

Nmaxg

NcRd

0.074

=

The resistance condition is fulfilled

Vertical displacement of post head

Udop

H

150

90 mm

⋅

=

:=

Up

85mm

:=

- from ROBOT

Up

85 mm

⋅

=

<

Udop

90 mm

⋅

=

condition is satisfied

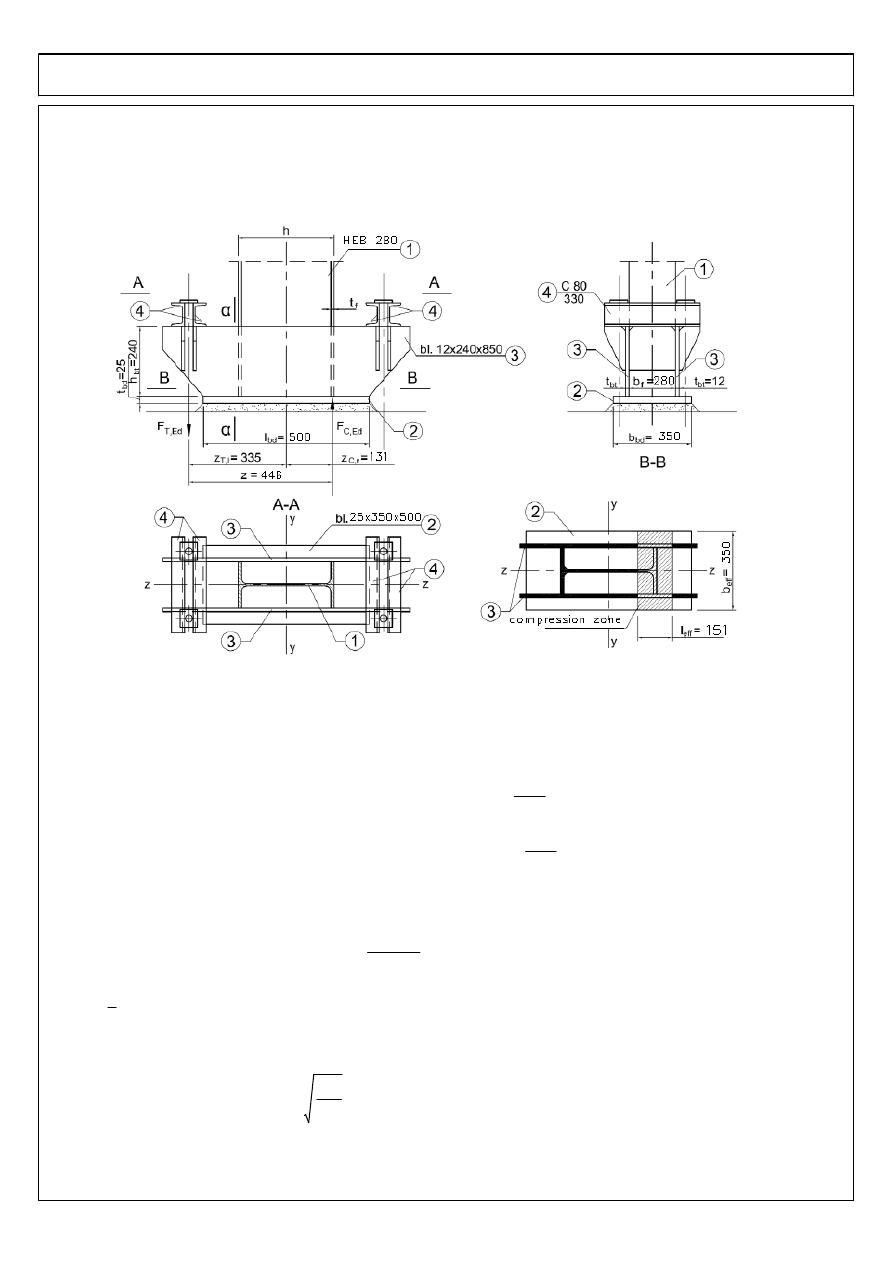
STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

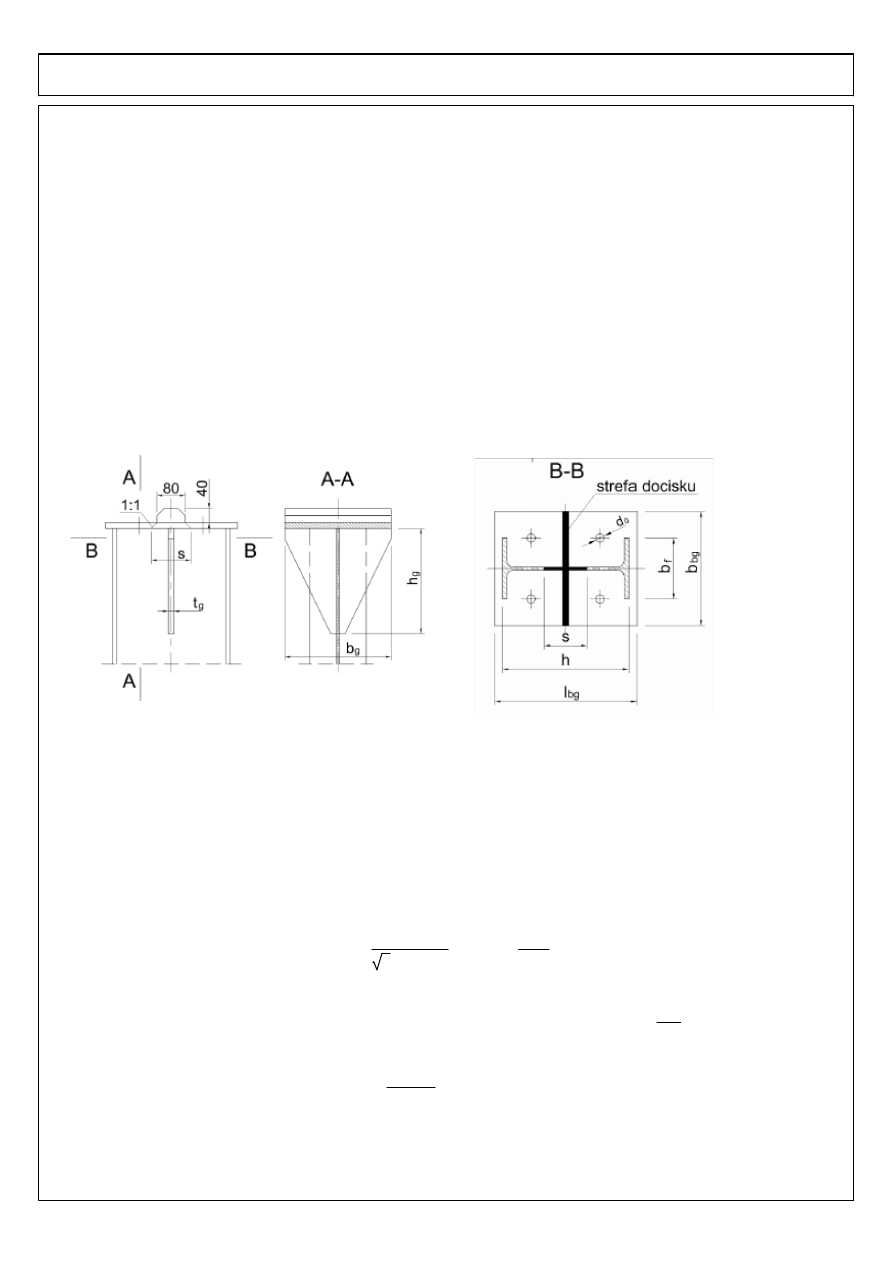
2.2.5 Column base

a) Assumptions

The geometry of the column base:

b) The resistance of the concrete

Assumptions:

class of concrete of foundations C25/30

fck

25

N

mm

2

:=

characteristic resistance of the concrete in compression

design resistance of the concrete in the compression

fcd

16.7

N

mm

2

:=

The design resistance of the connection in the pressure:

fjd

βj FRdu

⋅

beff leff

⋅

:=

βj

βj

2

3

:=

material coefficient of the foundation

The design resistance at the concentrated force acting on the concrete surface:

FRdu

Ac0 fcd

⋅

Ac1
Ac0

⋅

:=

Ac0

<

3 fcd

⋅

Ac0

⋅

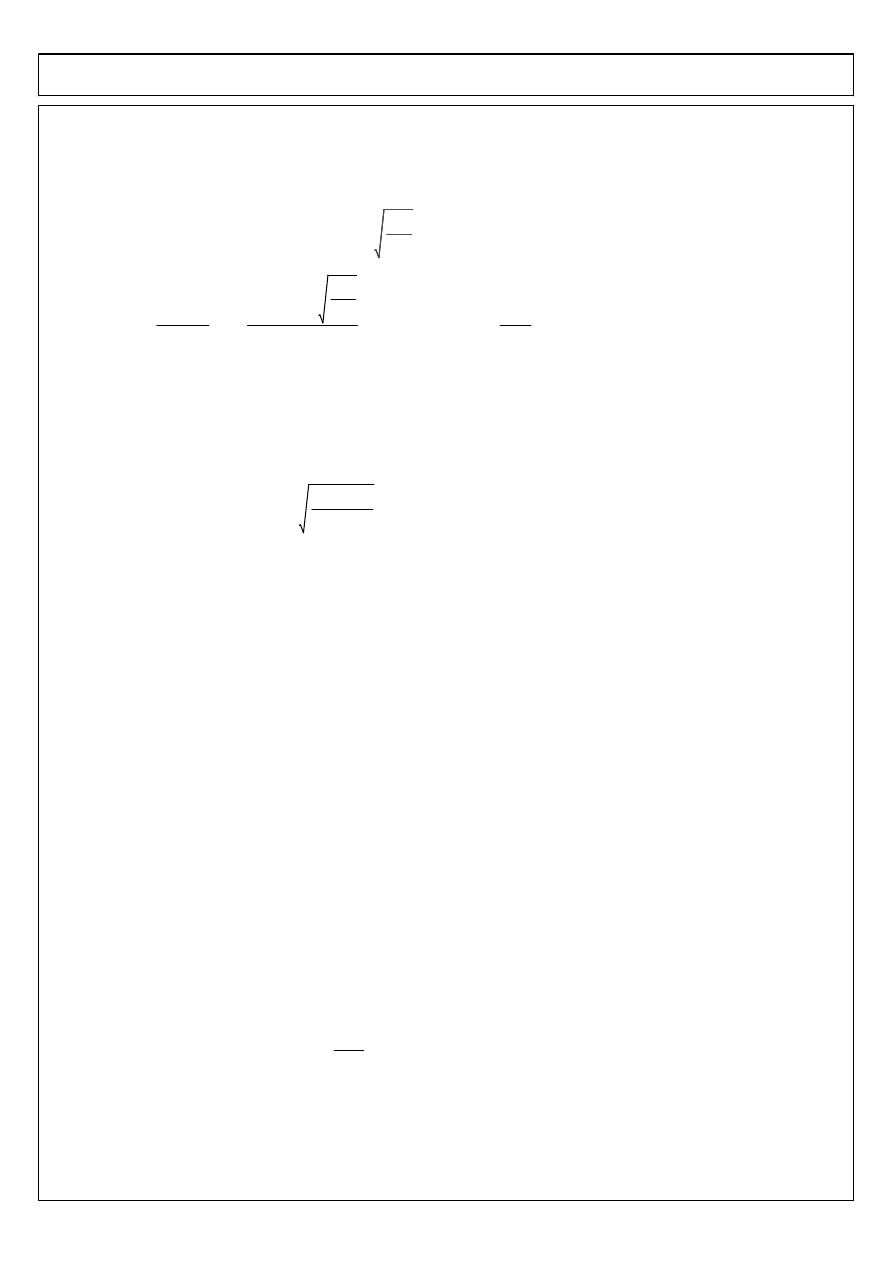
STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

area of pressure

Ac0

beff leff

⋅

:=

beff

beff

leff

effective width and length of the T-stub of flange and basis metal plate

Ac1

the area of distribution;

Ac1
Ac0

1.5

:=

Ac1
Ac0

fjd

βj FRdu

⋅

beff leff

⋅

:=

FRdu

=

βj Ac0

⋅

fcd

⋅

Ac1
Ac0

⋅

Ac0

=

1.5

βj

⋅

fcd

⋅

16.7

N

mm

2

=

c) Resistance condition of the compressed zone:

The maximum outreach of the pressure zone:

tp

25mm

:=

thickness of base plate

γm0

1

:=

c

tp

fy

3 fjd

⋅

γm0

⋅

⋅

67 mm

=

:=

The effective width b

eff

and length l

eff

of the T-stub of basis metal plate:

bdb

350mm

:=

tbt

12mm

:=

beff

min bdb bf 2 c tbt

+

(

)

⋅

+

,





350 mm

=

:=

lbd

500mm

:=

leff

min 0.5 lbd h

−

(

)

⋅

tf

+

c

+

tf 2 c

⋅

+

,





151 mm

=

:=

The design resistnace of compressed zone:

FcRd

fjd leff

⋅

beff

⋅

883.2 kN

=

:=

the level arm z at the dominant bending moment:

zTI

335mm

:=

zCr

0.5 h

tf

−

(

)

⋅

131 mm

=

:=

z

zTI zCr

+

466 mm

=

:=

the axial force:

The authoritative combination of loads COMB2

NEd

NEd2

85.26 kN

=

:=

MEd

MEd2

182.5 kNm

=

:=

FCEd

NEd

−

MEd

z

−

476.891

−

kN

=

:=

FCEd

476.9

−

kN

=

<

FcRd

883.2 kN

=

The resistance condition is fulfilled

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

d) The design resistance of tensioned zone

Accepted anchors ŁT30180 from steel S355

As

707mm

2

:=

fub

470

N

mm

2

:=

The carrying capacity of one anchor:

k2

0.9

:=

FtRd

k2 fub

⋅

As

⋅

γM2

239.2 kN

=

:=

Accepted that the anchor was properly fastened in the concrete, no tearing out.

the load of tension zone

FTEd

NEd

−

MEd

z

+

306.371 kN

=

:=

FTEd

306.371 kN

=

<

FTRd

2 FtRd

⋅

478.498 kN

=

:=

The resistance condition is fulfilled

e) The load by shearing force:

The auhoritative combination of loads: COMB 4

Vmax

VEd4

33.07 kN

=

:=

For the purpose of the takeover of the shearing force, under the metal plate of basis, cantilever from section
IPE 100 steel 235 was designed.

The carrying capacity of the section in shearing:

Area of flange:

bf1

55mm

:=

tf1

5.7mm

:=

Af

bf1 tf1

⋅

313.5 mm

2

=

:=

Area of web:

d

100mm

2 7

⋅

mm

−

2 5.7

⋅

mm

−

74.6 mm

=

:=

tw

4.1mm

:=

Aw

d tw

⋅

305.86 mm

2

=

:=

Af

Aw

1.025

=

> 0.6

fy

235MPa

:=

VcRd

Aw

fy

3

γM0

⋅

⋅

41.498 kN

=

:=

Vmax
VcRd

0.797

=

< 1

The resistance condition is satisfied

f) The resistance of the vertical fillet welds connecting the column with trapezoidal plates

anom

5mm

:=

>

0.2 tbt

⋅

2.4 mm

=

anom

5mm

:=

<

0.7 tf

⋅

12.6 mm

=

anom

5mm

:=

>

2.5mm

anom

5mm

:=

<

16mm

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

The design resistance of the fillet welds

βw

0.8

:=

γM2

1.25

:=

fvwRd

fu

3

βw

⋅

γM2

⋅

294.449

N

mm

2

=

:=

The design resistance of fillet weld per unit length:

FwRd

fvwRd anom

⋅

1472.24

N

mm

=

:=

FcEd

355kN

:=

The required length of the weld:

lw.req

FcEd

4 FwRd

⋅

60 mm

=

:=

Accepted length of weld:

hbt

240mm

:=

lw

max lw.req hbt

,

(

)

240 mm

=

:=

g) The design resistance of the horizontal and trapezoidal metal plates:

The bending moment i nsection

α

-

α

MTEd

FTEd zTI

h

2

−













⋅

59.742 kNm

=

:=

The class of section:

web bending

c

hbt

:=

c

tbt

20

=

<

72

ε

⋅

58.58

=

class I

flange compression - cantilever part

c

0.5 bdb bf

−

(

)

⋅

tbt

−

0.023 m

=

:=

tbd

25mm

:=

c

tbd

0.92

=

<

9

ε

⋅

7.323

=

class I

flange compression - span part

c

bf

:=

c

tbd

11.2

=

<

33

ε

⋅

26.849

=

class I

I class of section

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

The design plastic resistance of two trapezoidal metal plates at shearing

the effective area at shearing

Avbt

2 hbt

⋅

tbt

⋅

5760 mm

2

=

:=

VplRd

Avbt fy

⋅

3

γM0

⋅

781.501 kN

=

:=

resistance condition:

FcEd

VplRd

0.454

=

< 1

The resistance condition is fulfilled

The design plastic resistance of two trapezoidal metal plates at bending

Because the condition

FcEd

VplRd

0.454

=

< 0.5

is fulfilled, there is no necessity of taking into account shearing
influence on the bending reistance.

geometric and endurance parameters of the section:

yc

bdb tbd

⋅

0.5

⋅

tbd

⋅

2 hbt

⋅

tbt

⋅

0.5 hbt

⋅

tbd

+

(

)

⋅

+

bdb tbd

⋅

2 hbt

⋅

tbt

⋅

+

65.1 mm

=

:=

Iy

bdb tbd

3

⋅

12

bdb tbd

⋅

yc 0.5 tbd

⋅

−

(

)

2

⋅

+

2

tbt hbt

3

⋅

12

tbt hbt

⋅

yc

tbd 0.5 hbt

⋅

+

(

)

−





2

⋅

+













⋅

+

8.908

10

7

×

mm

4

=

:=

Wpl1

Iy

tbd hbt

+

yc

−

4.456

10

5

×

mm

3

=

:=

Wpl2

Iy

yc

1.368

10

6

×

mm

3

=

:=

Wply

min Wpl1 Wpl2

,

(

)

4.456

10

5

×

mm

3

=

:=

the design resistance in bending

McRd

Wply fy

⋅

γM0

104.726 kNm

=

:=

The resistance condition:

MTEd
McRd

0.57

=

< 1

The resistance condition is fulfilled

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

h) The resistance of fillet welds connecting metal plate of the basis with trapezoidal plates

anom

6mm

:=

>

0.2 tbd

⋅

5 mm

=

anom

6mm

:=

<

0.7 tbt

⋅

8.4 mm

=

anom

6mm

:=

>

2.5mm

anom

6mm

:=

<

16mm

Total length of welds:

lsp

2 2 lbd

⋅

h

−

(

)

⋅

1440 mm

=

:=

Stresses in welds:

from axial force:

σ1

FcEd

anom 2

⋅

2 leff

⋅

tf

−

(

)

⋅

2

73.607

N

mm

2

=

:=

σ2

0.9 fu

⋅

γM2

367.2

N

mm

2

=

:=

σp

min

σ1 σ2

,

(

)

73.61

N

mm

2

=

:=

τp

σp

:=

from shearing force COMB2:

VEd2

42.11 kN

=

Vsp

VEd2

2 lbd h

−

(

)

⋅

lsp

⋅

12.867 kN

=

:=

τpl

Vsp

4 anom

2

⋅

89.354

N

mm

2

=

:=

The resistance condition of the welds:

σp

2

3

τp

2

τpl

2

+









⋅

+









0.5

213.599

N

mm

2

=

<

fu

βw γM2

⋅

510

N

mm

2

=

The resistance condition is fulfilled

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

kNm

kN m

⋅

:=

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

I case: pressure

cpiP

0.2

:=

II case: suction

cpiS

0.3

−

:=

Wind load on the girder:

on surface of the roof:

weG

qp

1.2

−

(

)

⋅

0.89

−

kN

m

2

⋅

=

:=

weH

qp

0.7

−

(

)

⋅

0.519

−

kN

m

2

⋅

=

:=

weI

qp

0.2

−

(

)

⋅

0.148

−

kN

m

2

⋅

=

:=

internal pressure:

wiP

qp cpiP

⋅

0.148

kN

m

2

⋅

=

:=

wiS

qp cpiS

⋅

0.223

−

kN

m

2

⋅

=

:=

cs

1

:=

cd

1

:=

c

0.3m

:=

α

2.86deg

:=

b

2.7m

:=

e

14.75 m

⋅

:=

l

6m

:=

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

STEEL INDUSTRIAL HALL - LATERAL SYSTEM DESIGN

fjd

1.5

βj

⋅

fcd

⋅

:=