Instrumentation system

design

Sources of error

• Insufficient knowledge of process

parameters & design conditions

• Poor design
• Change in process parameters,

irregularities, upsets etc.

• Poor maintenance
• Error caused by person operating the

instrument or equipment

• Certain design limitations

Dynamic characteristics

these are determined by subjecting its primary element to unknown

and predetermined variations in the measured quantity

• Common variation

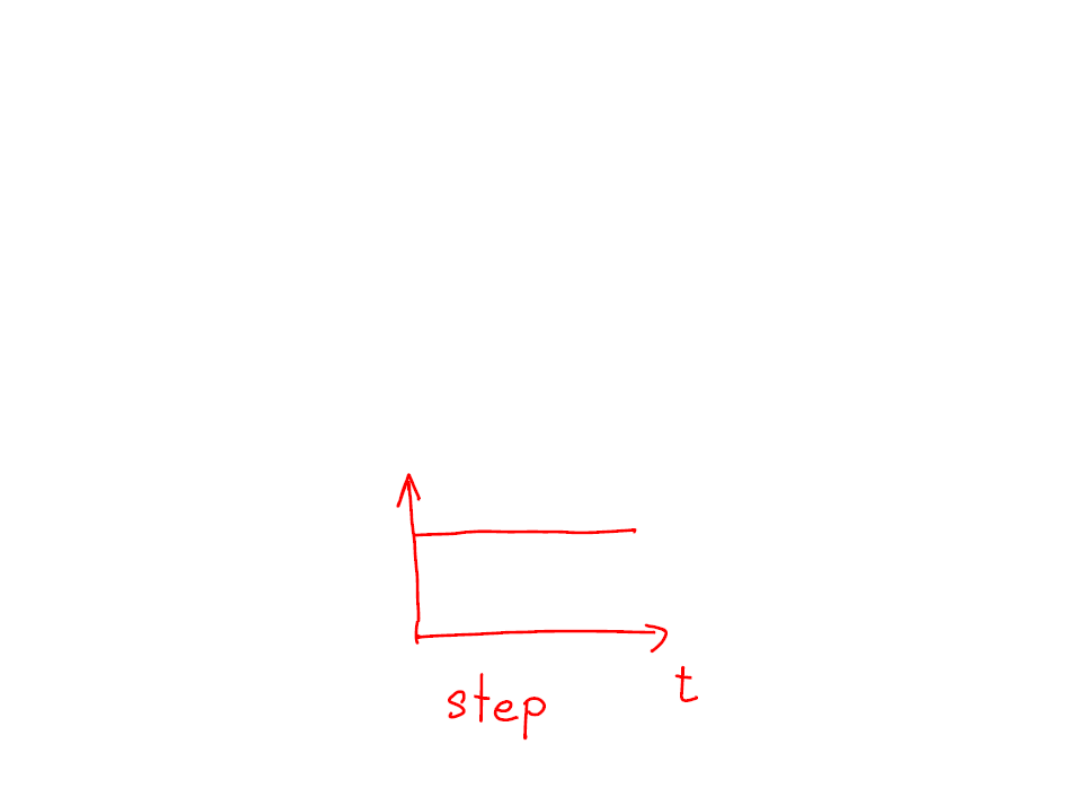
– Step change: primary element is

subjected to an instantaneous & finite
change in measured variable

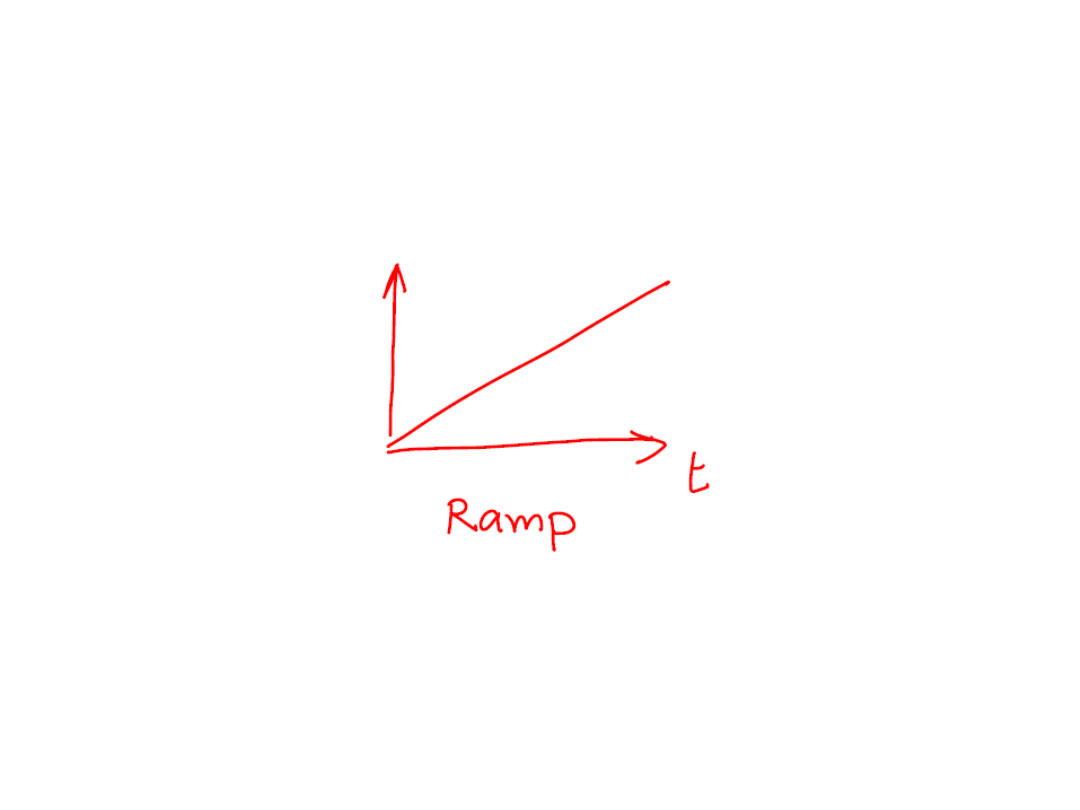
– Linear change: primary element is

following a measured variable, changing
linearly with time.

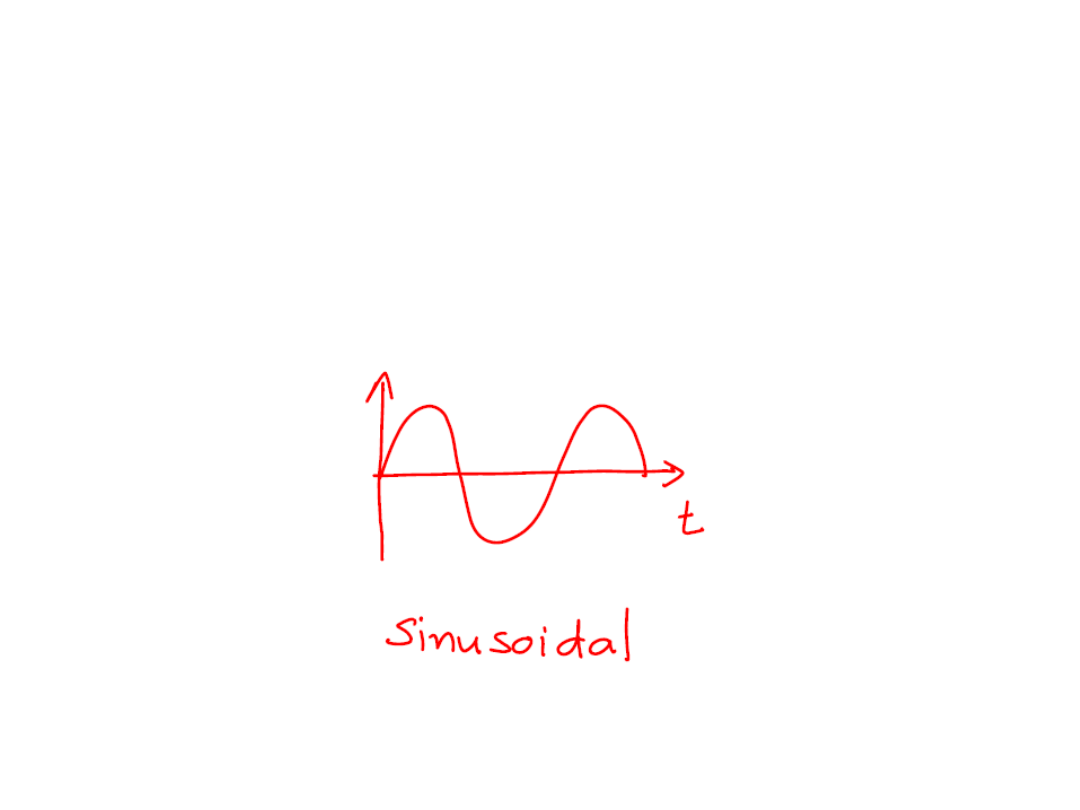
– Sinusoidal change: primary element

follows a measured, magnitude of which
changes in accordance with a sinusoidal
function of constant variable.

Dynamic characteristics

• Speed of response : rapidity with which an

instrument responds to changes.

• Fidelity : degree to which an instrument

indicates the changes in measured value

w/o dynamic error.

• Lag : retardation in the response of

instrument to change

• Dynamic error: difference b/w true value of

quan. Changing with time and the value

indicated by the instrument (no static error

is assumed)

Dynamic response

• Zero order instruments
• 1

st

order instruments

• 2

nd

order instruments

• Link:

http://www.jgsee.kmutt.ac.th/exell/Prac

Math/Instruments.html

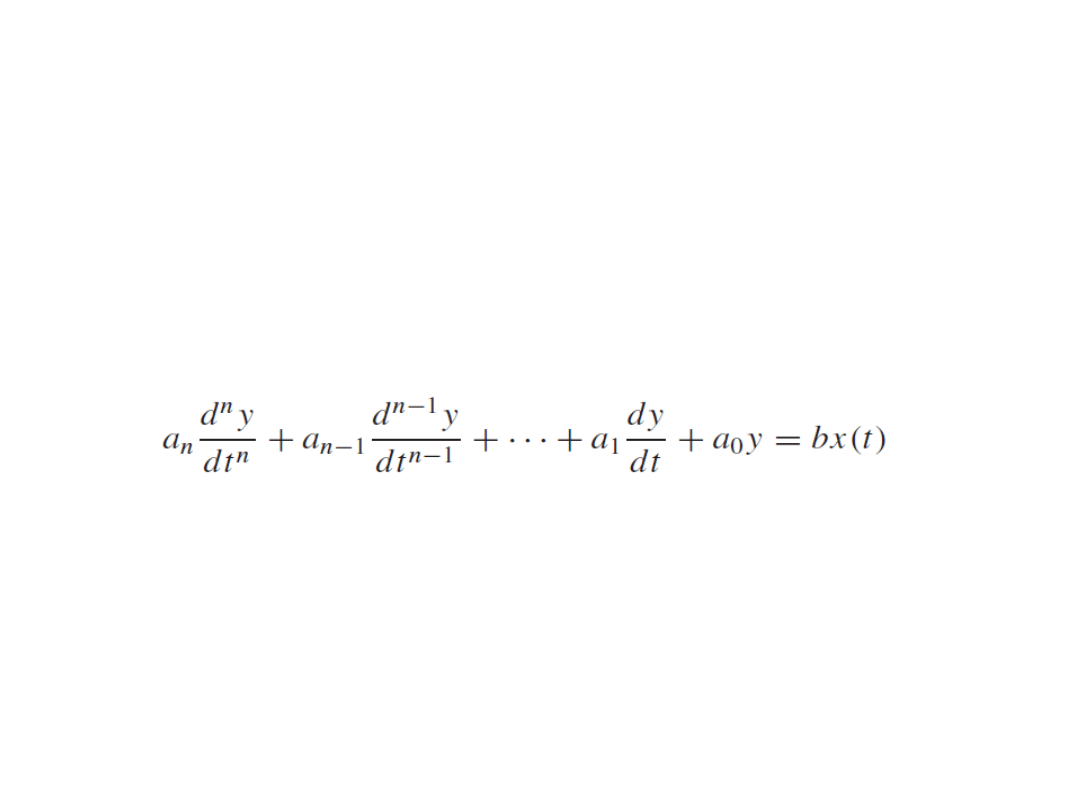
General Instrument

Response

The traditional way to investigate the dynamic
response of an instrument is to consider the
differential equation that describes the output. We
assume that the instrument response can be
modeled using a linear ordinary differential equation
with constant coefficients

where y is the instrument output, x is the input,
and n is the order of the instrument.

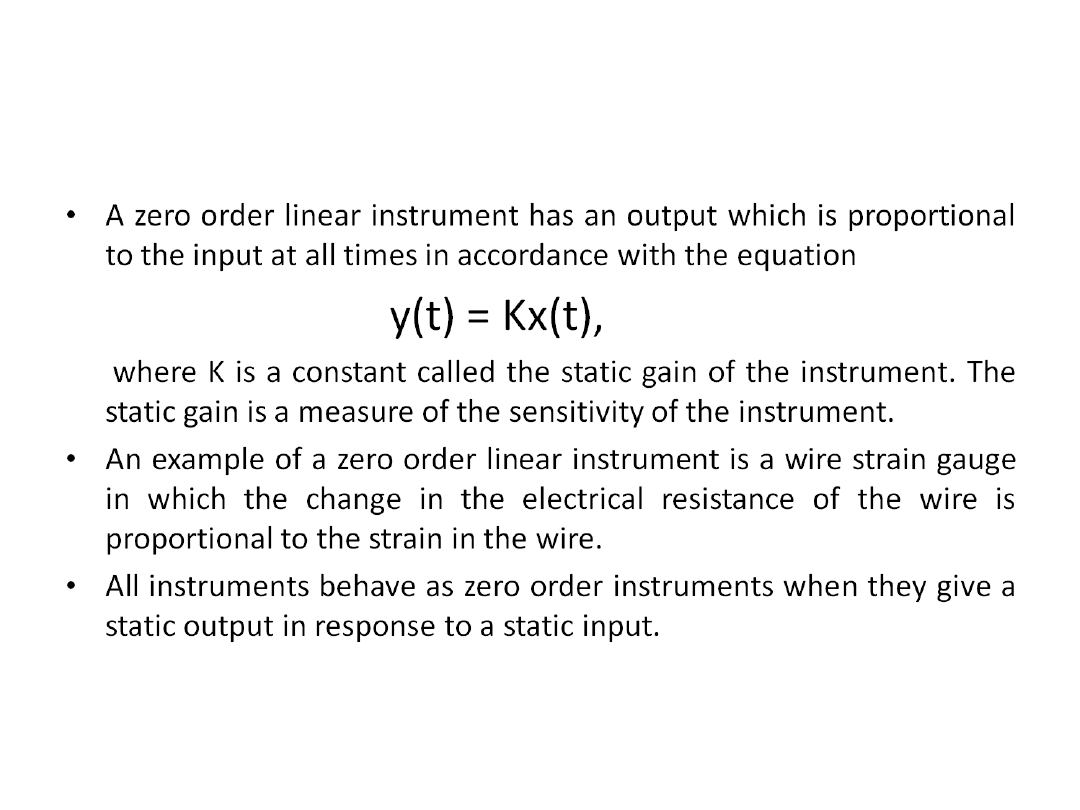
Zero Order Linear

Instrument

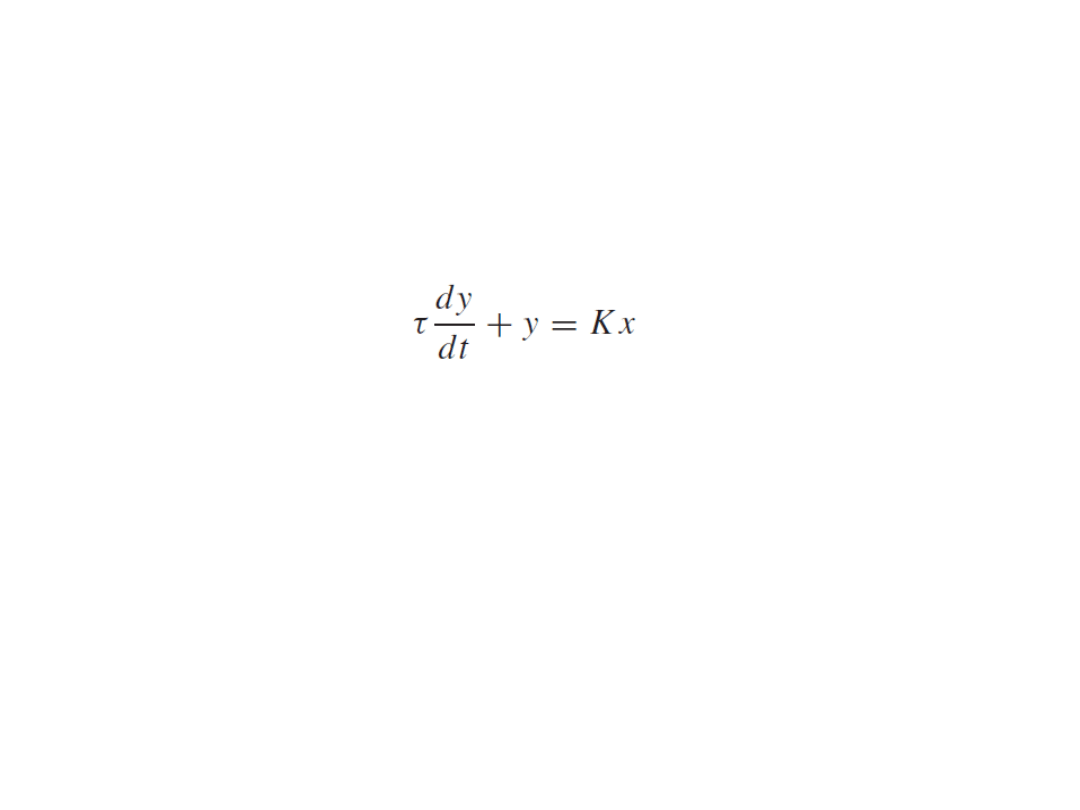
First Order Instruments

• A first order linear instrument has an output which is given

by a non-homogeneous first order linear differential equation

where τ (= a1/a0) is the time constant and K (= b/a0) is the

static gain.

• In these instruments there is a time delay in their response to

changes of input. The time constant tau is a measure of the

time delay.

• Thermometers for measuring temperature are first-order

instruments. The time constant of a measurement of

temperature is determined by the thermal capacity of the

thermometer and the thermal contact between the

thermometer and the body whose temperature is being

measured.

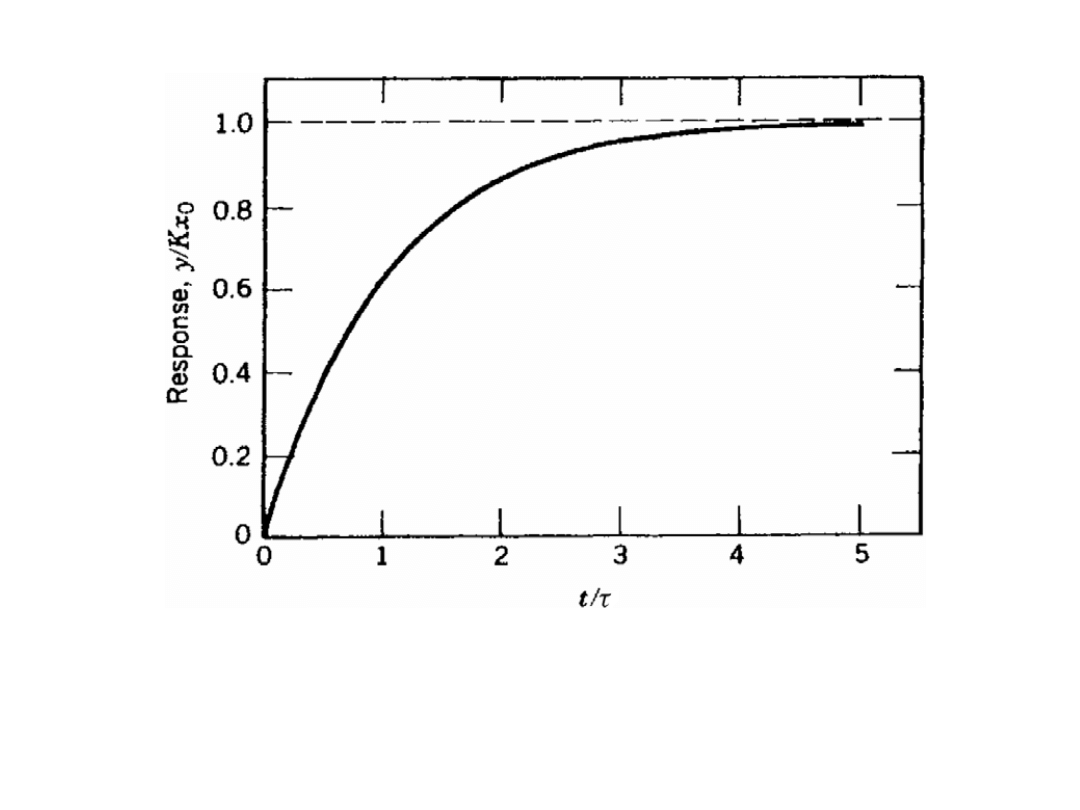
Response of a first-order instrument to a step change input

versus nondimensional time.

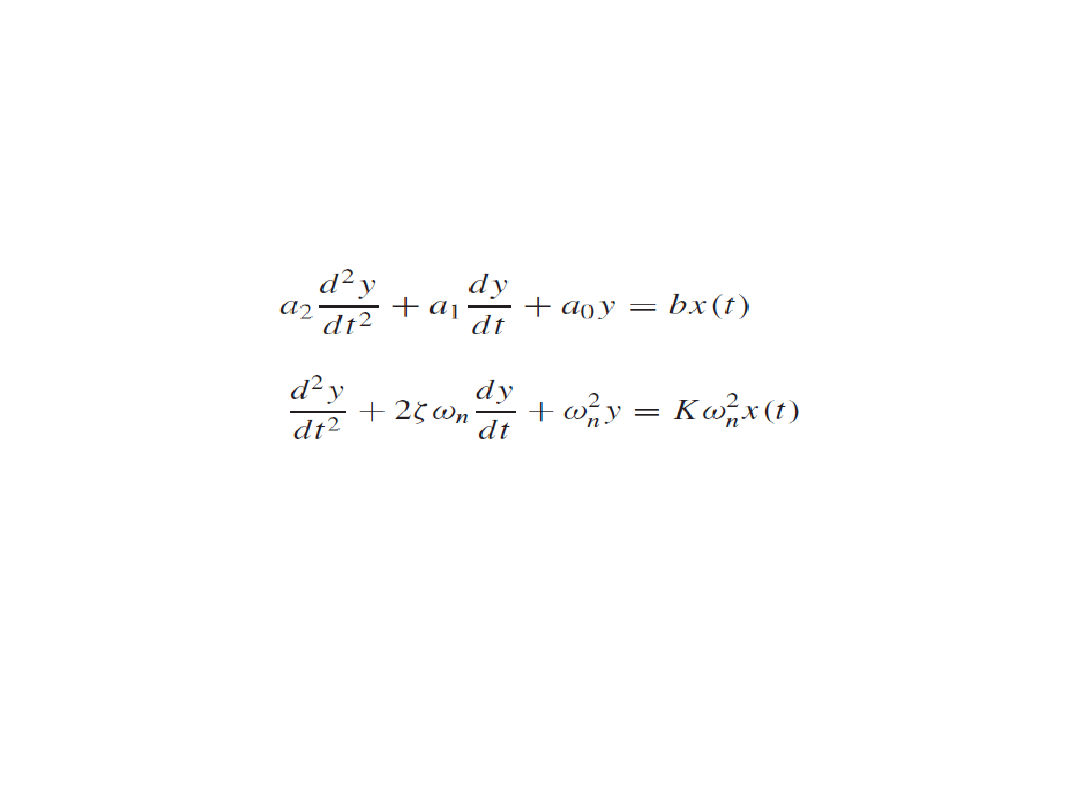
Second Order Instruments

• A second order linear instrument has an output which is given

by a non-homogeneous second order linear differential equation

K(= b/a0) is again the static gain, ζ(= a

1

/2√a

0

a

2

) is the damping

factor, and ωn(= √ a

0

/a

2

) is the natural frequency. Under a static

input a second order linear instrument tends to oscillate about its
position of equilibrium. The natural frequency of the instrument is
the frequency of these oscillations.

• Friction in the instrument opposes these oscillations with a

strength proportional to the rate of change of the output. The
damping factor is a measure of this opposition to the oscillations

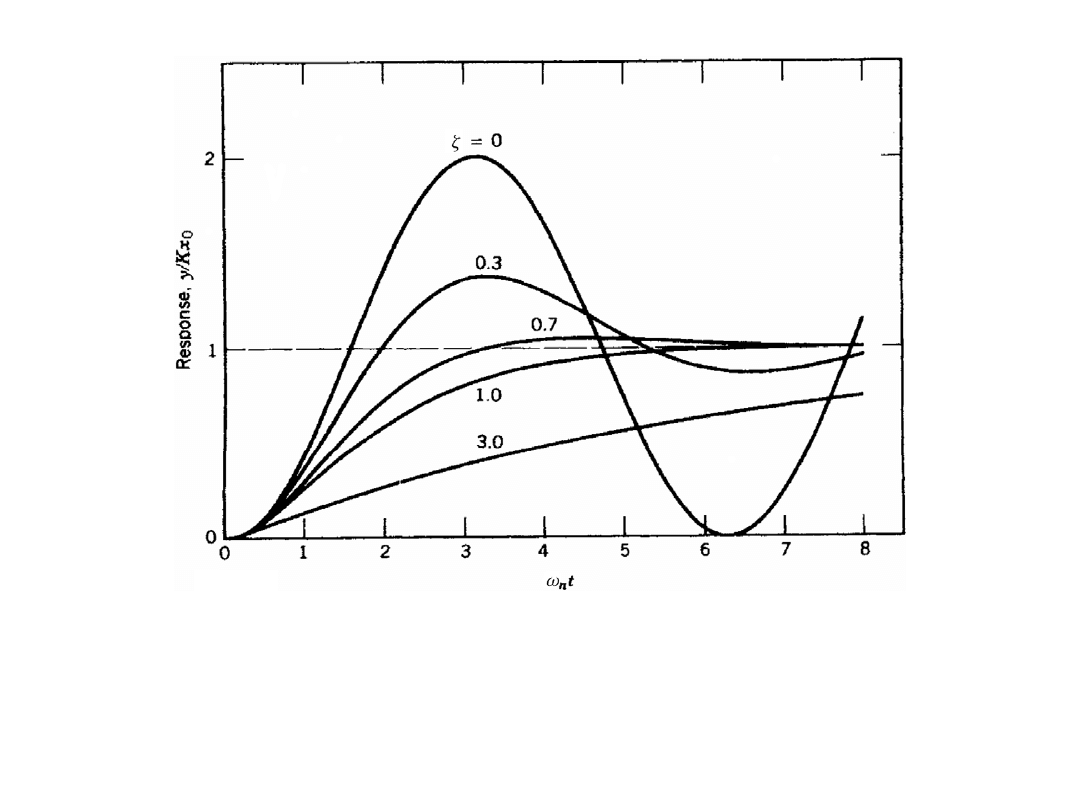
Response of a second-order instrument to a step

change input for various damping factors.

Statistical analysis

• Arithmetic mean
• Deviation from the mean
• Average deviation
• Standard deviation
• Limiting errors