Managerial

Managerial

Economics

Economics

Jarek Neneman

[e-mail]

601305093

Auctions and competitive bidding
(ch. 17, Samuelson & Marks)

Auctions

Everything is worth what
it’s purchaser will pay for it

Auctions

A exercise to start with – bidding for a

jar of money.

 Take the look at the jar
 Write down:

- estimated value of the jar (i. e. money)

- sealed bid for the jar

 The person with the highest bid will win and

pay and be given an equivalent of the money

in the jar

Auctions

Auctions and competitive bidding

 Auctions were used by the ancient

Babylonians as a way of distributing wives.

 The two most frequently used methods of

selecting a best alternative:

- sealed-bid auction

- English auction,

let’s play it!

- Third kind of auction method is Dutch

auction

Auctions

The advantages of auctions

 Auctions are between:

- posted prices, and

- negotiations

 Auctions ensure that competition among

buyers sets the highest price.

 Auctions are less time-consuming than

negotiations.

 Let’s see the example

Auctions

The advantages of auctions – a stock

repurchase

 A stock repurchase.
 A company is considering buying-back some

of its stocks.

 The current price is: $67 per share
 Management believes the value of the stock

is: $80 per share

 Management’s offer would be: $70, $72 or

$74

 The response of shareholders is not known

Auctions

The advantages of auctions – a stock

repurchase

Shareholders response (Q)

Price

strong medium weak

$

(π=1/3) (π=1/3) (π=1/3)

70

13

9

6

72

14

12

8

74

18

15

12

 Company’s profit is:

($80-P)*Q

Auctions

The advantages of auctions – a stock

repurchase

 What buy back price should the firm set?

Shareholders response (Q)

Price

strong

medium

weak

$

(π=1/3)

(

π=1/3)

(π=1/3)

70

13

9

6

72

14

12

8

74

18

15

12

 E(Π

$70

) = 1/3*10*13 + 1/3*10*9 + 1/3*10*6 =

93.3

 E(Π

$72

) = 1/3*8*14 + 1/3*8*12 + 1/3*8*8 =

90.67

 E(Π

$74

) = 1/3*6*13 + 1/3*6*9 + 1/3*6*6 = 90

Auctions

The advantages of auctions – a stock

repurchase

 Instead of setting the buy-back price, the firm

can organize an auction.

 In this system, each shareholder tenders any

number of shares at a price he names. eg. „I

will sell 10 at $70 or 20 at $72”.

 After collecting of the tenders, the firm buys

the shares at a single common price.

 What is the advantage of this „auction?
 Before deciding on price, the firm knows the

shareholders’ response.

Auctions

The advantages of auctions – a stock

repurchase

 The firm’s most profitable offer (price) is

contingent on demand.

Shareholders response (Q)

Price

strong

medium

weak

$

(π=1/3)

(

π=1/3)

(π=1/3)

70

13

9

6

72

14

12

8

74

18

15

12

 Strong response: price: $70, profit: $130
 Medium response: price: $72, profit: $96
 Weak response: price: $74, profit: $72

Auctions

The advantages of auctions – a stock

repurchase

 Strong demand: price: $70, profit: $130
 Medium demand: price: $72, profit: $96
 Weak demand: price: $74, profit: $72
 E(Π)= 1/3*130 + 1/3*96 + 1/3*72 = $99.3
 Auction compared to posted prices increased

expected profit by $6.

 $6 is the expected value of perfect

information about response

Auctions

The advantages of auctions – bidding vs.

bargaining

Searching for best price revisited
 Seller believes that the potential buyers’

offers to purchase the division are uniformly

distributed in the range form $40-64m

 The average is $52m
 Seller’s reservation price is: $40m
 What will be the average price in negotiation

with single buyer?

 $46m. on average is very likely (profit from

transaction is split fifty-fifty).

Auctions

The advantages of auctions – bidding vs.

bargaining

Searching for best price revisited
 The firm may do better by selling with use of

sealed-bid auction.

 With 6 buyers, the price offered by the

highest bidder will be $60m. on average.

 The sources of higher price are twofold:

- the higher the number of bidders, the

higher the probability that the one will hold a

high value

- the higher the number of bidders, the

stronger the incentive for them to place bid

near to its true value.

Auctions

Bidder strategies

 Optimal bid depends on:

- reservation price

- assessment of the extent of bidding

competition

- type of auction (the most important factor)

Auctions

Bidder strategies

 Time for another auction
 Vickery auction (second-price (second-

bid) auction)

 The highest bid wins the item.
 But the price is equal to the second-highest

bid

Auctions

Bidder strategies

Independent private value setting
 Each bidder assesses an individual value

(reservation price) for the item up for bid.

 Each bidder’s value is independent of the

other’s

 Values are private and bidders are aware of

the common probability distribution.

 The profit of the bidder is:

v

i

- P

Auctions

Bidder strategies – English and Dutch

auctions

English and Dutch auctions
 In oral English auctions bids continually

increase until the last and highest bidder

wins.

 Bidder’s strategy: bid up to the reservation

price if necessary – dominant strategy

 P is close to the second-highest reservation

price: v

2nd

.

 The seller can obtain exactly the same price

using Vickery auction (second-price (-

bid) auction)

P

2nd

= P

E

= v

2nd

Auctions

Bidder strategies – English and Dutch

auctions

 In Dutch auction the auctioneer starts the

sale by calling out a high price and the

lowers the price until a bid is made.

 Optimal strategy in Dutch auction is

significantly different than in English auction

 In Dutch auction there is risk, that another

buyer bid and win the item

 In English auction there is no risk
 Dutch auctions are similar to sealed-bid

auctions – the price achieved by seller is the

same

Auctions

Bidder strategies – sealed-bid auctions

Sealed-bid auctions are used to sell unique

items

 Each bidder faces trade-off between

probability and profitability of winning

 What bid will maximize the bidder’s expected

profit?

Auctions

Bidder strategies – sealed-bid auctions

Strategy against a bid distribution
 To win the auction the firm must beat the

best competiting bid.

 The distribution of competing bid is a key to

success.

 An example

Auctions

Bidder strategies – sealed-bid auctions –

an example

 v

1

= $342 thousand

 E(Π) = (342 – b)*[probability (b wins)]
 There are two more bidders
 Assessment winning chances and expected

profit are given in the table

Auctions

Bidder strategies – sealed-bid auctions –

an example

Bid

Winning Probability

Expected

$

profit

of winning

profit

300

42

0.00

0.00

310

32

0.06

1.92

320

22

0.25

5.50

326

16

0.42

6.67

328

14

0.49

6.86

332

10

0.64

6.40

336

6

0.81

4.86

340

2

1.00

2.00

 Optimal bid is:

$328

Auctions

Bidder strategies – sealed-bid auctions –

an example

 Firm’s winning chance depends on probability

that the best competing bid (BCB) is

smaller than the firm’s own bid.

 H curve is graphical presentation of BCB

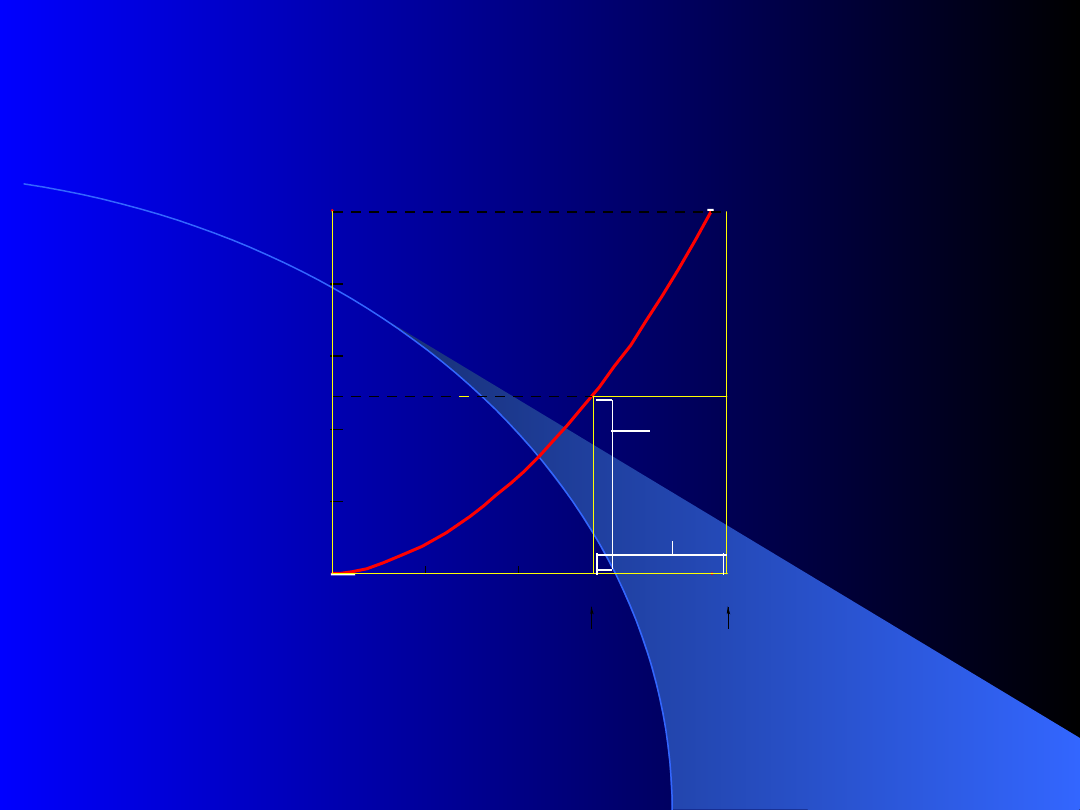
Auctions

Bidder strategies – sealed-bid auctions –

an example

1.0

.8

.6

.49

.4

.2

0

Probability

H curve

Probability

b = 328 wins

Firm 1's profit

v - b

310

300

320 328

342

Firm 1's

optimal bid

Firm 1’s
Reservation
price

At bid = $328, the area of the rectangle (342-b)H(b) is the

biggest.

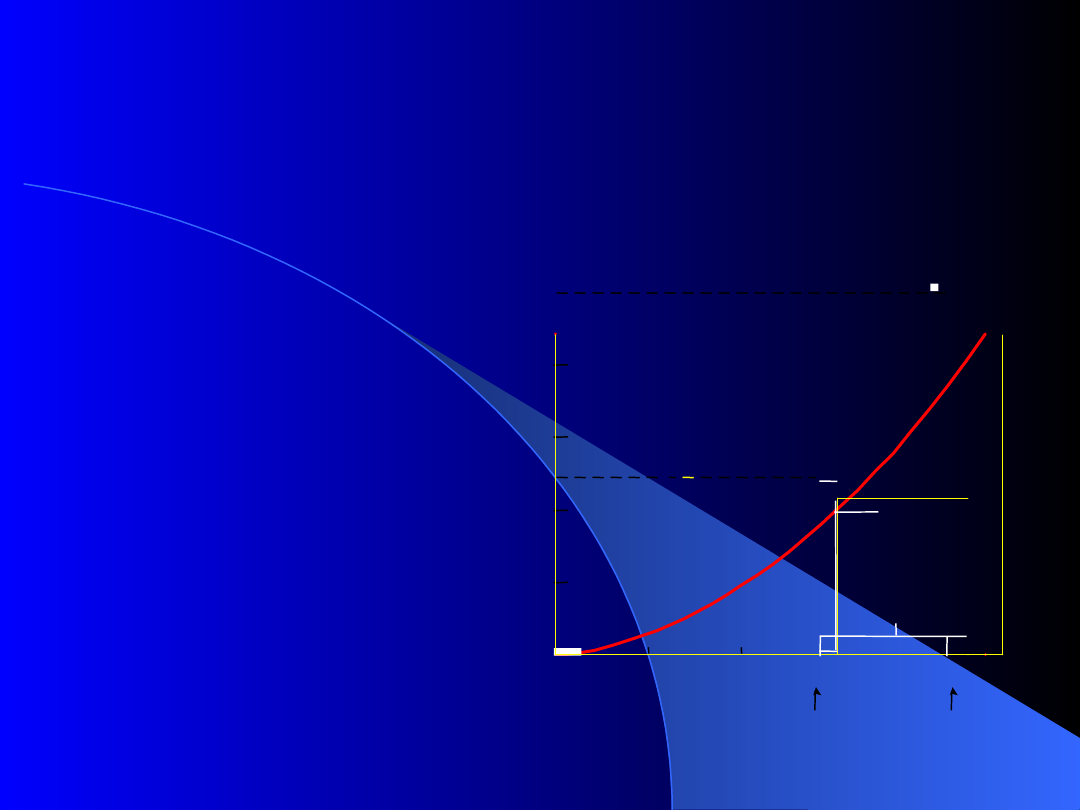
Auctions

Bidder strategies – sealed-bid auctions –

an example

1.0

.8

.6

.49

.4

.2

0

Probability

H curve

Probability

b = 328 wins

Firm 1's profit

v - b

310

300

320 328

342

Firm 1's

optimal bid

Firm 1’s
Reservation
price

H curve is graphical

presentation of

BCB

It precisely measures

the firm’s winning

chances for its

various bids

Median of the BCB is

slightly higher

than $328.

Auctions

Bidder strategies – sealed-bid auctions –

an example

Arriving at BCB
 Let G(b) denote the cumulative distribution

function for the bid of a single competitor.

 If firm’s bid is b, then the chances that this

bid is higher than the bid of the single

competitor is G(b)

 What if there are two competitors?
 If bids are independent of one another, then

probability, that firm’s bid is better than both

competiting bids is:

[G(b)]

2

Auctions

Bidder strategies – sealed-bid auctions –

an example

 Generally:

H(b) = [G(b)]

n-1

for n-1 competitors firm is facing

 An example continued
 Firm’s assessment is that each competitor’s

bid will be in the range of $300 and $340,

with all values in between equally likely. This

implies:

G(B) = (b-300)/40

 With two competitors: H(b) = [(b-300)/40]

2

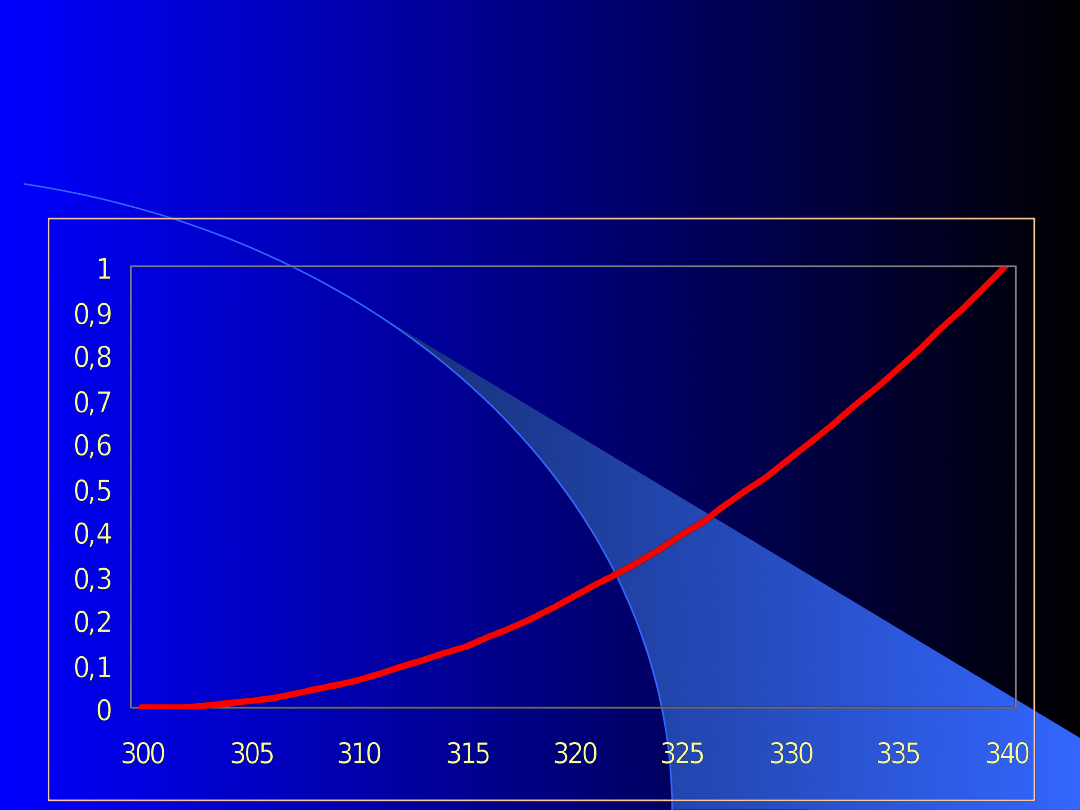
Auctions

Bidder strategies – sealed-bid auctions –

an example

N-1=

2

Auctions

Bidder strategies – sealed-bid auctions –

an example

N-1=

2

, n-1=

3

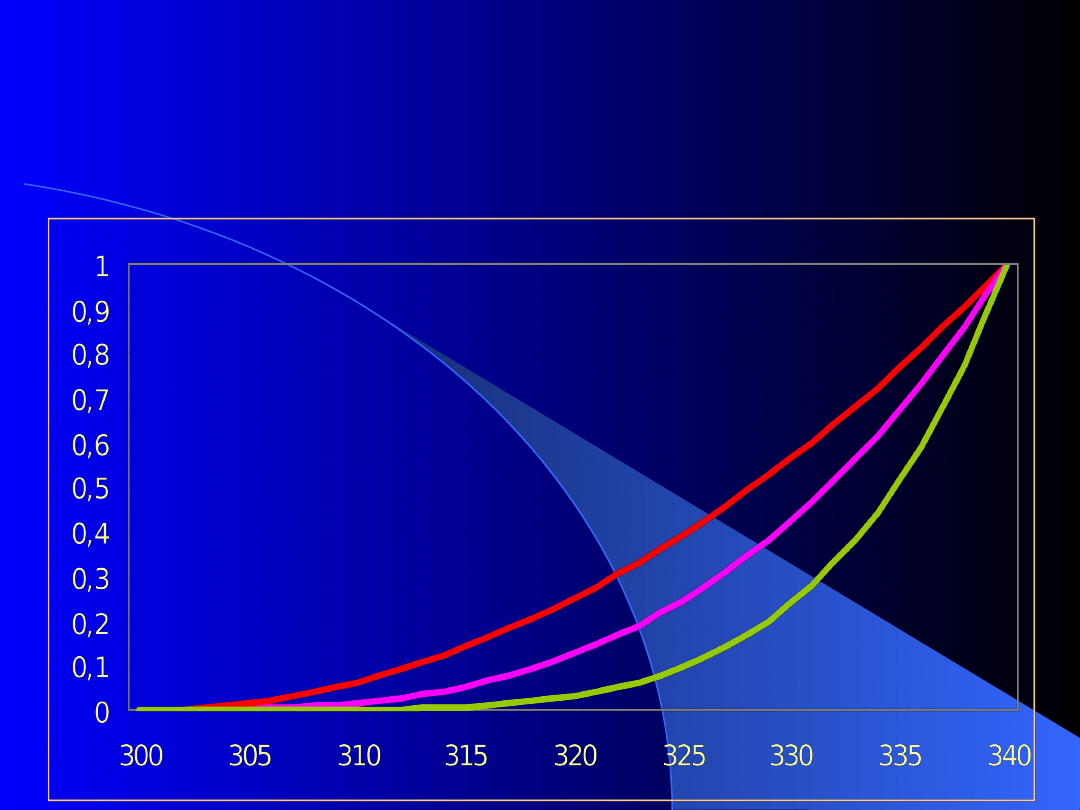
Auctions

Bidder strategies – sealed-bid auctions –

an example

N-1=

2

, n-1=

3

, n-1=

5

Auctions

Bidder strategies – sealed-bid auctions –

an example

N-1=

2

, n-1=

3

, n-1=

5

, n-1=

10

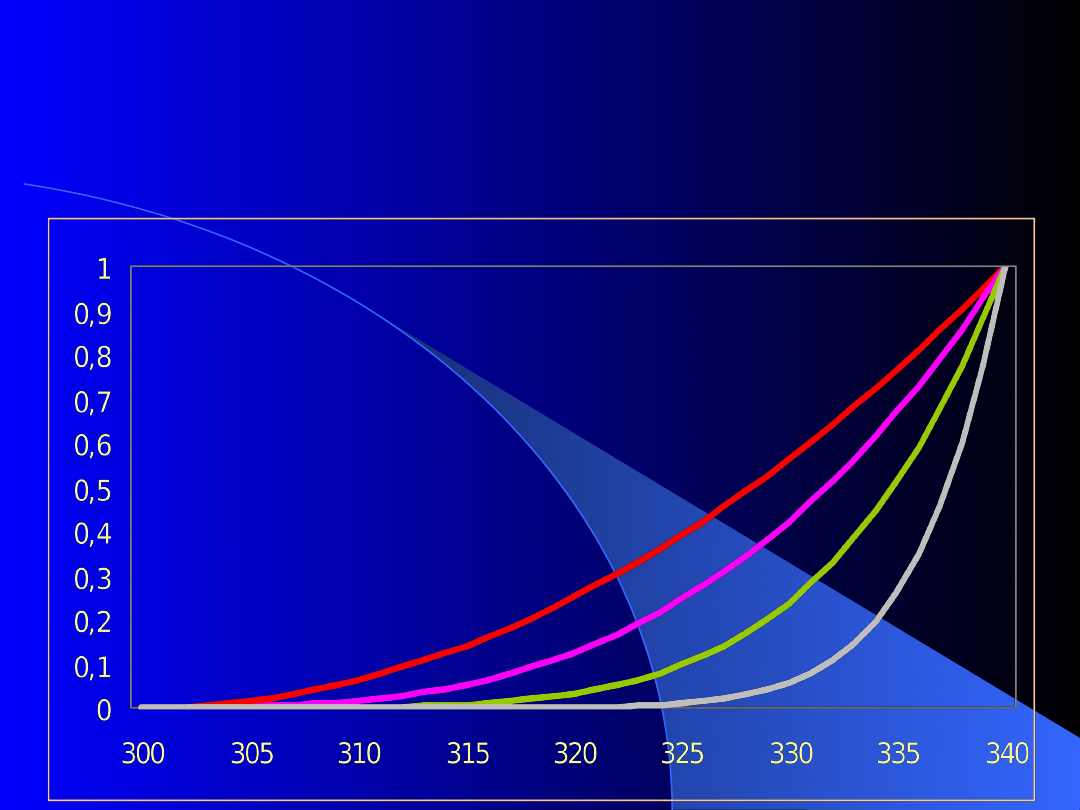
Auctions

Bidder strategies – sealed-bid auctions –

an example

 With increasing number of competitors, the

bid distribution of the typical firm, G(b), will

be shifted towards higher bids.

 This happens because:

- there are more competitors (that could be

seen on the previous graphs)

- each of them raise its bid

Auctions

Bidder strategies – sealed-bid auctions –

interdependence

Equilibrium bidding strategies
 Usually it is difficult for a firm to assess G(b)

or H(b)

 All competitors are setting competitive bid,

taking into account others’ strategies, i.e.

there is interdependence among bidding

strategies

Auctions

Bidder strategies – sealed-bid auctions –

interdependence

 Bidding for the good with common value.
 All bidders have the same reservation price.
 The unique equilibrium has each bidder

submitting a sealed bid exactly equal to this

common value (reservation price).

 Analogy for Bertrand competition

Auctions

Bidder strategies – sealed-bid auctions –

interdependence

Bidding for the good with different private

values

 n bidders,
 v

i

private value

 b

i

sealed bid

 Buyers’ values are drawn independently from

a common distribution

 Buyers values are independent
 Each buyer’s value is uniformly distributed

between $300 and $360

Auctions

Bidder strategies – sealed-bid auctions –

interdependence

 If bidder knows his v

i

but do not know the

reservation prices of the other competitors,

then

 his equilibrium bidding strategy is:

b

i

= 0.5 * 300 + 0.5v

i

 For v

i

= 300, b

i

=300

 For v

i

= 360, b

i

= 330

 Expected profit of the first firm is:

E(Π

1

) = (v

1

– b

1

)[probability(b

1

wins)]

Auctions

Bidder strategies – sealed-bid auctions –

interdependence

 If firm 2 uses equilibrium bidding strategy,

then its competiting bids are distributed

uniformly between $300 and $330,

 Thus b

1

wins with probability:

(b

1

– 300)/30, plugging this into expected

profit function gives:

E(Π

1

) = (v

1

– b

1

)*[(b

1

– 300)/30]

 Setting dE(Π

1

)/db

1

= (v

1

– 2b

1

+300)/30 to

zero, implies:

b

1

= 0.5 * 300 + 0.5v

1

Auctions

Bidder strategies – sealed-bid auctions –

interdependence

 For n competiting firms, the common

equilibrium strategy is:

b

i

= (1/n)*L + [(n-1)/n]* v

i

 In sealed-bid auction, the equilibrium

strategy is to submit a bid b

i

, equal to the

expected value of the highest of the n-1

other buyers values, conditional on these

values being lower than v

i

.

b

i

= E(v’|v’≤v

i

)

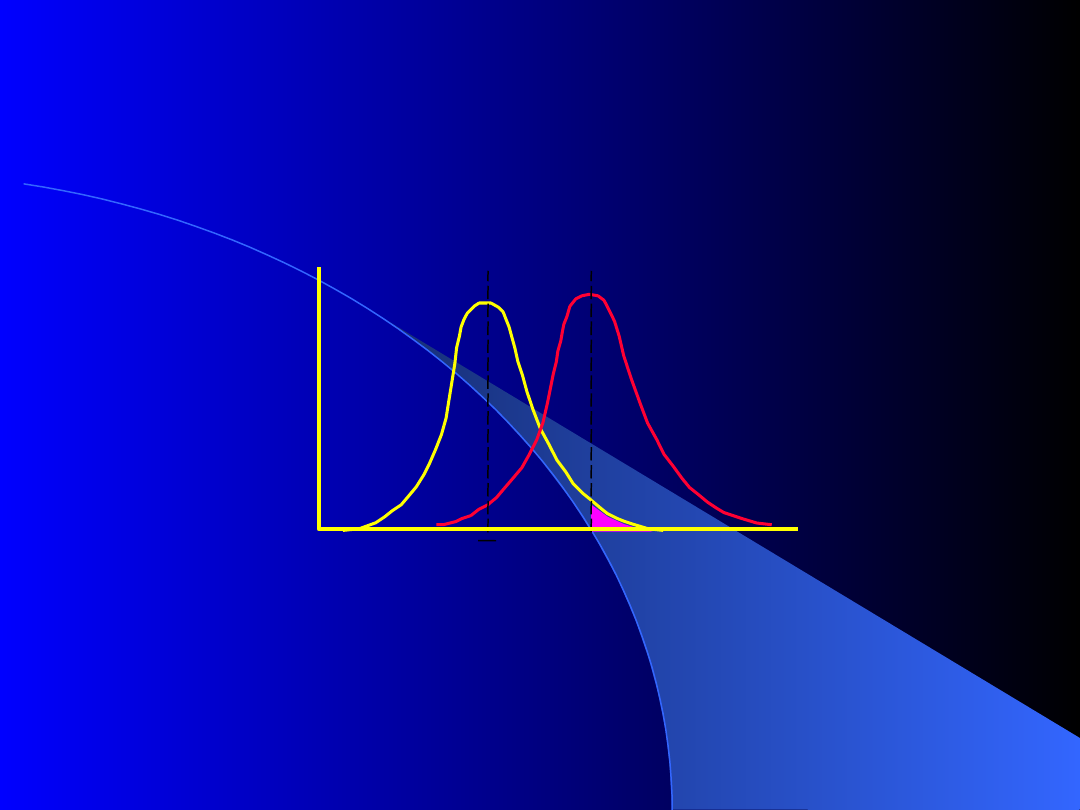
Auctions

Bidder strategies – common values and the

Winner’s curse

 Auctions for common but unknown value

Bids (B)

Estimates (E)

B

V

Bid distribution is centered to the left, as

bidders are seeking profits and bid below

the estimate of value

Auctions

Bidder strategies – common values and the

Winner’s curse

 Winner’s curse – after the auction (ex post)

winner finds that the good is worth less than

the price paid for it.

 Overestimation of the value is the source of

winner’s curse

 Winning conveys information about the

bidder’s estimate relative to others.

 Winner’s curse depends on:

 degree of uncertainty
 number of bidders

Auctions

Bidder strategies – common values and the

Winner’s curse

 There is a significant difference between

sealed-bid auction and English auction of an

common, but unknown value item.

 Observing the number of active bidders and

when they drop out conveys information

about competitor’s estimates of the item’s

value.

Auctions

Optimal auctions – expected auction

revenue

Optimal auctions from the seller’s perspective
 Expected auction revenue
 In private value model and risk-neutral buyer,

English, sealed-bid, Dutch and Vickrey

auctions generate identical expected

revenues – revenue equivalence theorem

Auctions

Optimal auctions – expected auction

revenue

Optimal auctions from the seller’s perspective
 Seller’s expected revenue in the English and

Vickrey auctions:

E(P

E

) = E(v

2nd

)

 Seller’s expected revenue in the Dutch and

sealed-bid auctions:

b

i

= E(v’|v’ ≤ v

i

)

Auctions

Optimal auctions – expected auction

revenue

A uniform example
 Assume n buyers with reservation prices

independently and uniformly distributed

between L and U.

E(v

max

) = [1/(n+1)]L + [n/(n+1)]U

E(v

2nd

) = [2/(n+1)]L + [(n-1)/(n+1)]U

L

U

E (v

m ax

)

E (v

2nd

)

1

n+ 1

2

n+ 1

n - 1

n + 1

n

n + 1

Auctions

Optimal auctions – expected auction

revenue

V

max

is n/(n+1) toward U, and

He applies the factor in shading his bid below

his value: (n-1)/n

b

i

= (1/n)*L + [(n-1)/n]* vi

Multiplying: n/(n+1) by (n-1)/n gives:

(n-1)/(n+1) toward U – exactly as in English

auction

L

U

E (v

m ax

)

E (v

2nd

)

1

n+ 1

2

n+ 1

n - 1

n + 1

n

n + 1

Auctions

Optimal auctions – expected auction

revenue

Common-value setting
 English auction produces greater revenue, on

average, than the sealed bid auction

 Why?
 Intuitively:
 In common-value settings, buyers must

discount their bids to avoid the winner’s

curse.

 The greater the uncertainty (and the number

of bidders) the greater the bid discount.

Auctions

Optimal auctions – expected auction

revenue

Common-value setting
 English auction conveys more information

about others bidder’s estimates of value.

 Thus English auction informational advantage

translates into revenue advantage for the

seller.

 In auction experiments, however, bidder fall

pray to the winner’s curse and sealed-bid

auction holds a slight revenue advantage to

English auction, especially when the item’s

value is highly uncertain and when

competition is high.

Auctions

Optimal auctions – expected auction

revenue

Bidder risk aversion
 No effect on bidding in English auction

(bidding up to full value is still dominant

strategy

 In sealed-bid auctions this implies higher bids

– smaller but more certain profit.

 If a bidder’s value for an item is uncertain,

risk aversion implies reduction in certainty

equivalent value, which in turn lowers bids.

Auctions

Optimal auctions – expected auction

revenue

Value asymmetry
 Buyers values are draw from different

distributions

 Sealed-bid auction gives higher expected

revenue than English auction

Auctions

Optimal auctions – summary

Experiments confirm the following:
For common-value auctions:

For risk-neutral bidder, the rank order is:

English

> second highest sealed bid > Dutch =

highest price sealed bid

The seller does better with English auctions, whether

risk neutral or not.

Auctions

Optimal auctions – expected auction

revenue

Experiments confirm the following:
For private-value auctions:

For

risk neutral bidders

, English, Dutch, highest

sealed bid, or second highest bid auctions are all

alike in their outcome.
When bidders are

risk-averse,

the rank order from

highest is:

Dutch = highest price sealed bid

> English = second

highest sealed bid

Auctions

Additional readings

http://www.auctusdev.com/auctiontypes.html

http://www.youdontknowauctions.com/com_sect_

1.php