SLOPE STABILITY

D. A. Cameron, UniSA

Rock and Soil Mechanics 2008

Hummocky ground

Failure scarp in glacial till

“Toe
”

“Scar
p”

“Scar
p”

CONTENT

• What causes soil to move
• Why a soil mass can rotate out of position

– circular slips

• Stability assessments

– Factors of safety
– the influence of water
– Bishop’s simplified method
– choosing the appropriate soil strength

• Avoiding landslip

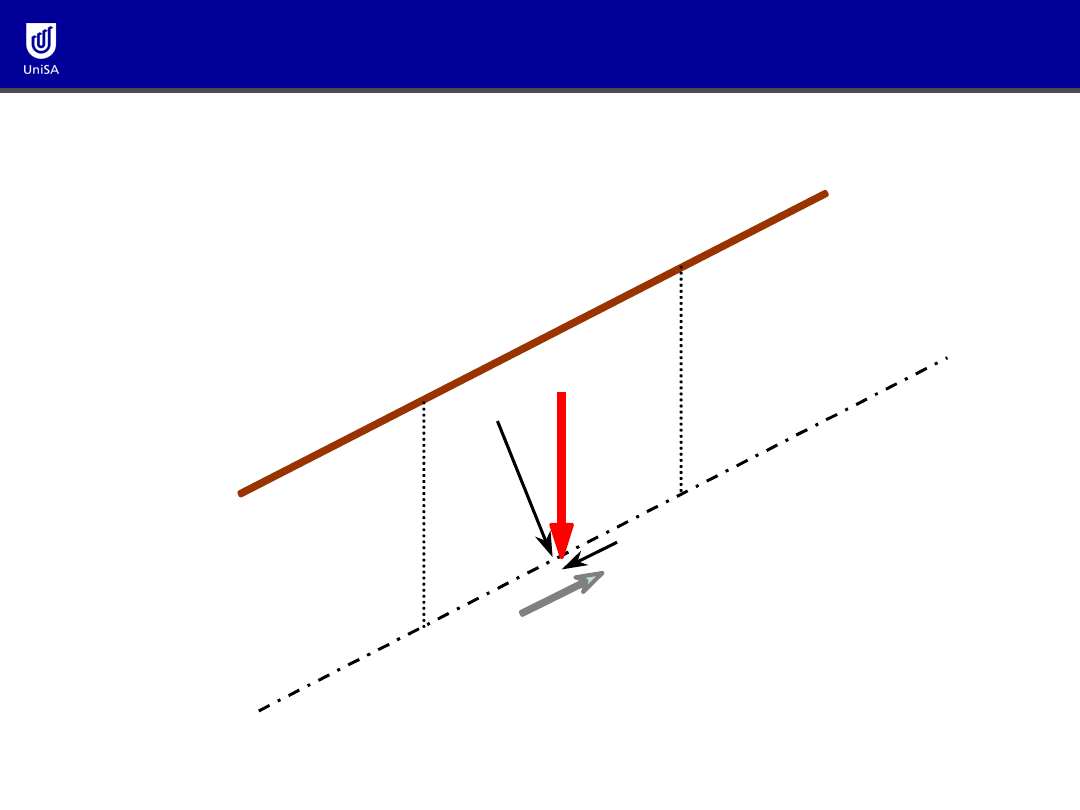
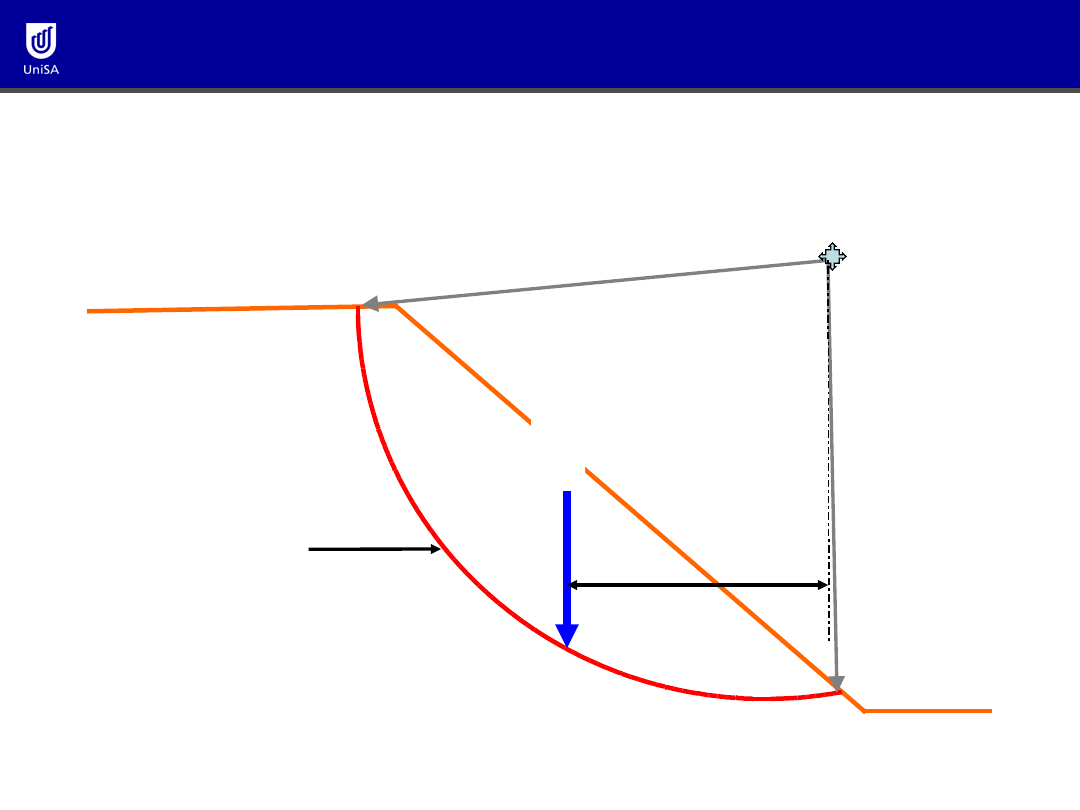
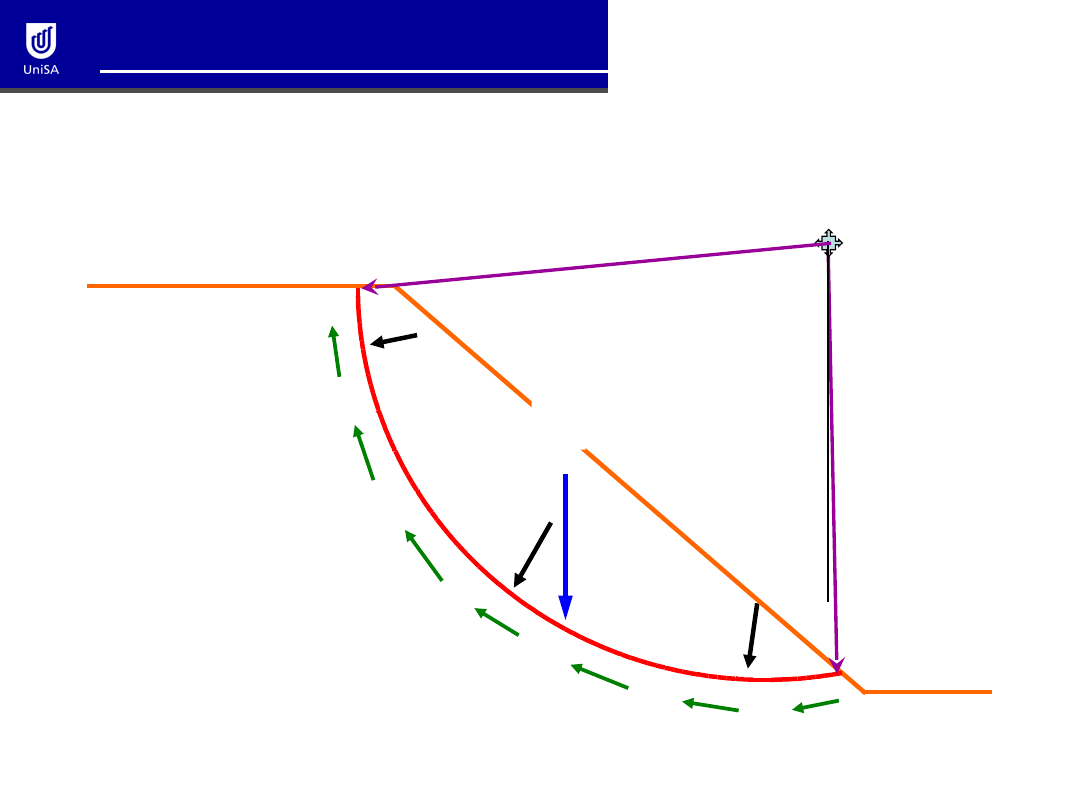
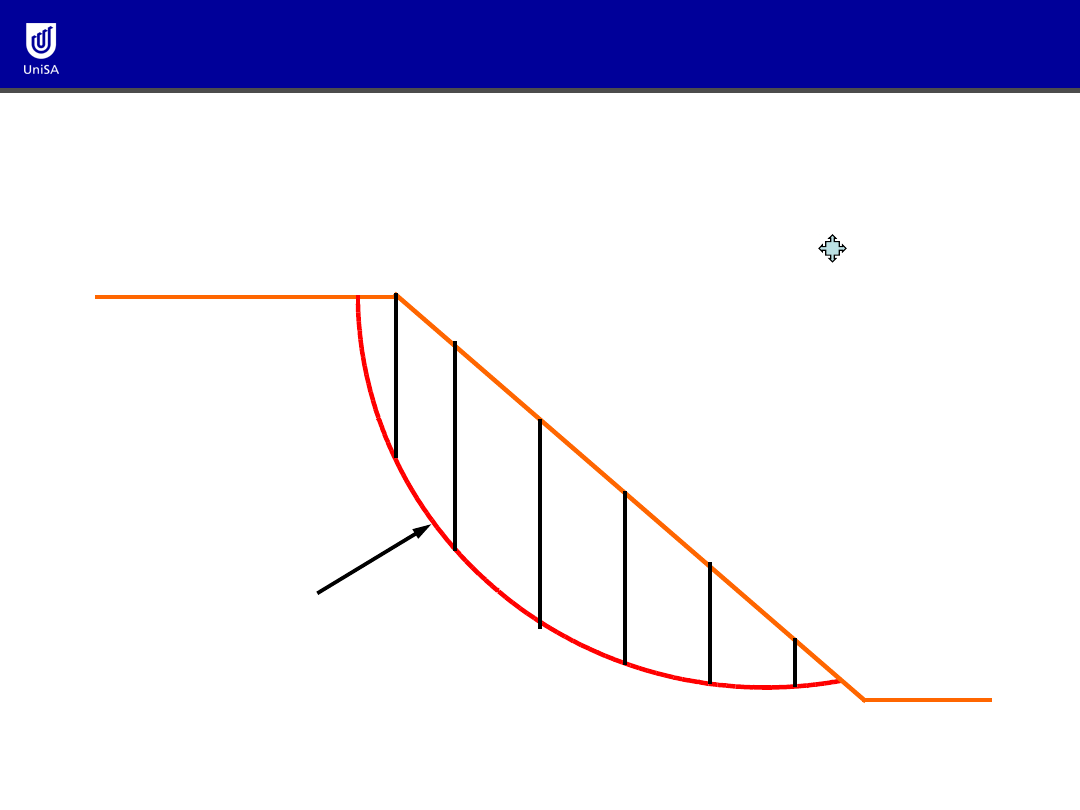
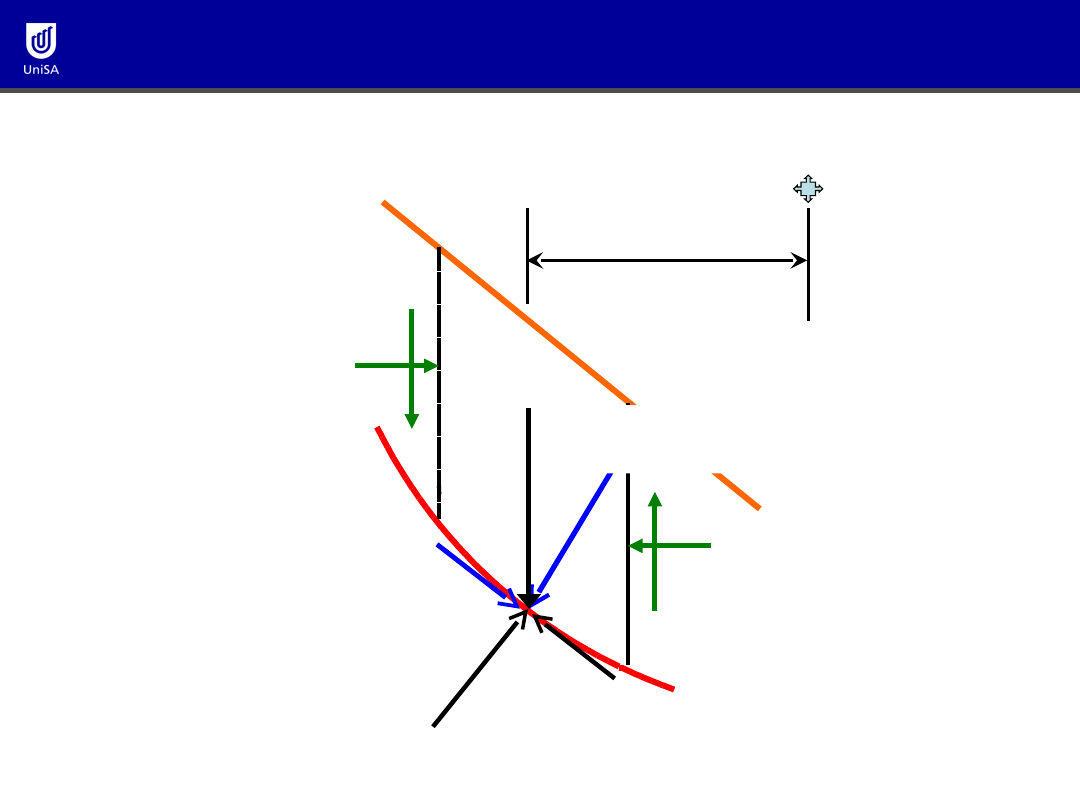
Infinite slopes?

res

ista

nce



Slid

ing

sur

fac

e

h

b

Vertical
slice

W

Force equilibrium – the slice

W

W

P

W

N

cl +

W

N

tan

'

W

N

= Wsin

l = length of
sliding
surface

STABILITY

Stability IF,

W

P

 (C + F)

 

where C = cl = resistance due to cohesion (kN)

and F = W

N

tan' = resistance due to friction  

Factor of Safety (FoS) = restoring force

disturbing force

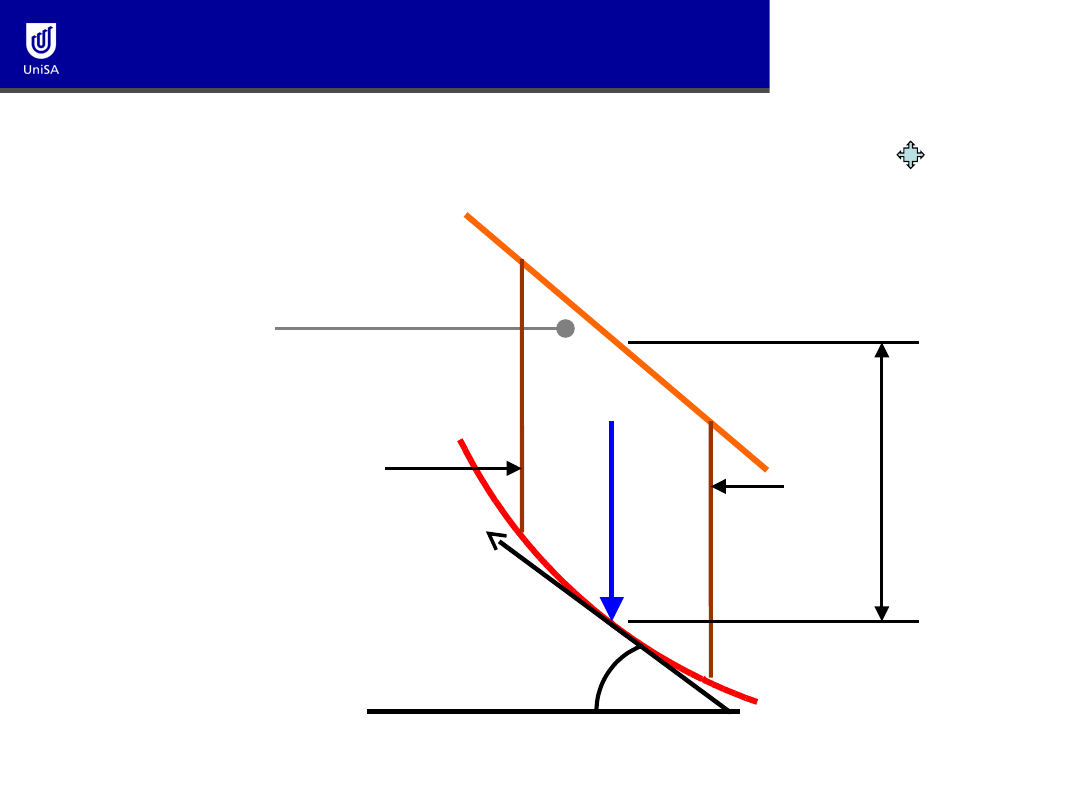
CASE 1: c = 0, so C = 0 (clean
sand)

tanβ

tan

FoS





The natural angle
of repose ?

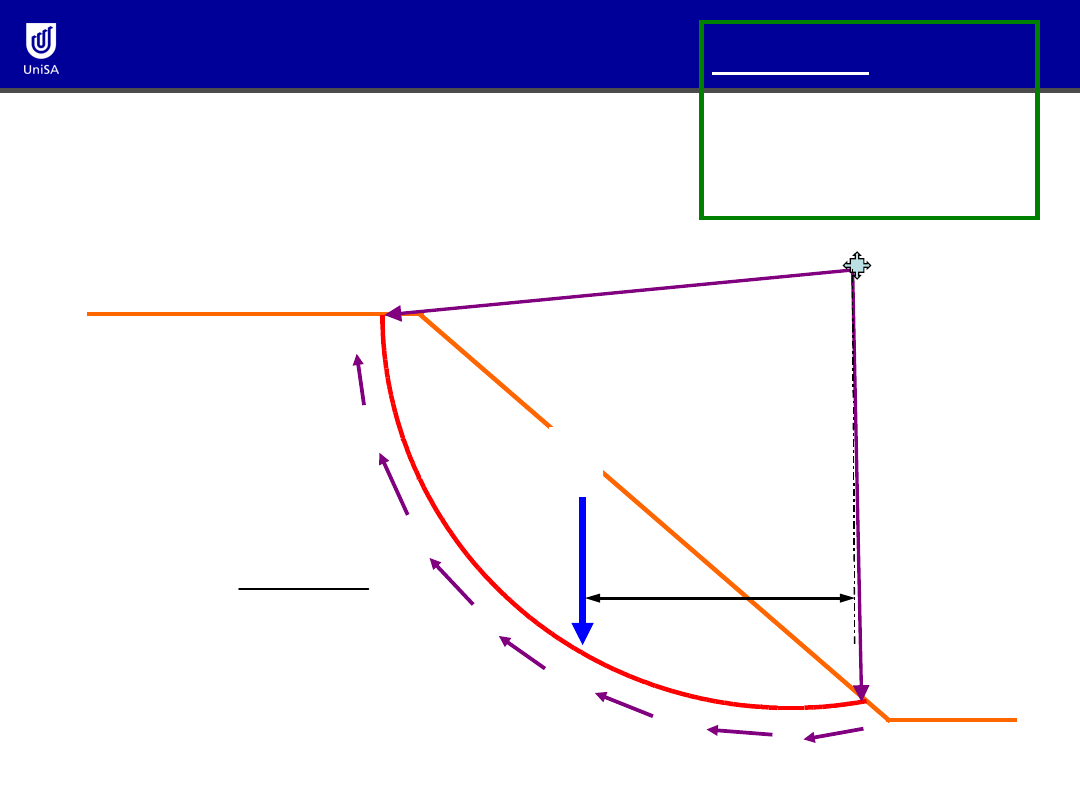
Case 2: c = 0,

Seepage down the slope

Phreatic surface at slope surface

Pore force, U, on sliding base due to pore water pressure

cosβ

bh

hl

U

w

w









Effective normal force reduced – less friction!

Case 2: solution













cosβ

sinβ

tan

cosβ

β

cos

FoS

2











– almost only half the FoS!

Stable Slope Angles (FoS = 1.3) c = 0
soils



Type of slope

 = 30 - 40

dry slope

 = 24 - 30.5

slope with seepage

 = 12.5 - 18

slope

crest of
slope

sliding
surface

centre of
circle

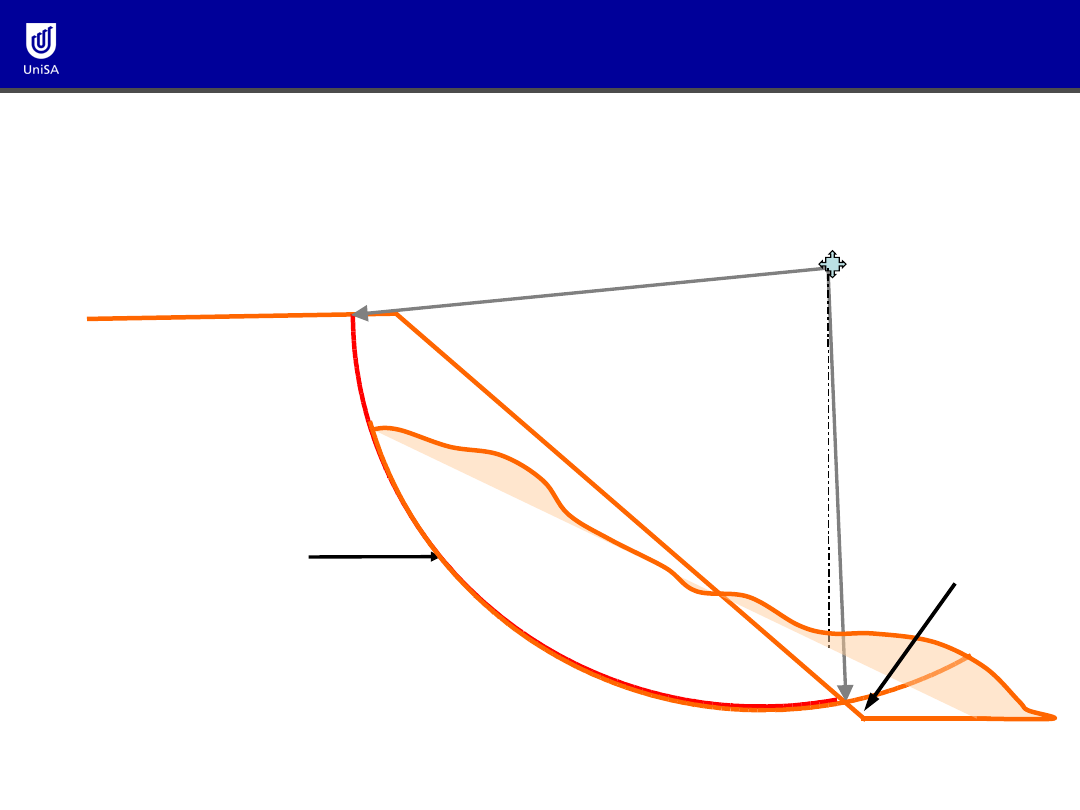
CIRCULAR SLIPS

More common in cohesive
soils

toe of
slope

A
potential
sliding
surface

toe

crest

centre of
circle

W

x

CIRCULAR SLIPS

CIRCULAR SLIPS

Stability? Limit
equilibrium

centre of
circle

Case 1:  =

0

c = c

u

W

x

Wx

R

L

c

FoS

arc

u



Taylor’s Charts

– slope stability for undrained shear strength, c

u

• Simple slopes
• Homogeneous
• Relative depth, D
• Stability number, N

s

WARNING: slopes are rarely
homogeneous

Taylor’s Charts:

F = FoS

















H

1

F

c

N

u

s

Bedrock?

D
H

H



Unit weight of soil =


Shear
strength =
c

u

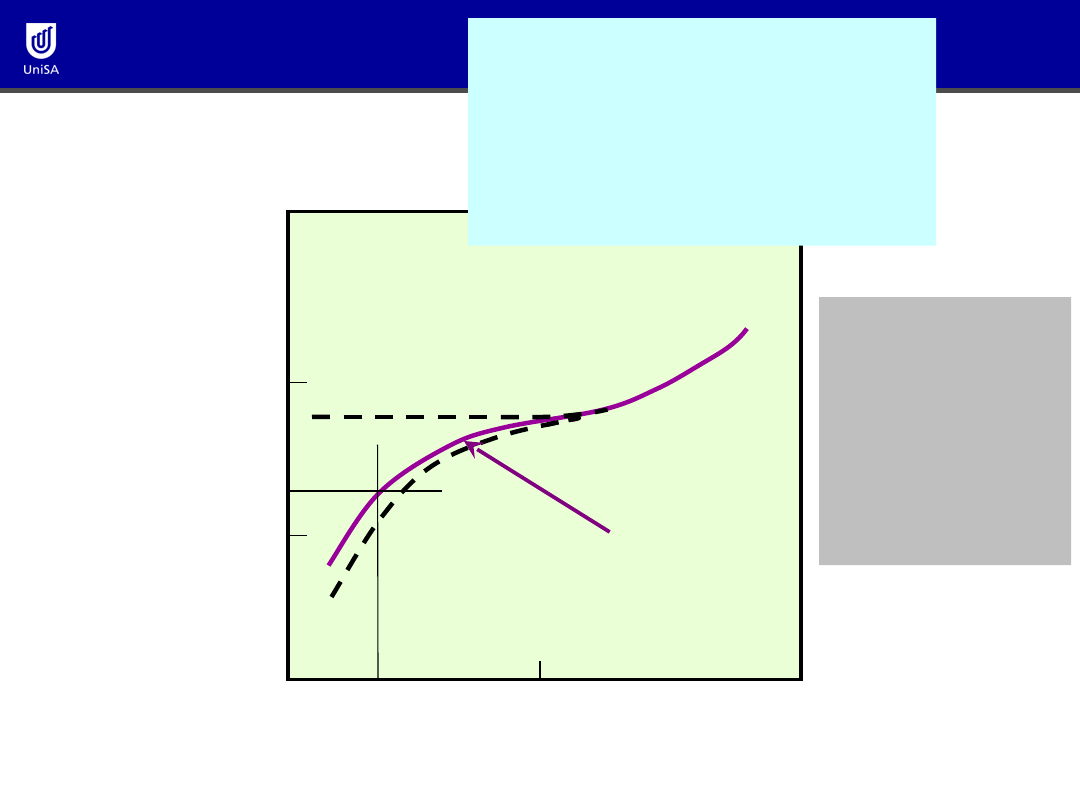
Example

0.1

0.2

0.0

S

ta

b

il

it

y

N

u

m

b

e

r,

N

s

Slope angle
(º)

45

90

H = 10 m, DH = 13 m

 = 20º,

F = 1.25

 = 18 kN/m

3

, c

u

= 30

kPa

20



N

s

= 30/

[1.25(18)10]

= 30/225

= 0.133

D = 13/10

= 1.3

D =


D =
1

D =
1.3

centre of
circle

W

x

CIRCULAR SLIPS

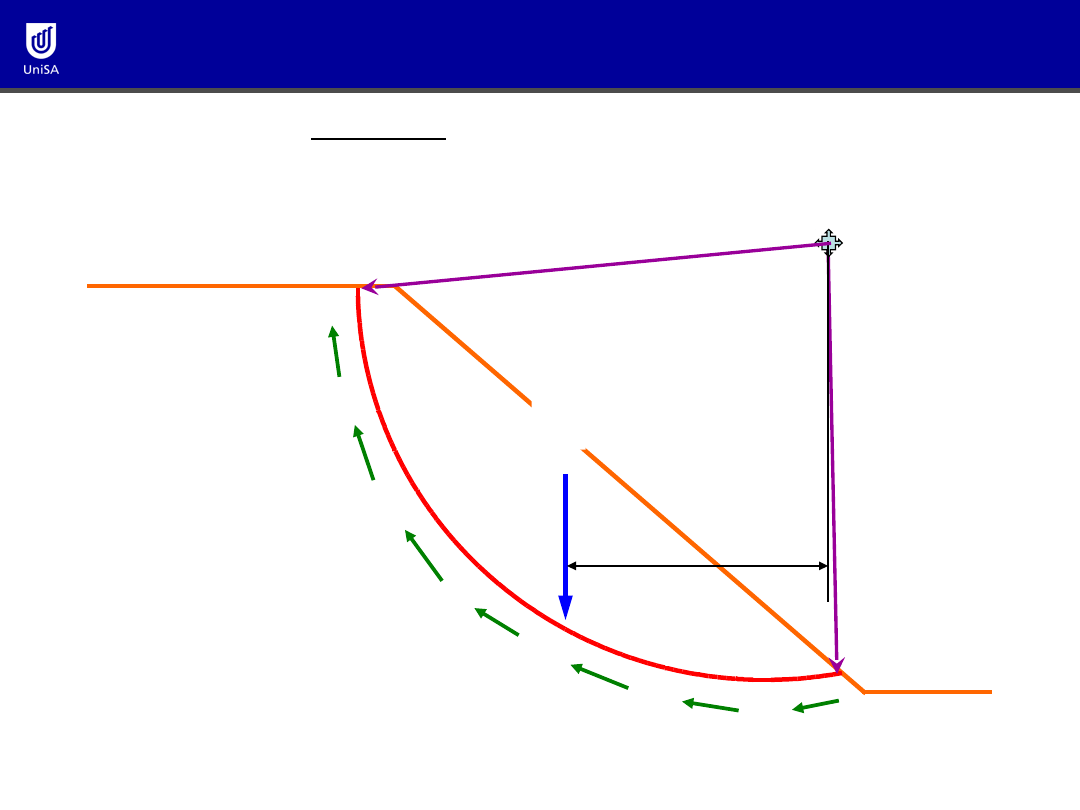
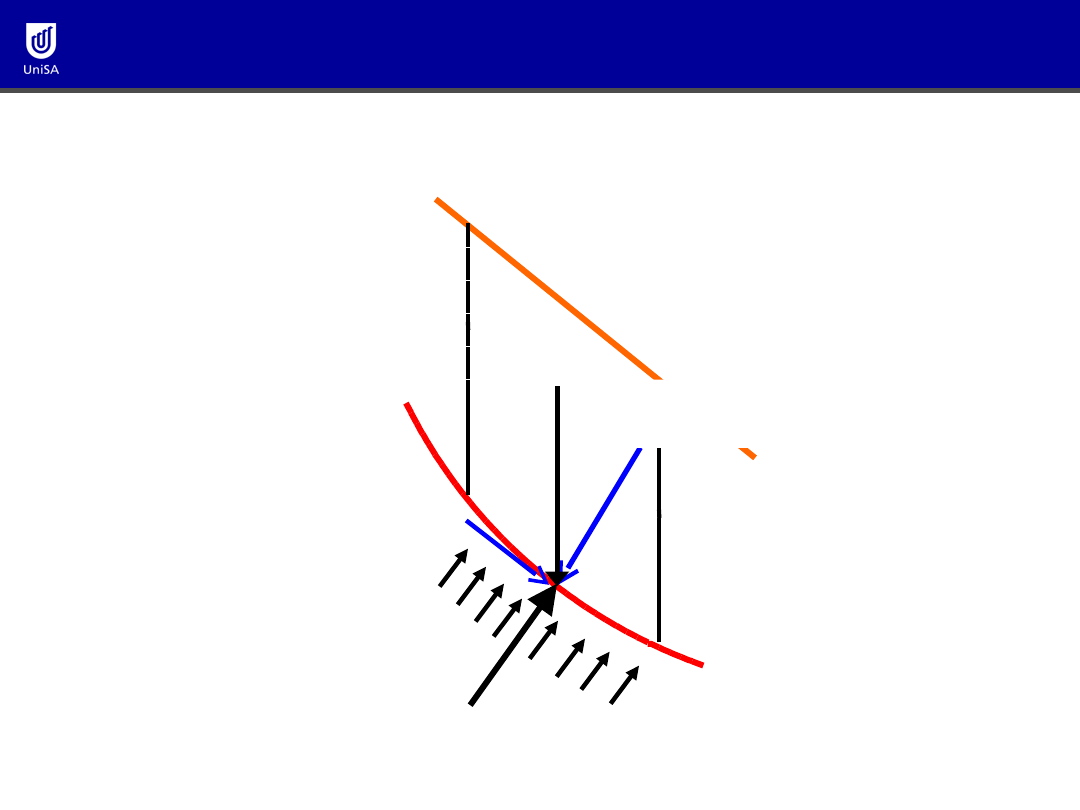
Stability - Case 2:

  0 (stiff clays, sandy

clays, etc)

What do
the green
arrows
now
represent?

Force on Slip Plane:

c',  soil

Nea
r
toe



varies with

position



= c + 

n

tan







Nea
r
cres
t

W

centre of
circle

CIRCULAR SLIPS

“Method of Slices”

A
potential
sliding
surface

1

3

5

7

2

4

6

Reasons for Slices

•

Frictional shear resistance varies

with both 

N

and 

•

Varying cohesion with depth

•

Non-uniform pwp’s from seepage

analysis

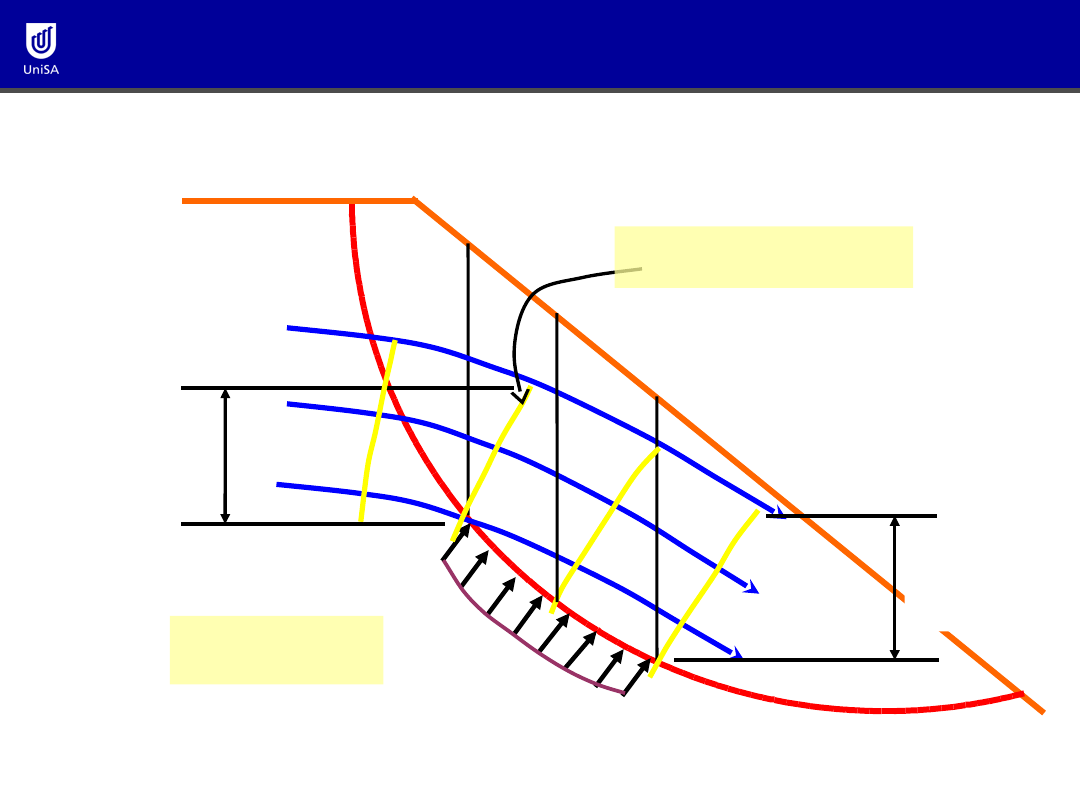
PWP influence

- u values from

flownet

u

i

= 

w

h

wi

h

w8

h

w1

equipotential

General Method of Slices

• FoS by summation over all

slices for trial failure surface

• 100’s of trial surfaces evaluated

 thank you for the pc!

 XSLOPE and GALENA

• Lowest FoS



the “critical failure

surface”

Slice i

centre of
circle

Stability of a

Vertical Slice



i

W

i

b

i

h

i

centre
of circle

Stability of a Slice

(no pwp)

W

i

T

i

N

i

E

i

X

i

E

i+1

X

i+1

W

i

cos

Wsin

x

i

PWP influence

W

i

W

i

cos

W

i

sin

Force U

i

= u

i

l

i

Slices - overall too many unknowns!

- need simplifying assumptions to get a solution!

Side Forces:

• Assumptions re these forces

= differences in methods

e.g. Fellenius v. Bishop’s simplified method

Fellenius Method

Resultant of side forces = zero
i.e. X

i

= X

i+1

and E

i

= E

i+1

For homogeneous soil

For homogeneous soil:

restoring shear force = cL

arc

+ tanN

where, N

i

 = W

i

cos

i

- u

i

l

i

and

l

i

= arc length of slice, i

Factor of Safety - Fellenius

plane

slip

on

force

sliding

force

shear

restoring

F 



















ΣWsin

ul

Wcos

Σ

tan

L

c

F

arc

Warning:

method regarded as simplistic and non-

conservative

Simplified Bishop Method

-

a superior method

•

Resultant of side forces acts horizontally

•

Apply FoS (F) to restoring shear force

T = [l(c+ 

N

tan)]/F

•

Sum all vertical forces

W = [Ncos

+ [(cl+ Ntan)

sin

]/F

]

•

Solve for N

•

Substitute in





















Wsin

tan

N

l

c

FoS

The Bishop Equation





i

i

i

i

i

i

i

sin

ΣW

M

)

tan

b

u

W

b

c

(

F

















































F

tan

tan

1

cos

M

i

i

i

Wher
e

Simplified Bishop Method

• Requires iteration

─ assume initial F, then solve for F

─ when trial F and determined F are equal, it’s a

solution

• Spreadsheet for simple slopes

• XSLOPE & GALENA otherwise

─ 1000 trial surfaces in 1 minute

XSLOPE

(University of Sydney)

Other Methods

• More exact solutions exist, but little

improvement on accuracy

• Choosing the soil shear strength

factors and soil layers are far more
important

What strength should be applied?

• MUST be appropriate to the field

stress levels



stresses may be quite low

• Undrained or Drained



short term (just constructed) or

long term stability?

What strength?

1. Peak strength

- First time slides? Or compacted soils

2. Softened strength (critical state)

-

Fissured, stiff clays?

3. Residual strength

-

Evaluation of stability of slips or pre-existing
slides

-

Bedding shear planes

Typical strength values

•

Peak effective friction angle, 

For NC soils (Kenney 1959)

sin = fn [log(Plastic Index)]

e.g. 30 for PI = 20%: 18 for PI  120%

•

RETAINING WALL STANDARD,

AS4678 – 2002

gives guidance on c-  soils (see lecture notes)

Residual strengths, 

r





r



Clay mineral

5

Montmorillonite

10

Illite

15

Kaolin

London Clay 16 - Skempton (1964)

Numerical Approach to Slopes

FEA or Finite Difference

Benefits:
• Progressive failure

─ shear strength mobilization not uniform

along sliding surface

• Distortions as well as safe slope angle

But more effort

Avoiding Landslip

AUSTRALIAN GEOMECHANICS SOCIETY

Landslide Risk Management (Apr 07)

http://www.australiangeomechanics.org/index.htm

SUMMARY: KEY POINTS

a. Angle of repose for dry granular soils

b. Influence of seepage on granular soils

c. Slope stability for homogeneous slopes in

saturated clay (NC)

i.

simple analyses

ii. Taylor’s charts

d. Frictional soils more difficult

iii. Method of slices

e. Slope stability programs use limit equilibrium

POINTS, continued

f.

Slope stability programs search for the
failure surface with lowest FoS

iv. circular or non-circular slips?

g. Bishop’s simplified method for circular

slips

v. further refinement unwarranted?

h. Importance of shear strength parameters

vi. drained and/or undrained?

vii. peak, ultimate or critical state?

Some web sites

•

http://www.em.gov.bc.ca/Mining/Geolsurv/Surficial/landsli
d/ls2.htm

•

http://landslides.usgs.gov/

•

http://www.ew.govt.nz/enviroinfo/hazards/naturalhazards
/landslide/#Heading1