Ograniczenie wymiarowości w nanostrukturach

półprzewodnikowych.

QUANTUM CONFINEMENT IN SEMICONDUCTOR

NANOSTRUCTURES

In a bulk semiconductor, carrier motion is unrestricted along all three spatial directions. However, a nanostructure has one or more of its dimensions reduced to a nanometer length scale and this produces a quantisation of the carrier energy corresponding to motion along these directions.

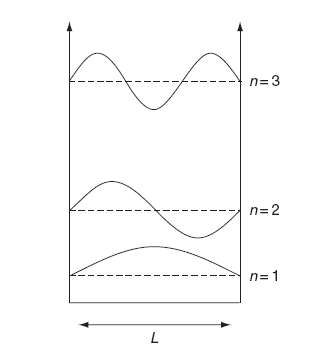
Consider initially an isolated, thin semiconductor sheet of thickness L.

Carrier motion is unrestricted along the two orthogonal directions within the plane of the sheet, but is quantised perpendicular to the plane, forming a one-dimensional quantum well.

The resultant quantised energy levels are found by solving the one-dimensional form of the time-independent Schrodinger equation :

Solving the Schrodinger equation and applying the boundary

condition that the wavefunctions must be zero at the edges of the sheet, results in the following energies and wavefunctions:

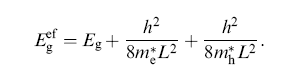
This results in a finite-depth potential well as shown in Figure For a semiconductor quantum well both the electron and hole motion normal to the plane will be quantised, resulting in a series of confined energy states in the conduction and valence bands.

One consequence of this quantum confinement is that the effective band gap of the semiconductor Eefg is increased from its bulk value by the addition of the electron and hole confinement energies

corresponding to the states with n= 1:

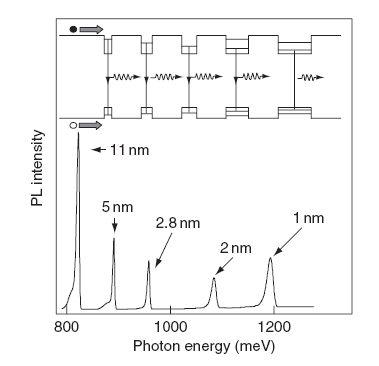
This effective band gap will determine, for example, the energy of emitted photons, and can be altered by varying the thickness of the well.

Each well emits photons of a different energy;

the energy increasing as the width of the well decreases.

Figure shows an example of this behaviour where the emission spectrum of a structure containing five quantum wells of different widths is shown. Each well emits photons of a different energy; the energy increasing as the width of the well decreases,

The total energy for a carrier in the nth confined state is therefore given by

Since k// is unrestricted, this equation gives a continuum of energies for each value of n; these energy bands are known as subbands.

Quantum confinement in two dimensions: quantum wires

A quantum wire consists of a strip of one semiconductor confined within a second, larger band gap barrier semiconductor. Unrestricted carrier motion is now only possible along the length of the wire and is quantised along the two remaining orthogonal directions.

Using the infinite-depth well approximation for the quantized energies, the total energy for a carrier in a quantum wire with z and y

dimensions Lz and Ly respectively is

The total energy depends on the two quantum numbers n and m and

the wave vector for free motion along the wire kx.

Quantum confinement in three dimensions: quantum dots

A quantum dot consists of a small region of one semiconductor totally surrounded by a second, larger band gap barrier semiconductor.

Carrier motion is now quantised along all three spatial directions and there remains no unrestricted carrier motion. For a simple shape such as a cube or cuboid, confinement for the three spatial directions can be considered separately. In the infinite-depth well approximation, the total energy for a carrier in a cuboid-shaped dot of dimensions Lz, Ly and Lx is a function of three quantum numbers n, m and l:

The energy is now fully quantised and the states are discrete, in a manner similar to those of an atom. The shapes of real quantum dots are more complex than simple cuboids and a calculation of the

confined energy levels requires a numerical solution of the relevant Schrodinger equation.