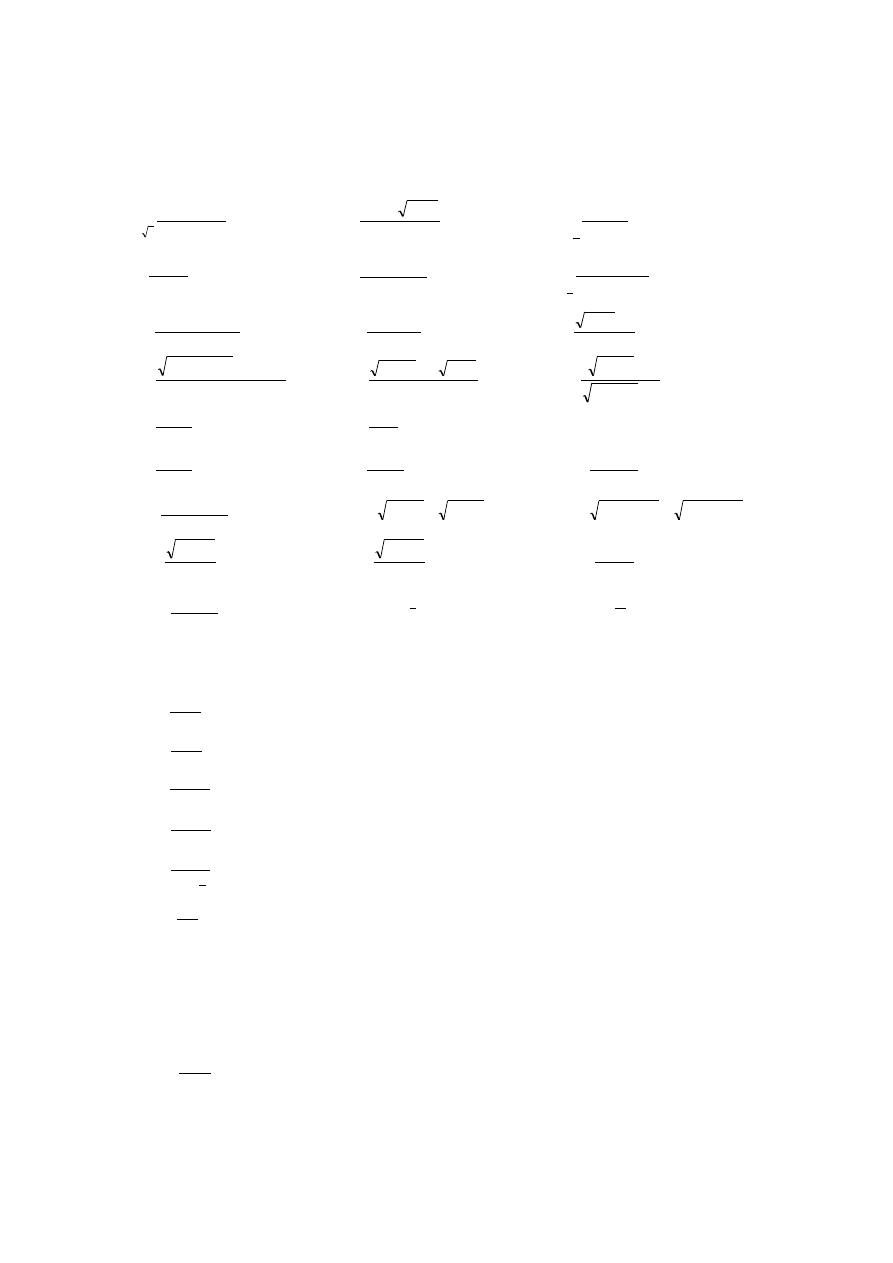
1
Granica i ciągłość funkcji
Zadanie 1
Obliczyć granice funkcji:
1)
1
3
lim
2
4
2
3
x
x
x
x
2)
1
2
1
lim
2
1
x
x
x
x
3)
1
2
1
4
lim
2
2
1
x
x
x
4)
2
8
lim
3
2
x
x
x
5)
1
2
1
lim
2
2
1
x
x
x
x
6)
1
5
6
1
8
lim
2
3
2
1
x
x
x
x
7)
6
2
3
lim
2
2
3
2
x
x
x
x
x
x
8)
50
2
125
lim
2
3
5
x
x
x
9)
2
1
3
lim
2
x
x
x
10)
x
x
x
x
x
)
1
(
2
1
lim
2
0
11)
9
1
2
13
lim
2
3
x
x
x
x
12)
5
25
1
1
lim
2
2
0
x
x
x
13)
x
x
x
4
sin
lim
0
14)
x
x
x
sin
tg
lim
0
15)
x
x
x
ctg
lim
0
16)
x
x
x
3
5
sin
lim
0
17)
x
x
x
5
sin
4
sin
lim
0
18)
2
3
2
1
lim
x
x
x
x
19)
2
2
3
2
1
3
lim
x
x
x
x
20)
1
1
lim
2
2
x
x
x
21)
2
2
1
1
lim
x
x
x
x
x
22)
1
4
lim
2
x
x
x
23)
1
4
lim
2
x
x
x
24)
1
5
2
3
5
3
lim
x
x
x
x
25)
x
x
x
x
x
5
4
lim
2
2
26)
x
x
x
3
0
2
1
lim
27)
1
2
1
0
1
lim
x
x
x
Zadanie 2
Obliczyć granice jednostronne funkcji:
a)
3
1
)
(
x
x
f
w punkcie
3
x
b)
x
x
f
3
1
)
(
w punkcie
3
x
c)
4
1
)
(
2
x
x
f
w punkcie
2
x
d)
4
1
)
(
2
x
x
f
w punkcie
2
x
e)
x
e
x
f
1
1
1
)
(
w punkcie
0
x
f)
3
1
1
)
(
x
e
x
f
w punkcie
1
x
Zadanie 3
Czy niżej podane funkcje są ciągłe w przedziale
)
,
(
?
a)
0
dla
0
0
dla
4
)
(
2
x
x
x
x
f
b)
6
dla
5
6
dla
6
1
)
(
x
x
x
x
f

2
c)
2
dla
2
2
dla
1
0
dla
2
)
(
x
x
x
x
x
f
d)
1
dla
1
1
dla
)
(
x
x
x
x
f
e)
0
dla
)
2
(
0
dla
3
2
)
(
2
x
x
x
x
f
x
f)
0
dla
3
0
dla
1
)
(
x
x
x
x
f
x
g)
1
dla
log
1
dla
1
)
(
x
x
x
x
f
h)
1
dla
log
1
dla
1
)
(
x
x
x
x
x
f
Zadanie 4
Niech
0
dla
0
dla
)
(
x
x
a
x
e
x
f
x
Dla jakiej wartości a funkcja
)
(x
f
będzie funkcją ciągłą w całym przedziale
)
,
(
?
Zadanie 5
Niech
0
dla
0
dla
5
)
(
1
x
a
x
x
f
x
Dla jakiej wartości a funkcja
)
(x
f
będzie funkcją ciągłą w całym przedziale
)
,
(
?
Zadanie 6
Czy można dobrać stałą a tak, aby funkcja
7
1
dla
ln
2
1
dla
1
5
dla
1
)
(
2
x
x
x
a
x
x
x
f
była ciągła w całej swojej dziedzinie?
Wyszukiwarka
Podobne podstrony:
5 Ciagi,granica i ciaglosc funkcji
Granica i ciągłość funkcji
Granica i ciągłość funkcji zadania
3 granica i ciaglosci funkcji i Nieznany (2)
Granica i ciągłość funkcji
GRANICE I CIAGLOSC FUNKCJI, Inżynieria środowiska
Granice i ciaglosc funkcji, IB Nieznany
Granica i ciągłość funkcji
Arkusz zadan Granice i ciaglosc funkcji id 6 (2)
GRANICE I CIĄGŁOŚC FUNKCJI
3 Granica i ciągłość funkcji
granica i ciaglosc funkcji
granica i ciaglosc funkcji id 1 Nieznany
analiza GRANICE I CIAGLOSC FUNKCJI
Granice i ciaglosc funkcji id 1 Nieznany
granica i ciaglosc funkcji zespolonych
W 2 Granica i ciągłość funkcji, Informatyka ns 2009-2013, Semestr I, Analiza Matematyczna
5 Ciagi,granica i ciaglosc funkcji
Granica i ciągłość funkcji
więcej podobnych podstron