Bioleaching kinetics

Lecture #5

Biooxidation versus chemical

oxidation

• The rate of bacterial oxidation is

higher than that of chemical
oxidation under the same
conditions.

Model of leaching kinetic

• The leaching of mineral particles by a

reagent in solution can be
represented by the reaction:

aA

(s)

+ bB

(aq)

 aqueous and solid

products

A – the solid undergoing leaching
B – the reagent in solution
„a” and „b” are stoichiometric coefficients

Subprocesses in

biooxidation

• Growth bacteria
• Ferrous to ferric oxidation Fe

2+

Fe

3+

• Disolved oxygen consumption O

2

H

2

O

• Disolved carbon dioxide consumption

CO

2

The rtes of oxygen and carbon dioxide
utilization are measured by gas
analyzers.

Subprocess

• The bacterial oxidation of ferrous (Fe

2+

) to

ferric (Fe

3+

) ions.

• The kinetic of bacterial growth was

described by Monod equation:

 

max

-maximum of specific growth rate [1/h]

• K

S

– Monod’s constant [mol/L]

• c

S

- substrate concentration [mol/L]

S

S

c

K

c

S





max





Kinetic equations

• The rate of oxygen utilization is correlated

to ferric to ferrous ratio:

• The rate of ferrous iron utilization:

 













2

3

max

2

2

2

1

Fe

Fe

K

q

q

O

O

O

 



















2

3

max

2

2

2

1

Fe

Fe

K

q

q

Fe

Fe

Fe

Kinetic parameters for Fe

2+

oxidation

Acidithibacillus

ferrooxidans

Leptospirillum

ferrooxidans

Units

q

O2

max

2.2

1.7

molO

2

/

molC/h

q

Fe2+

max

8.8

6.8

molFe

2+

/

molC/h

K

O2

0.05

0.0005

K

Fe2+

0.05

0.0005

Logistic model

• The logistic equation is written for the

rate of conversion of sulfide mineral [X].

where: k

m

rate constant.

• The fraction bioleached with time is

given by integrating logistic equation.

















max

1

X

X

X

k

dt

dX

m

 





t

k

t

k

m

m

e

X

X

e

X

t

X







1

1

max

0

0

Kinetic of (bio-) leaching

process

• The kinetic of leaching reaction are

described by:

The shrinking core modeel
The shrinking particle model

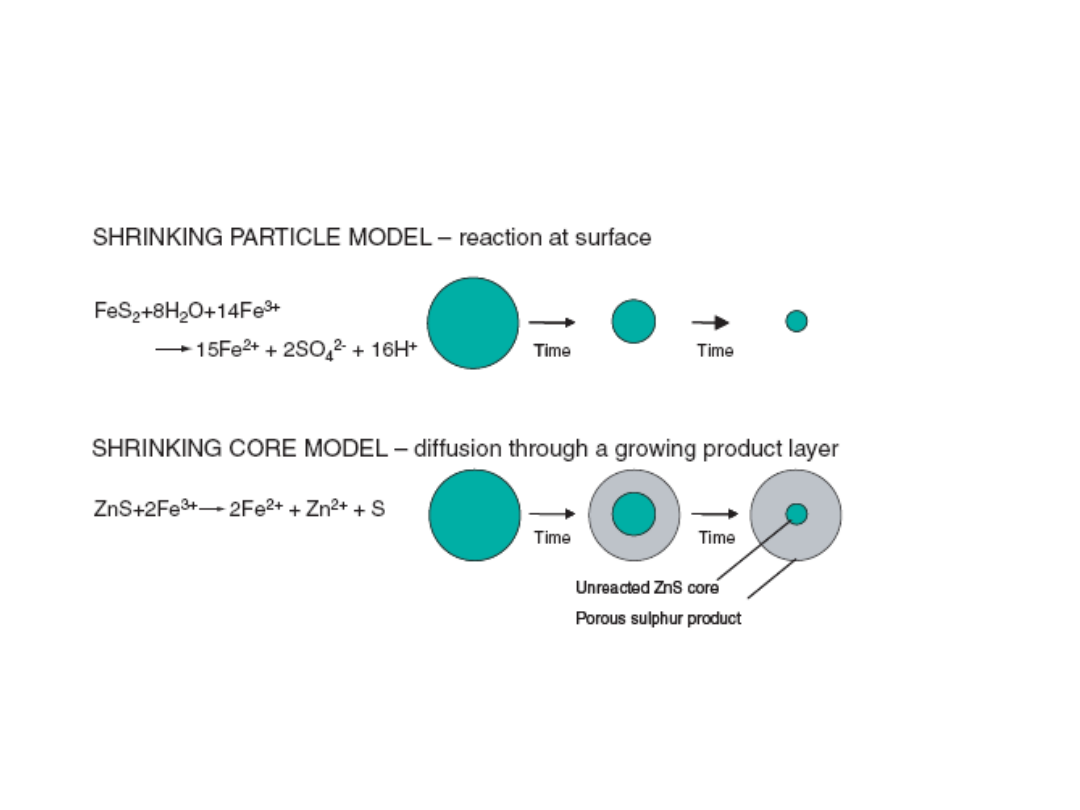
Shrinking particle and core

models

Kinetic of bioleaching

• The rate of the heterogeneous

reaction is controlled by:
1. film diffusion

2. chemical reaction

3. product layer diffusion

 















































x

x

t

k

x

t

k

x

t

k

1

2

1

3

1

1

1

3

2

3

3

1

2

1

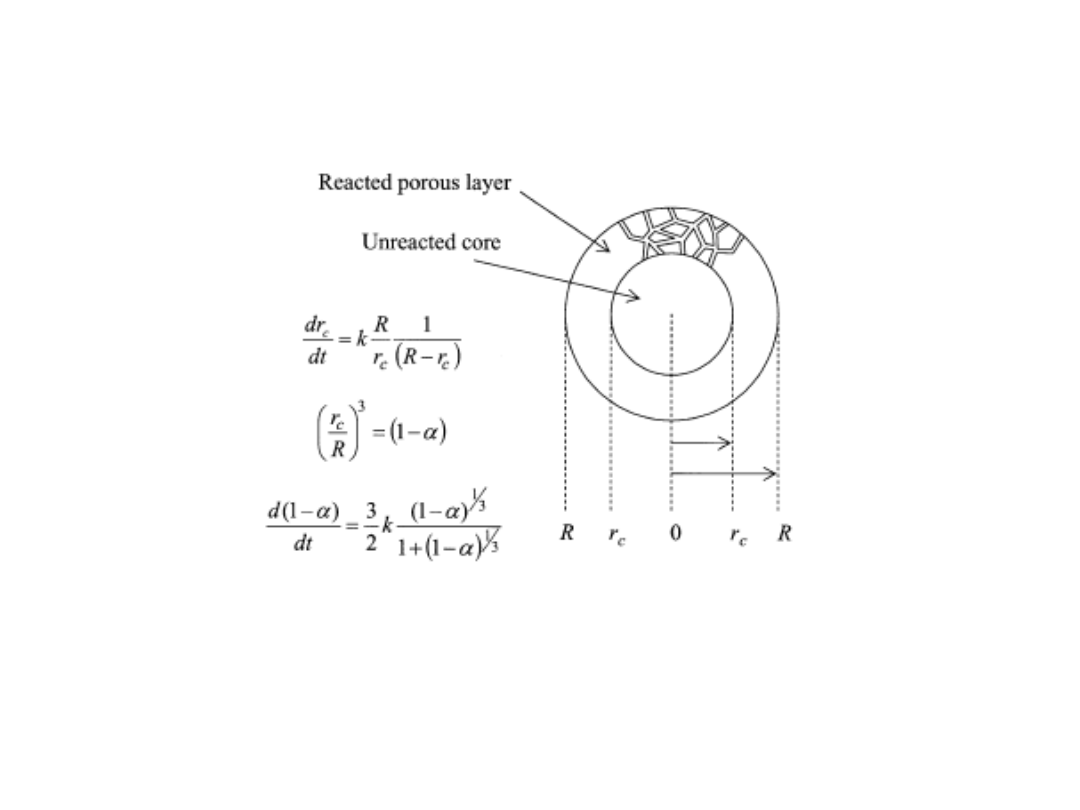
Shrinking core model

1-(2/3)α-(1-α)

2/3

=kt

α-is the fraction of leached

k-is the rate constant (1/day)

t –is time (day)

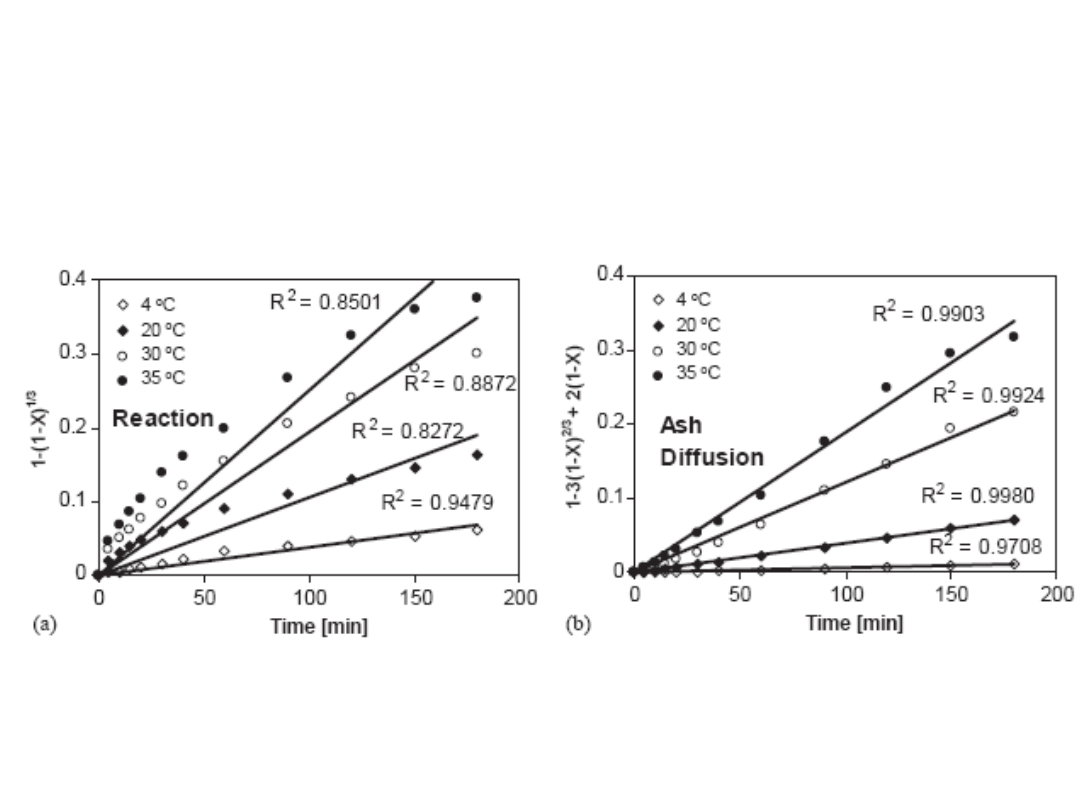
Shrinking particle and core

models

[1-(1-X)

1/3]

versus time

[1+2(1-X)-3(1-X)

2/3

] versus

time

where X is fractional conversion

Bacteria oxidation

• According to the model proposed by Michaelis-

Mentan the dissolution rate is given by following

equation:

where: V is the extraction rate of metal
V

max

is the maximum metal extraction rate

K

s

is the Michaelis’ constant

S is the pulp density
K

s

constant gives an idea aobout the efficiency of

bacteria to the mineral surface.





S

K

S

V

V

s





max

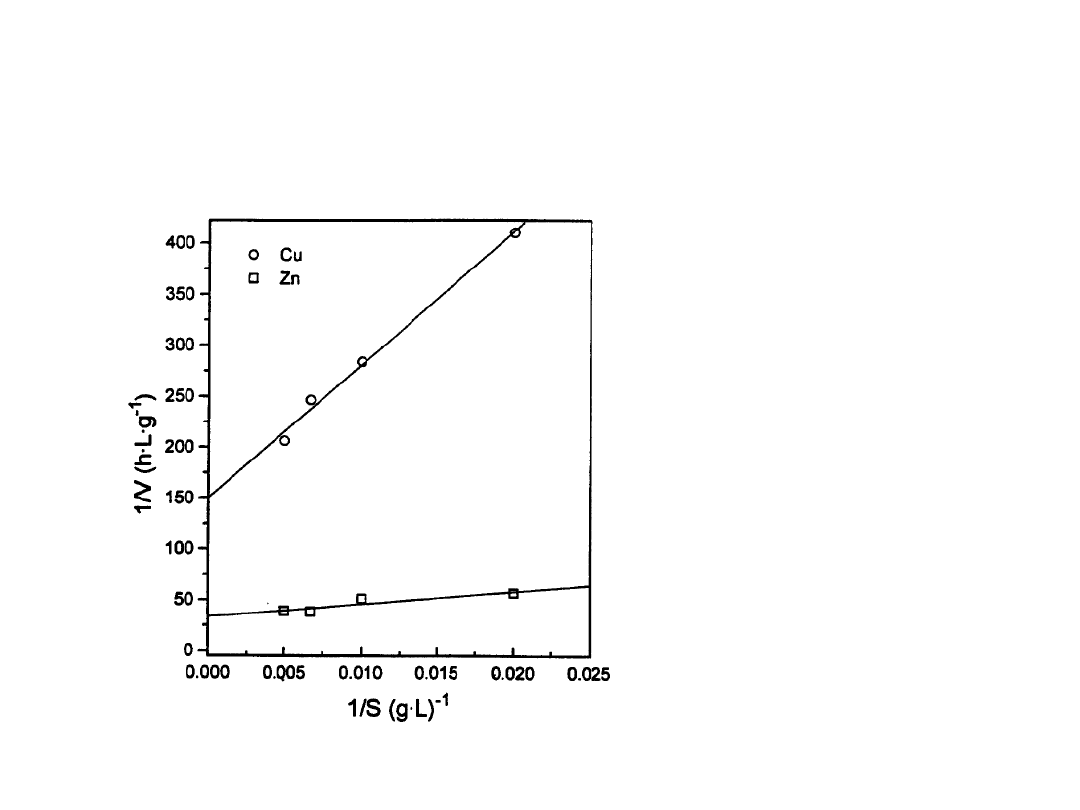
Plot of Michaelis-Menten for the copper

and zinc dissolution

Plot of 1/V versus
1/S for Cu and Zn

The higher rate K

S

for Cu then K

S

for

Zn indicates a
preference of
bacteria for copper.

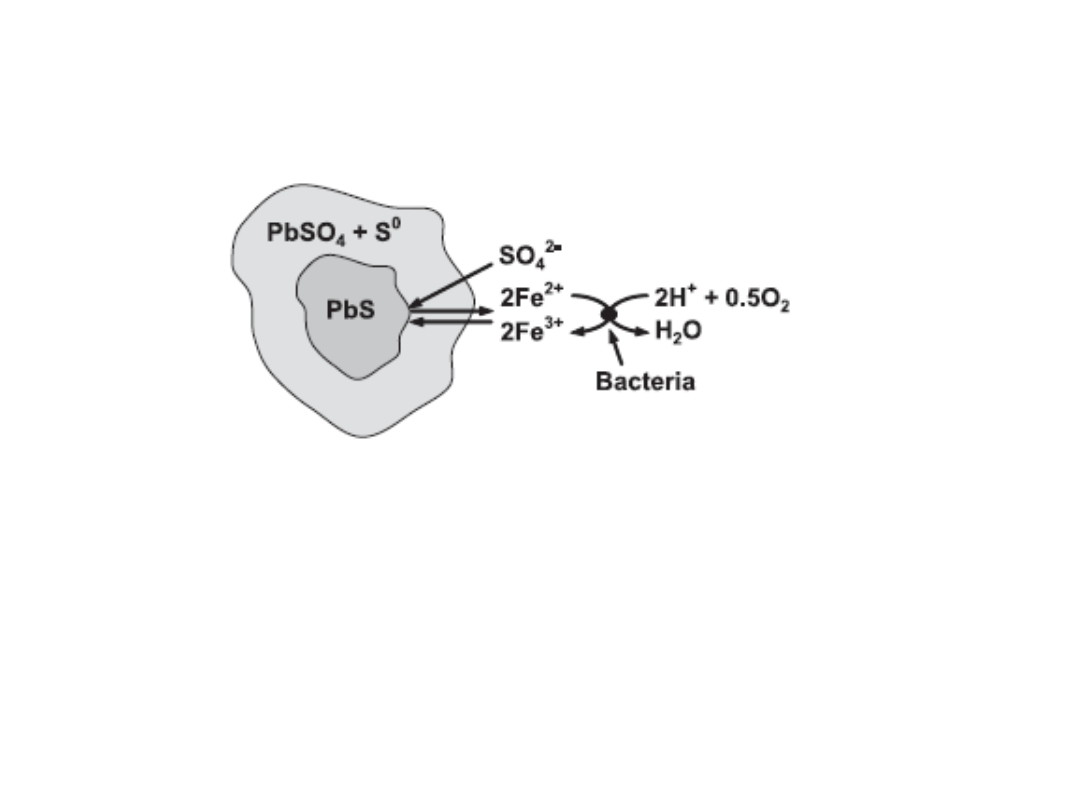
Indirect mechanism of bioleaching of

galena

PbS

(s)

+ 2Fe

3+

(aq)

 Pb

2+

(aq)

+S

0

(s)

+ 2 Fe

2+

(aq)

Pb

2+

(aq)

+ SO

4

2-

(aq)

 PbSO

4(s)

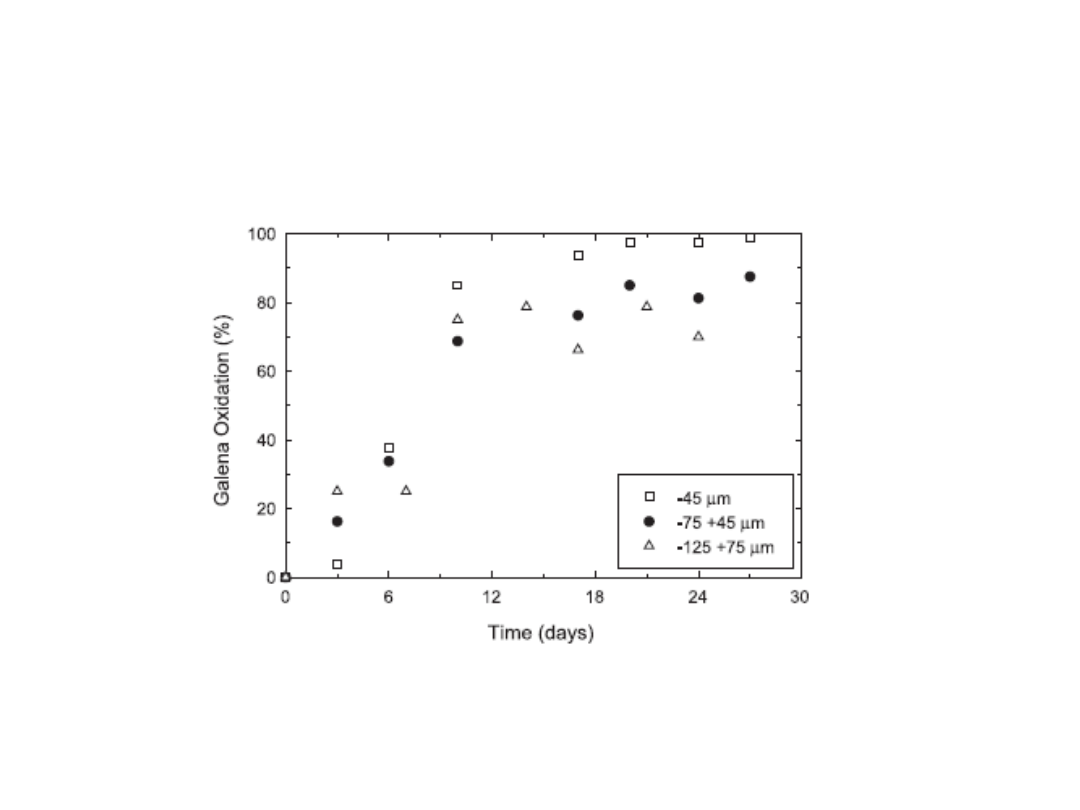
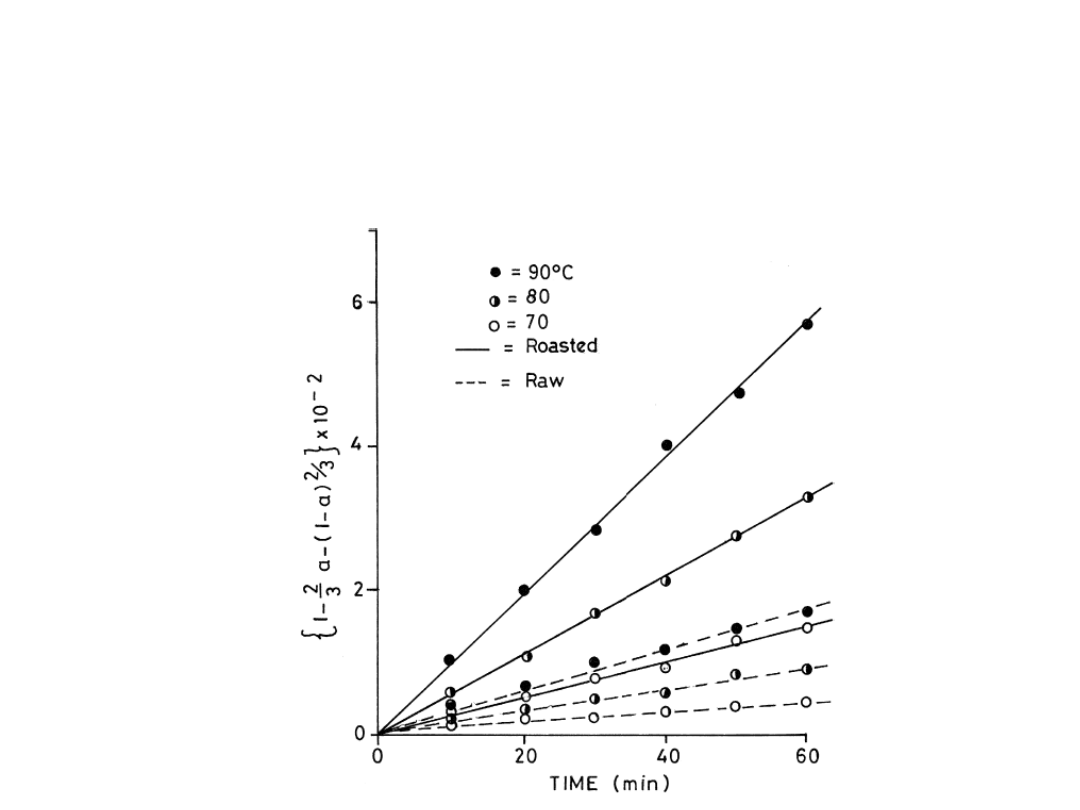
Galena bioelaching

Effect of particle size on the bioleaching of
galena

Microphotograph (magnification of 50x0 of a

partially oxidized galena particle

a) unreacted galena

b) lead sulfate/elemental sulfur product layer