The Uncertainty Principle
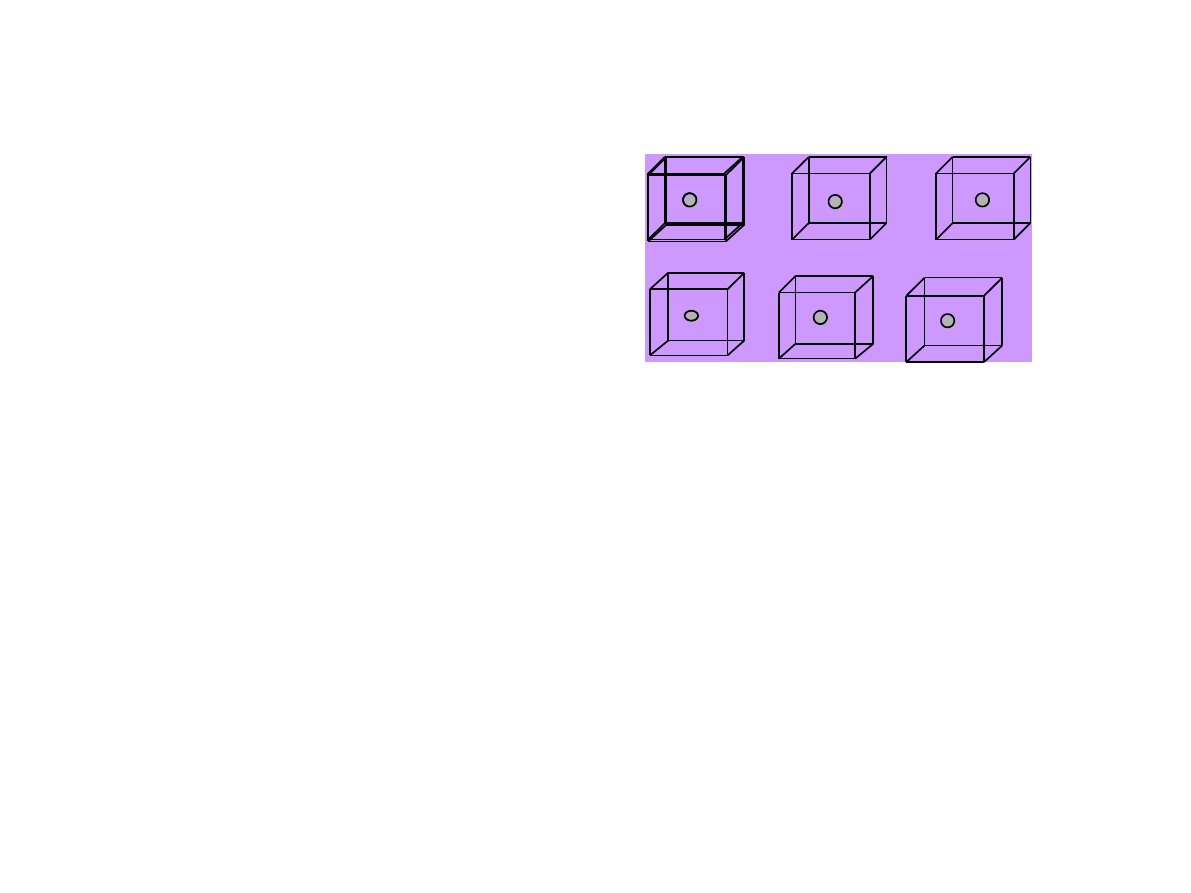
Consider a large number N of
identical boxes with identical
particles all described by the
same statefunction
Ψ
(x,y ,z) :
Consider the observable A represented by the operator ˆ
A
Let [ˆ
A , ˆ
H ]
≠
0
Thus the system described by
Ψ
do not
have sharp value for A.
The average (expectation ) value is defined by :
< A >=
Ψ
*
∫
ˆ
A
Ψ
d
τ
Appendix B
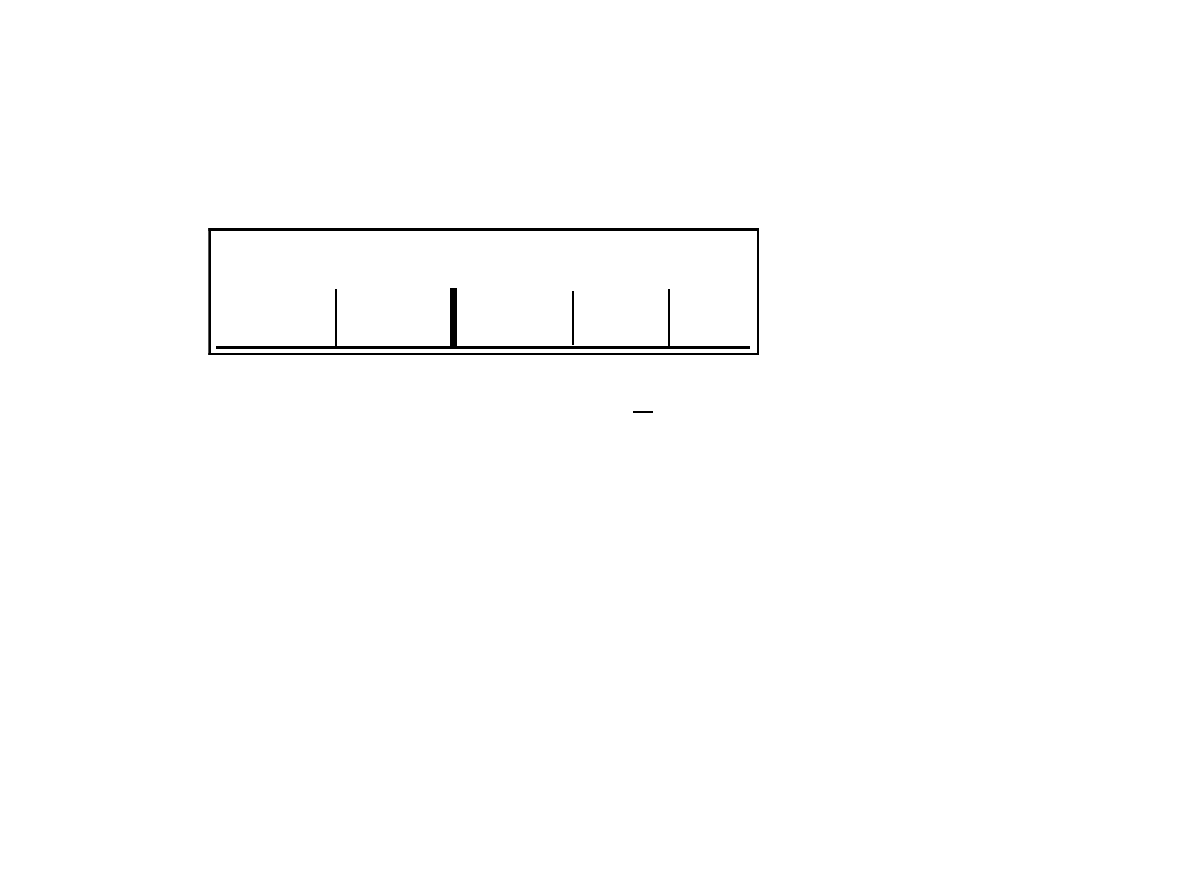
<A>
A1
A2 An
The Uncertainty Principle
The measurement of A on each of the n identical
systems will give a different outcome A
i
We define the variance as :
1
n
i
∑
A
i
− <
A
>
(
)
2
=
σ
A
2
=
(
∆
A)
2
σ
A
2
= Ψ
*
( ˆ
A
∫
− <
A
>
)
2
Ψ
d
τ
= Ψ
*
( ˆ
A
2
∫
−
2
<
A
>
ˆ
A
+ <
A
>
2
)
Ψ
d
τ
= Ψ
*
ˆ
A
2
∫
Ψ
d
τ −
2
<
A
> Ψ
*
∫
ˆ
A
Ψ
d
τ+ <
A
>
2
Ψ
*
∫
Ψ
d
τ
= Ψ
*
ˆ
A
2
∫
Ψ
d
τ
− <
A
>
2
=
<
A
2
> − <
A
>
2
Appendix B
The Uncertainty Principle
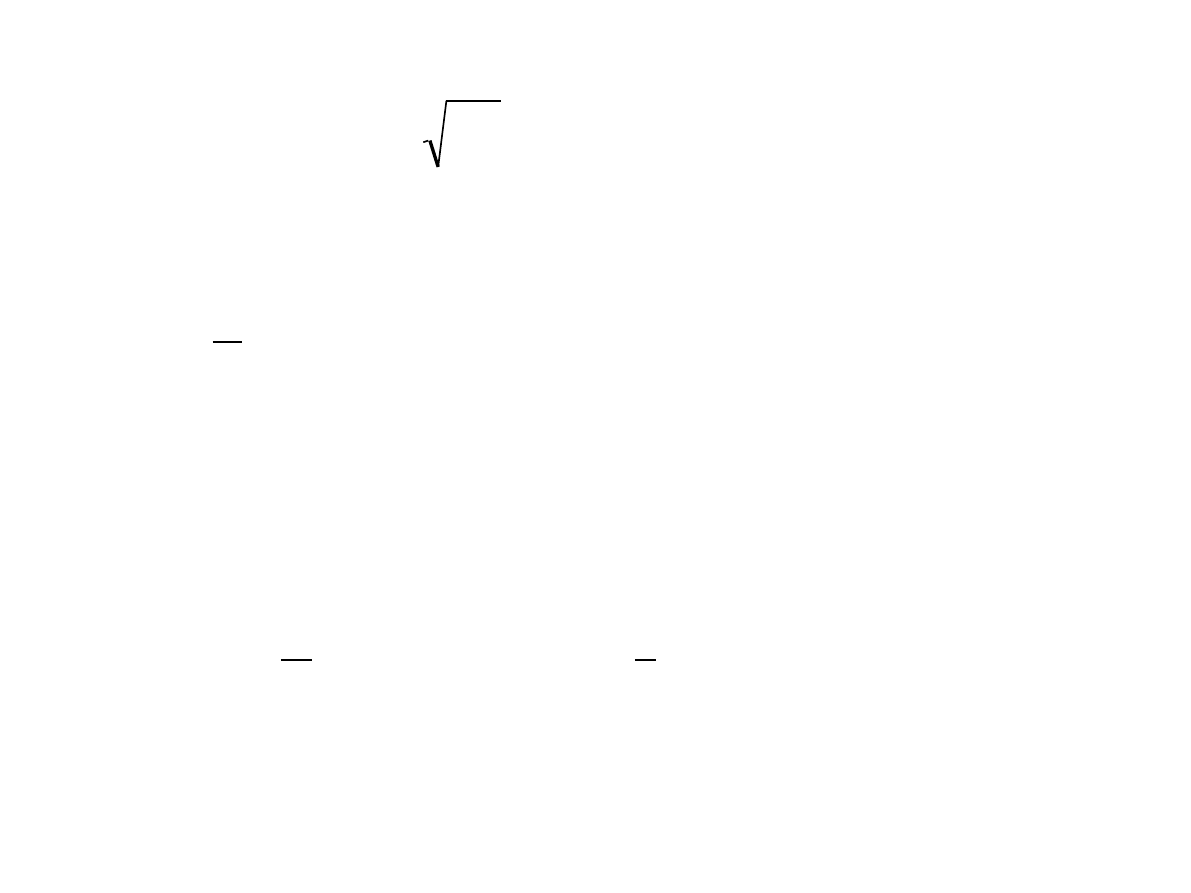
We define :
∆
A =
σ
A
2
as the standard deviation
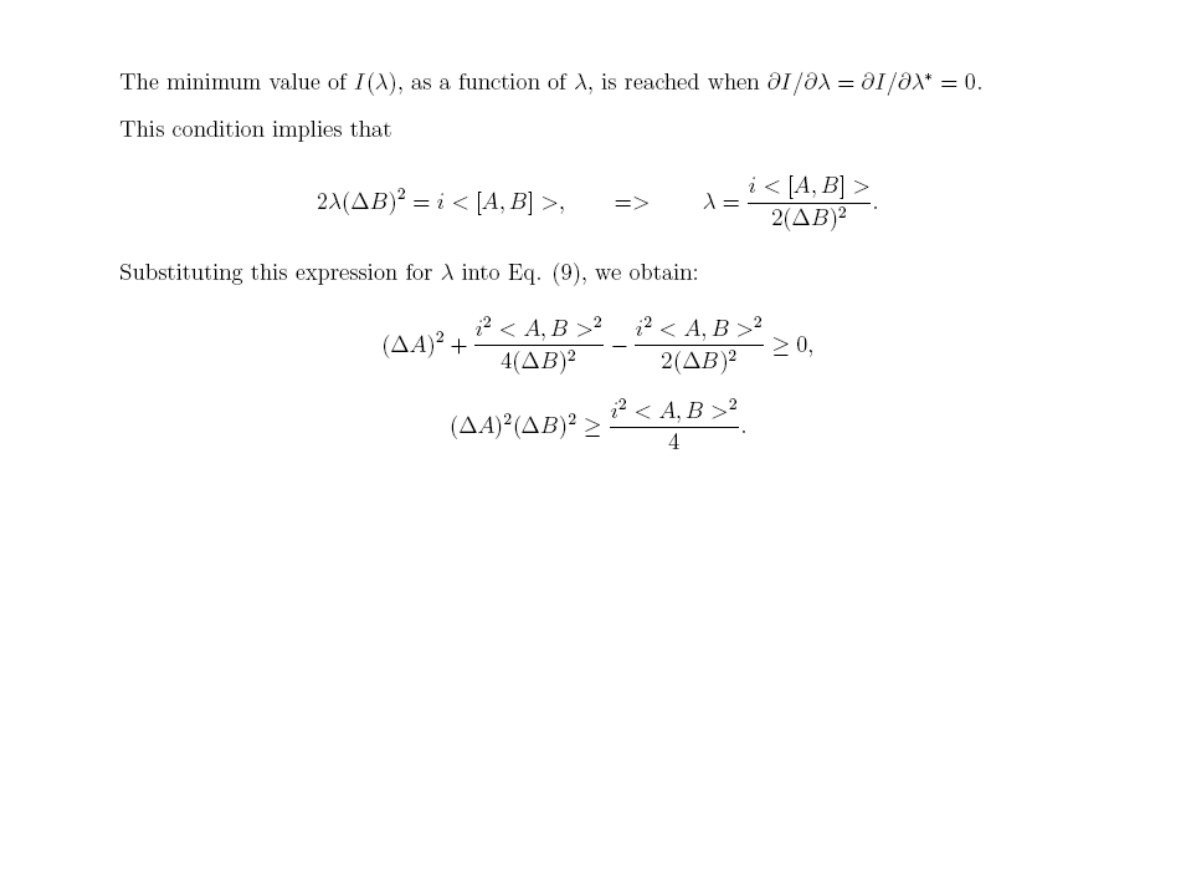
We shall later show that two for two observables A and B
∆
A
∆
B =
1
2i
Ψ
*
∫
[ ˆ
A , ˆ
B ]
Ψ
d
τ
Consider as an example ˆ
x and ˆ
p
x
[ˆ
x , ˆ
p
x
]
=
ih
Since :
∆
x
∆
p
x
=
1
2i
Ψ
*
∫
[ˆ
x , ˆ
p
x
]
Ψ
d
τ
=
1
2
h
We can not simultaniously obtain sharp values
for x and p
x
Appendix B
