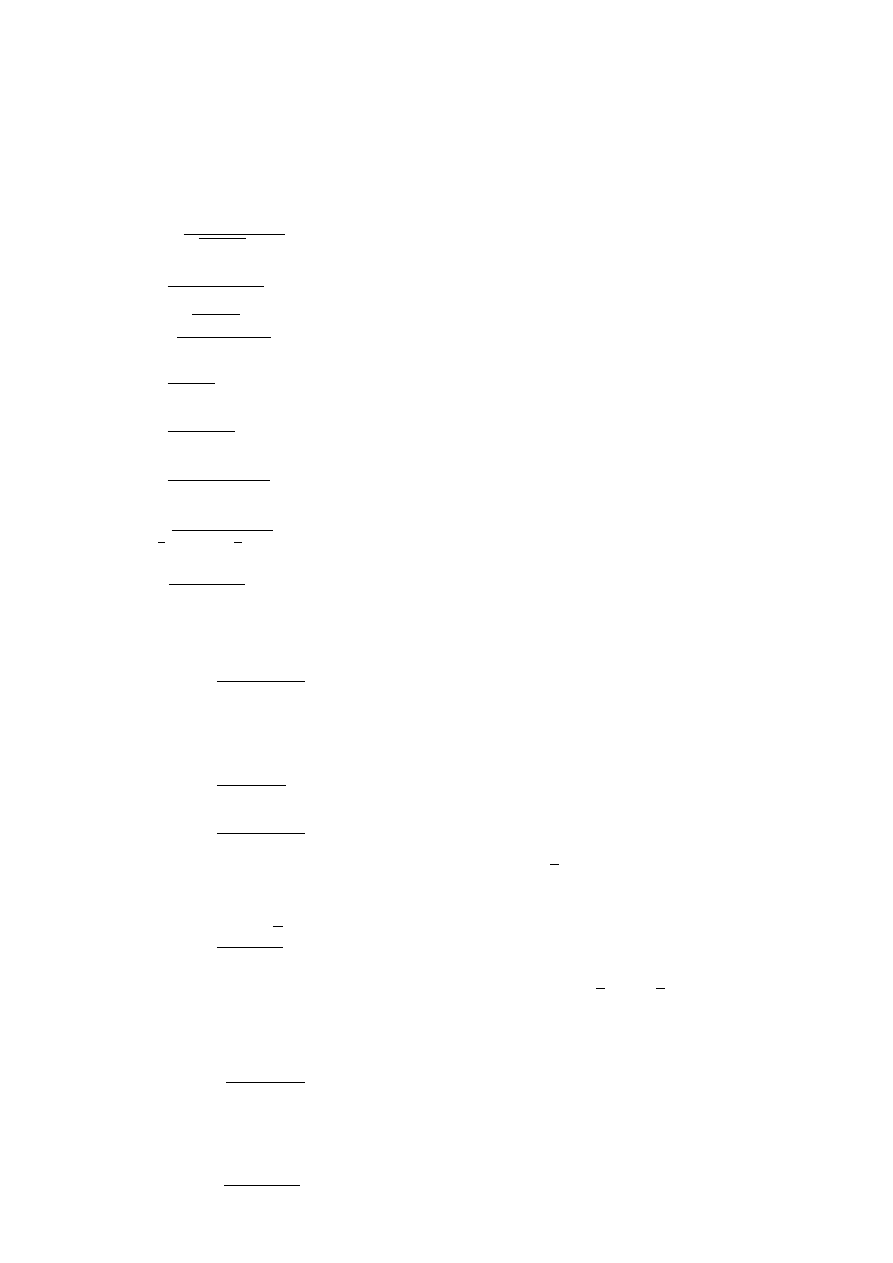
Analiza 1 - ćwiczenia SIMR 2010/2011 ćw 2 - 2 godz.
Temat: granica funkcji, pochodna

1. Obliczyć granicę funkcji:

(a)

lim

x→−∞

x

3

+ x

√

x

6

+ 4 + x

2

(b) lim

x→2

x

2

+ 4x − 12

x

3

− 8

(c) lim

x→−1

√

x

2

+ 8 − 3

x + 1

(d) lim

x→0

x sin x

sin

2

x

(e) lim

x→0

sin

2

x

cos x − 1

(f) lim

x→0

sin(x

2

+ x

3

)

tg(2x

2

+ 3x

3

)

(g) lim

x→

π

2

sin 2x + cos x

x −

π

2

(h) lim

x→π

sin

2

x

1 + cos 5x

2. Dla jakich wartości parametrów funkcja f : R → R jest ciągła:

(a) f (x) =















































x

3

+ 2x − 3

x

2

+ x − 2

dla x > 1

ax + b

dla 0 ¬ x ¬ 1

ln(1 − x)

x

dla x < 0

Odp: a = 2 , b = −1

(b) f (x) =



























x

2

+ ax − 6

x

2

− 4

dla x > 2

b

dla x ¬ 2

Odp: a = 1 , b =

5

4

(c) f (x) =







































x + a

√

x

x + sin x

dla x > 0

bx + c

dla − 1 ¬ x ¬ 0

x

2

dla x < −1

Odp: a = 0 , b = −

1

2

, c =

1

2

(d) f (x) =











































a

x

2

− x + 2

x

2

+ x − 2

dla x > 2

x

dla 0 ¬ x ¬ 2

b

1 − cos 2x

4x

2

dla x < 0

Odp: Funkcja jest zawsze nieciągła.

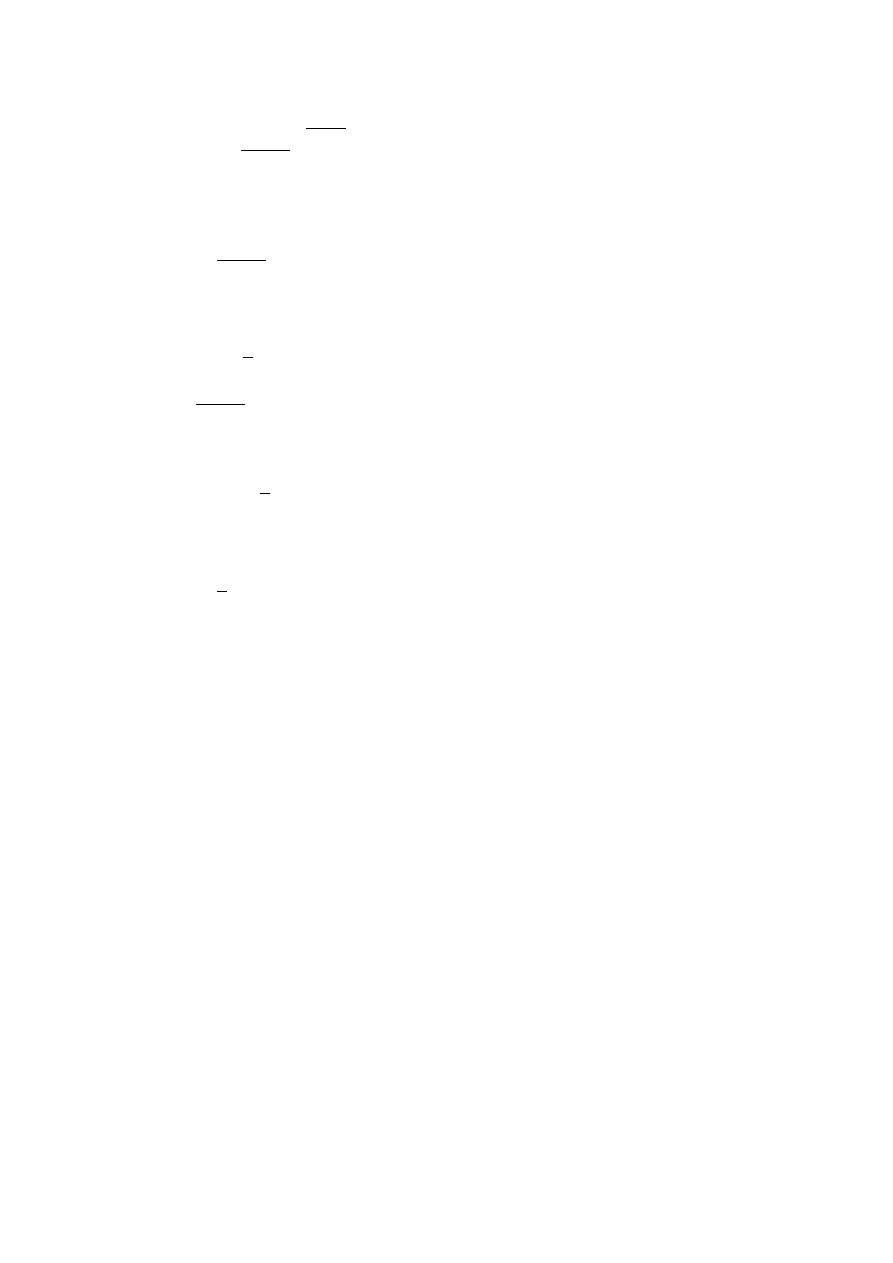
(e) f (x) =























































a

x

2

2x − 1

!

1

1 − x

dla x > 1

b

dla − 1 ¬ x ¬ 1

x

4

+ x

x + 1

dla x < −1

Odp: a = −3 , b = −3

3. Obliczyć pochodną funkcji f (x) :

(a) f (x) = x

2

cos

1

x

(b) f (x) =

x

2

+ 1

x

2

− x

(c) f (x) = xe

x

2

(d) f (x) = ln (x

3

sin(2x + 1))

(e) f (x) = arc sin

√

x + (1 + x

4

) arc tg(x

2

)

4. Dla jakich wartości parametrów funkcja f : R → R jest różniczkowalna:

(a) f (x) =



































1

x

dla x > 1

ax + b

dla 0 ¬ x ¬ 1

cx cos x + d

dla x < 0

(b) f (x) =































a ln x

2

+ 1

dla x > 1

2x + b

dla 0 ¬ x ¬ 1

sin cx + d

dla x < 0