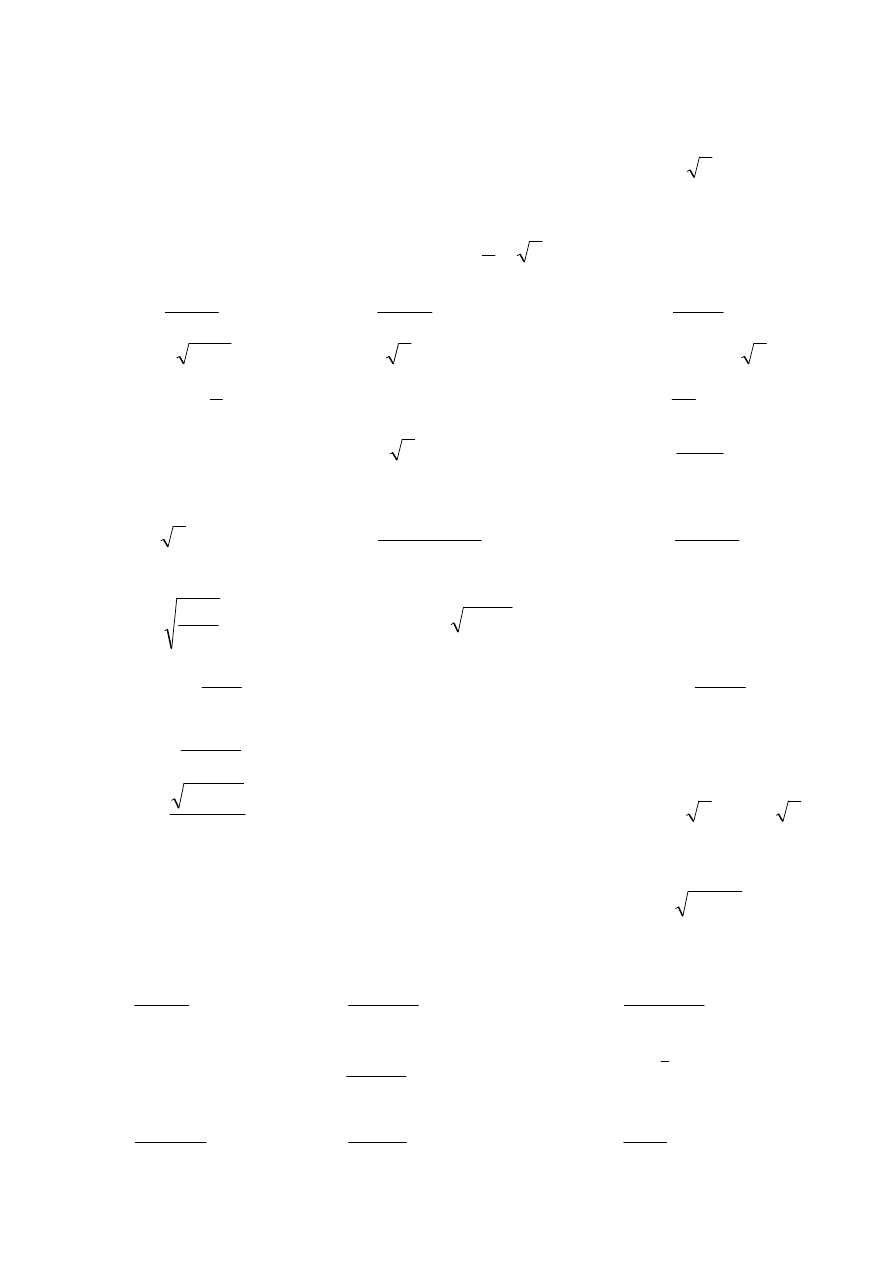
ZESTAW 6. Pochodna funkcji. Reguła de l’Hospitala
Zadanie 6.1. Korzystając z definicji obliczyć pochodną funkcji f w punkcie
0
x
a)
2
,
4
2
)
(
0
2
3
=
−
+
=
x
x
x
x
f
b)
1
,
)
(
0
3
=
=
−
x
x
x
f
c)
4
,
)
(
0
=
=
x
x
x
x
f
Zadanie 6.2. Obliczyć pochodne następujących funkcji
a)
4
)
(
=
x
f
b)
x
x
x
x
x
f
+
−
+
=
2
2
)
(
3
c)
2
4
3
4
)
(
x
x
x
f
−
=
d)
x
x
x
f
−
+
=
1
3
4
)
(
e)
2
4
)
(
2
2
+
=
x
x
x
f
f)
1
3
)
(
4
−
=
x
x
f
g)
3
)
(
+
=
x
x
x
f
h)
)
2
)(
1
(
)
(
1
−
+
=
−
x
x
x
f
i)
)
2
(
4
)
(
3
3
x
x
x
f
−
=
j)
x
x
x
x
f
e
)
1
(
)
(
2
+
=
k)
5
4
)
(
+
=
x
x
f
l)
x
x
x
f
3
4
)
(
=
m)
x
x
x
f
ln
)
(
=
n)
3
ln
2
2
)
(
+
−
=
x
x
x
f
o)
x
x
x
f
+
=
2
ln
4
)
(
p)
x
x
f
2
log
)
(
2
=
r)
x
x
x
f
cos
sin
)
(
+
=
s)
x
x
x
f
cos
)
(
3
=
t)
x
x
x
f
sin
)
(
=
u)
x
x
x
x
x
f
cos
sin
cos
sin
)
(
−
+
=
v)
2
3
sin
2
)
(
2
+
=
x
x
x
f
w)
x
x
x
f
arcsin
)
(
+
=
x)
x
x
x
f
arccos
arcsin
)
(
+
=
y)
x
x
x
f
arctg
)
(
=
z)
x
x
x
f
+
−
=
1
1
)
(
aa)
)
e
1
e
ln(
)
(
x
x
x
f
+
+
=
ab)
5
2
e
)
(
−
=
x
x
f
ac)
x
x
x
f
−
=
1
sin
)
(
ad)
4
2
)
3
2
(
)
(
−
=
x
x
f
ae)
6
2
1
1
)
(
+
+
=
x
x
x
f
af)
3
ln
1
ln
)
(
+
=
x
x
x
f
ag)
)
5
3
cos(
)
(
2
x
x
x
f
+
=
ah)
x
x
f
5
sin
)
(
=
ai)
3
2
5
1
3
)
(
x
x
x
f
+
=
aj)
1
2
3
)
3
4
(
)
(
+
⋅
+
=
x
x
x
f
ak)
)
sin
1
(
)
(
3
x
x
x
f
+
=
Zadanie 6.3.
Obliczy
ć
pochodne
f
′
,
f
′′
,
f
′′′
dla podanych funkcji
a)
x
x
x
f
ln
)
(
2
=
b)
x
x
x
f
sin
)
4
(
)
(
3
+
=
c)
2
)
(
2
+
=
x
x
f
Zadanie 6.4.
Korzystaj
ą
c z reguły de l’Hospitala obliczy
ć
nast
ę
puj
ą
ce granice
a)
8
4
lim
3
2
2
−
−
→
x
x
x
b)
x
x
x
)
1
ln(
lim
0
+
→
c)
2
0
1
lim
x
x
e
x
x
−
−
→
d)
x
x
x
ln
lim
0
+
→
e)
e
1
ln
lim
e
−
−
→
x
x
x
f)
)
1
e
(
lim
1
−
−∞
→
x
x
x
g)
4
0
cos
1
lim
x
x
x
−
→
h)
x
x
x
x
cos
sin
lim
0
→
i)
3
2
ln
lim
x
x
x
∞
→
PDF Created with deskPDF PDF Writer - Trial :: http://www.docudesk.com

j)
x
x
x
e
lim
∞
→
k)
−
−
+
→
x
x
x
x
ln
1
1
lim
1
l)
x
x
x
ln
1
)
1
(
lim
−
+
→
m)
2
1
0
)
1
(
lim
x
x
x
+
+
→
n)
1
1
)
1
2
(
lim
+
+
∞
→
x
x
x
o)
)
ln
(
lim
2
x
x
x
−
∞
→
PDF Created with deskPDF PDF Writer - Trial :: http://www.docudesk.com
Wyszukiwarka
Podobne podstrony:
Geometria krzywych i powierzchni, zestaw 9
Geometria krzywych i powierzchni, zestaw 11
Geometria krzywych i powierzchni zestaw 9
Geometria krzywych i powierzchni zestaw 11
Geometria krzywych i powierzchni zestaw 7
Geometria krzywych i powierzchni zestaw 14
Geometria krzywych i powierzchni zestaw 8
Geometria krzywych i powierzchni, zestaw 7
Geometria krzywych i powierzchni, zestaw 13
Geometria krzywych i powierzchni, zestaw 10
Geometria krzywych i powierzchni, zestaw 12
Geometria krzywych i powierzchni, zestaw 9
Geometria krzywych i powierzchni, zestaw 11
Geometria krzywych i powierzchn Nieznany
Geometria krzywych i powierzchn Nieznany
geometria-krzywe-i-powierzchnie-notatki-z-wykladu 3
GK 8 Modelowanie krzywych i powierzchni(1)
geometria krzywe i powierzchnie notatki z wykladu 3
Fraktalny Rendering Krzywych i Powierzchni p26
więcej podobnych podstron