Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

1/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

STATICALLY DETERMINED PLANE BAR

STURCTURES

(TRUSSES)

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

2/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

Fram
e

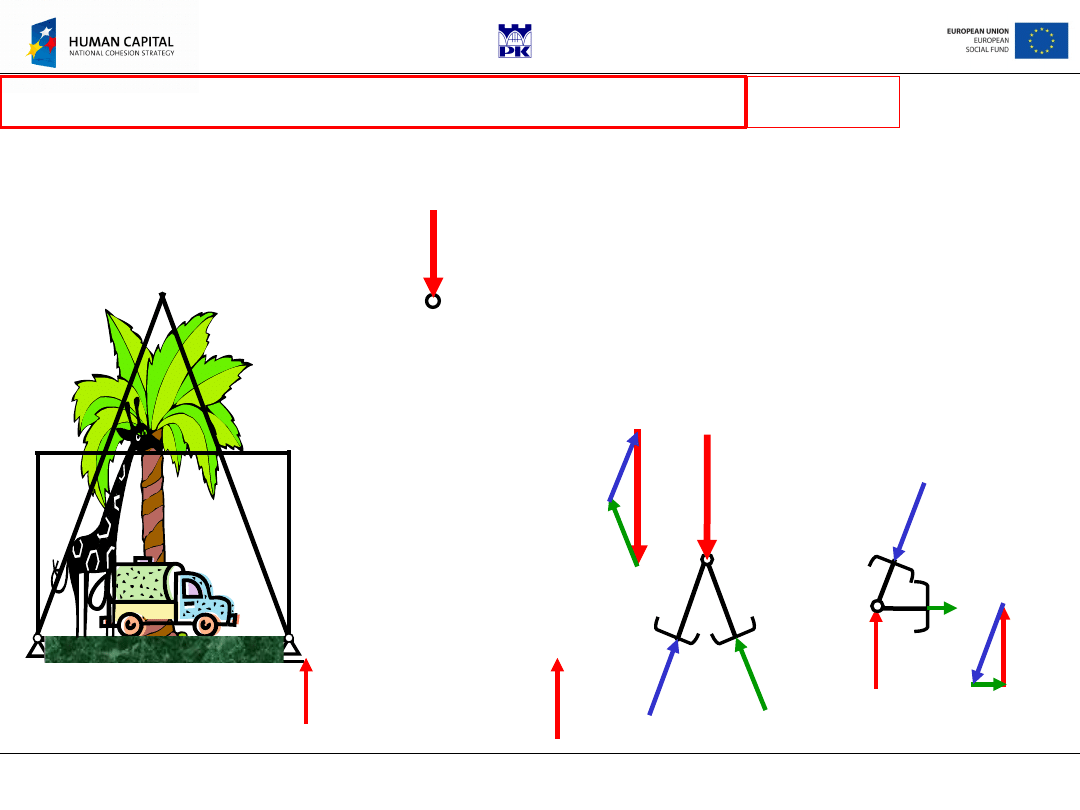
The simplest truss consist of
three bars connected in
hinges.

If the only loading will be forces acting at
hinges then only cross sectional will be
normal forces which can be found
considering equilibrium of hinges.

Formal definition:
A frame is a plane (2D) set of straight bars connected at hinged joints (corners) loaded
at hinges by concentrated forces

Truss

Unstabl

e!

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

3/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

Frame

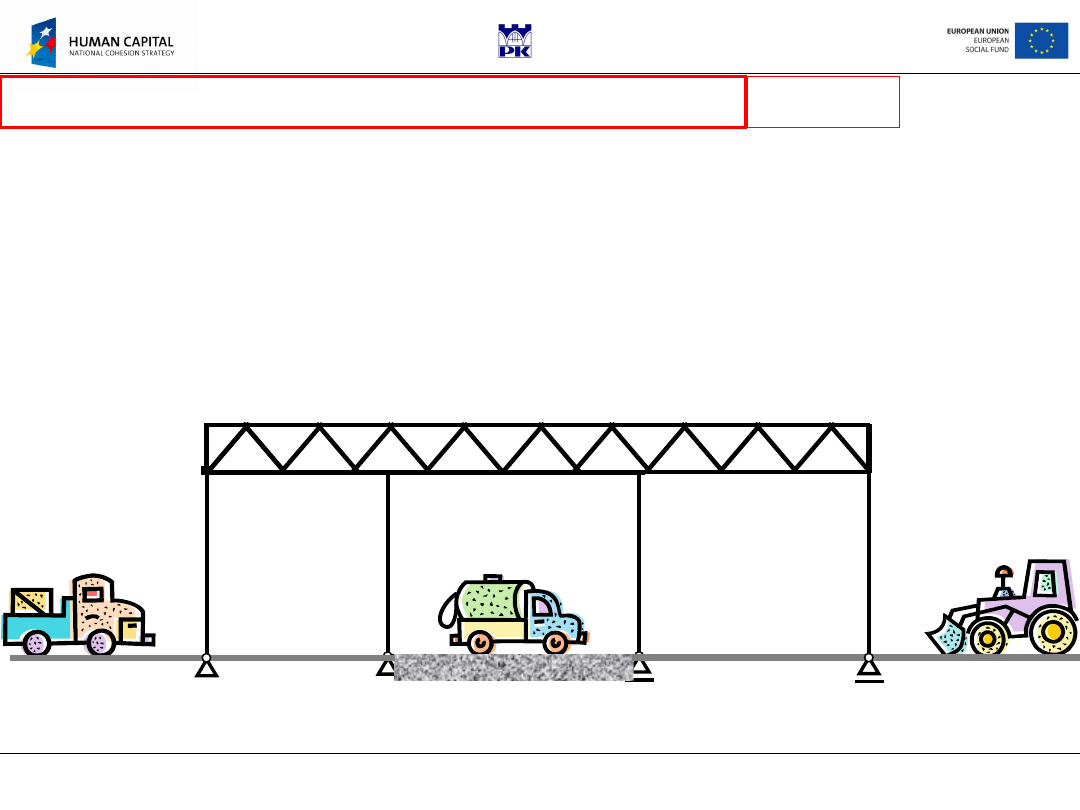
Motivation to use trusses is quite different

Truss

Trusses are aimed to span large areas with a light but durable
structures

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

4/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

5/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

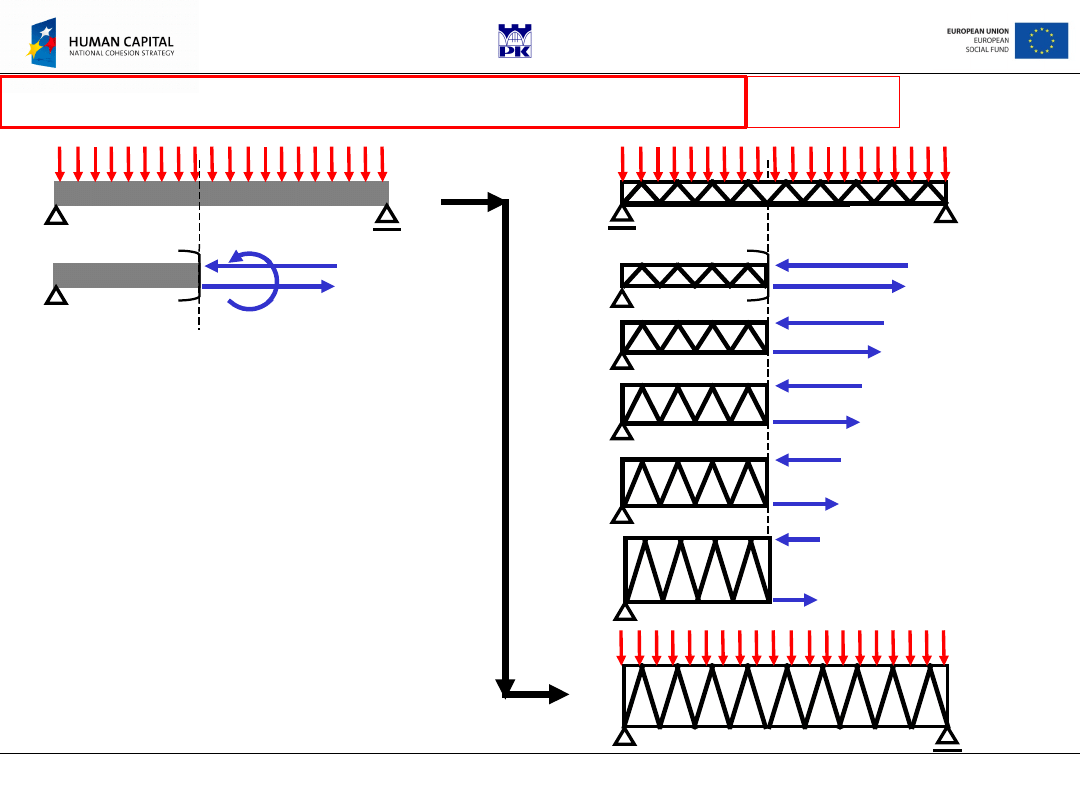
Under the assumptions:
if the structure consists of straight bars

connected and loaded

at hinges

its elements have to bear the normal cross-sectional forces only

Stru
t

The structure has to be kinematically
stable!

Tie

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

6/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

Structure has to be kinematically stable, but can be

statically determine or in-determine!

ν = 2w – p –
3

ν <
0

ν =
0

ν >
0

Kinematics

stable

stable

unstabl
e

Statics

determine

In-determine

undefined

w – number of
joints

p – number of
bars

Too many

joints, too few

bars!

Too many

bars, too few

joints

2w = number of
equations

p +3 = number of
unknowns

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

7/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

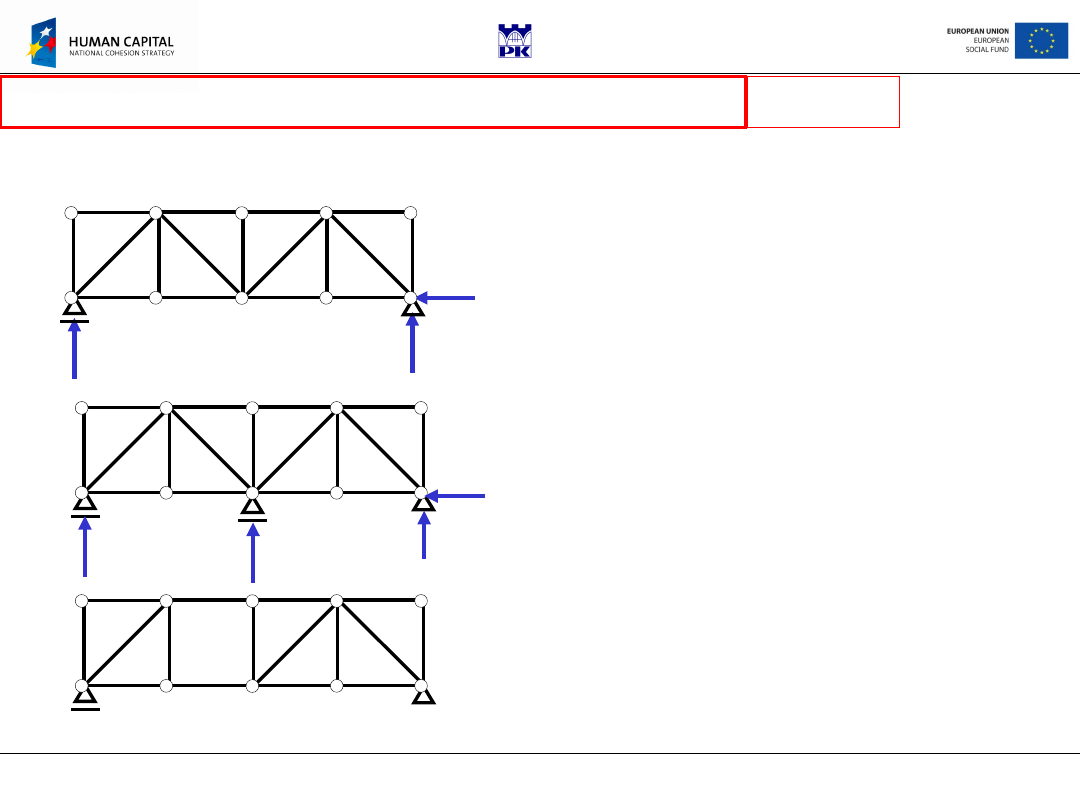
Examples of kinematically and statically
determination

Externally determined

ν = 2·10 – 16 – 3
= 1

ν = 2w – p –
3

Internally
indetermined

w = 10, p
=16

Kionematically
stable

Internally and
externally determined

ν = 2·10 – 17 – 3
= 0

w = 10, p =
17

ν = 2·10 – 17 – 3
= 0

w = 10, p =
17

Internally determined

Externally indetermined

Kinematically
unstable

Kinematically
unstable

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

8/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

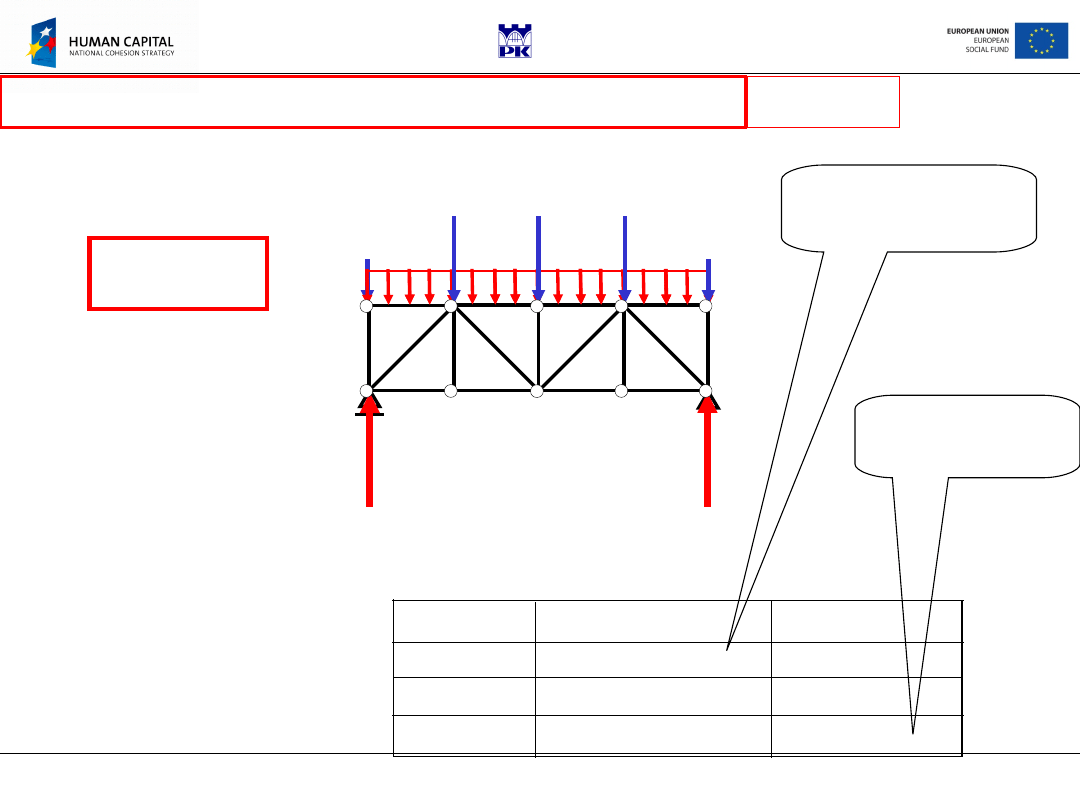
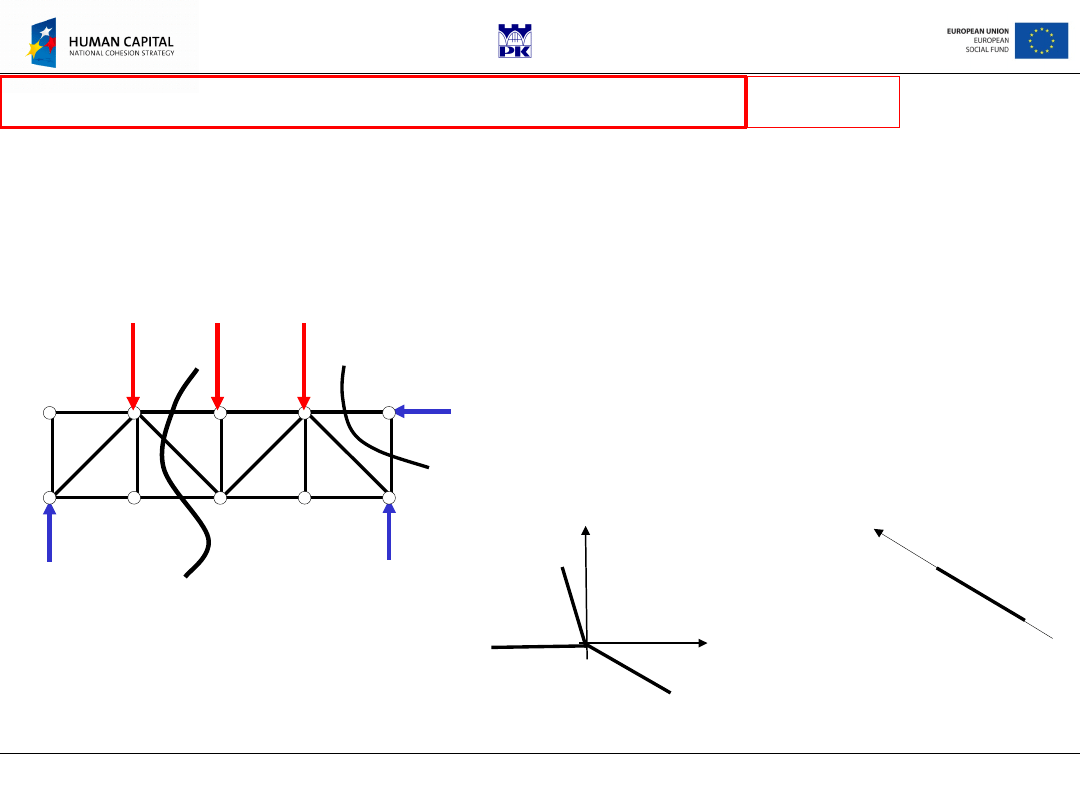
Normal forces in truss elements are to be found from the fundamental
axiom that if the whole structure is in equilibrium then any part of it is
in equilibrium, too.

Such a part of a structure can be obtained by cutting off the truss
through three bars not converging in a point (A), or through two bars
converging in a node. (B). In the former case we have three equations
of equilibrium, in the latter – two.

A node can be cut off; then we have
two equations of equilibrium (C) , or

A

B

X

C

X

Y

D

X = 0
Y = 0
M

K

= 0

X = 0
Y = 0

X = 0

any bar with one equation of
equilibrium (D).

X = 0
Y = 0

B

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

9/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

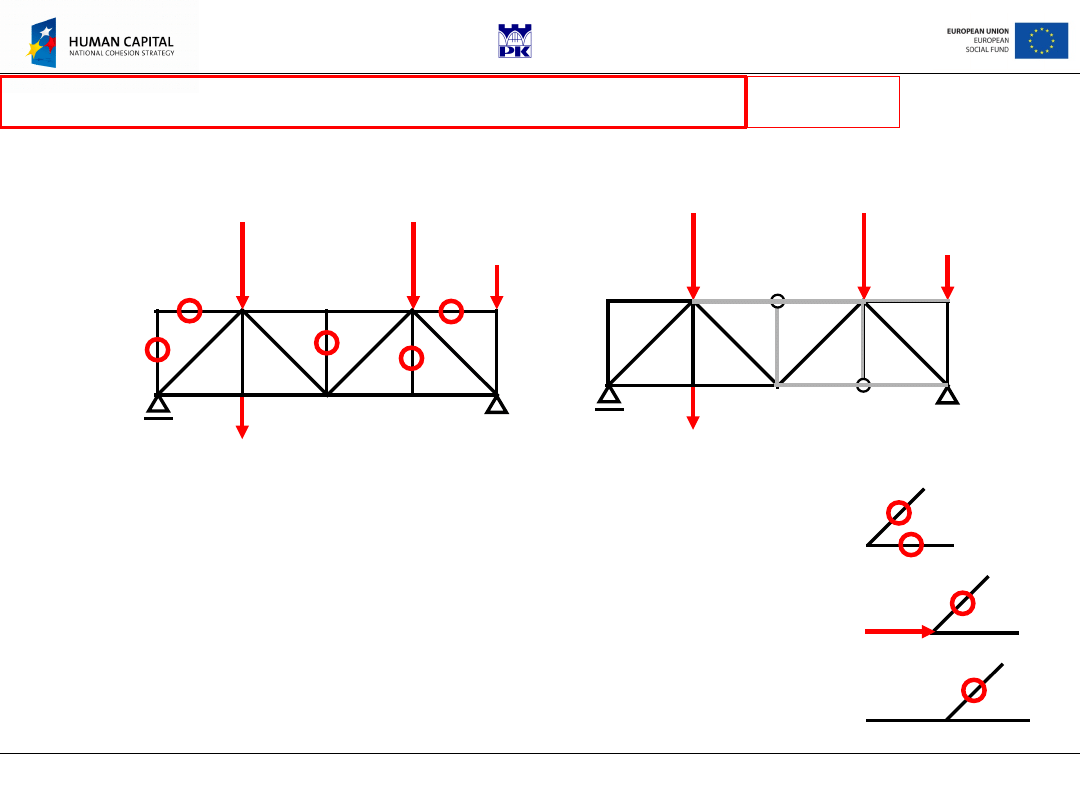
Certain bars are required solely for the purpose of keeping a truss
kinematically stable. The cross-sectional forces in these bars vanish; one
can call them „0-bars”.

There are three cases in which we can easily spot „0-
bars”:

A. When only two bars converge in a node which is
free of loading

C. When unloaded node connects three bars, two of
them being co-linear

B. When a node connects three bars but the loading
acts along of any of these bars

B

A

C

C

A

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

10/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses

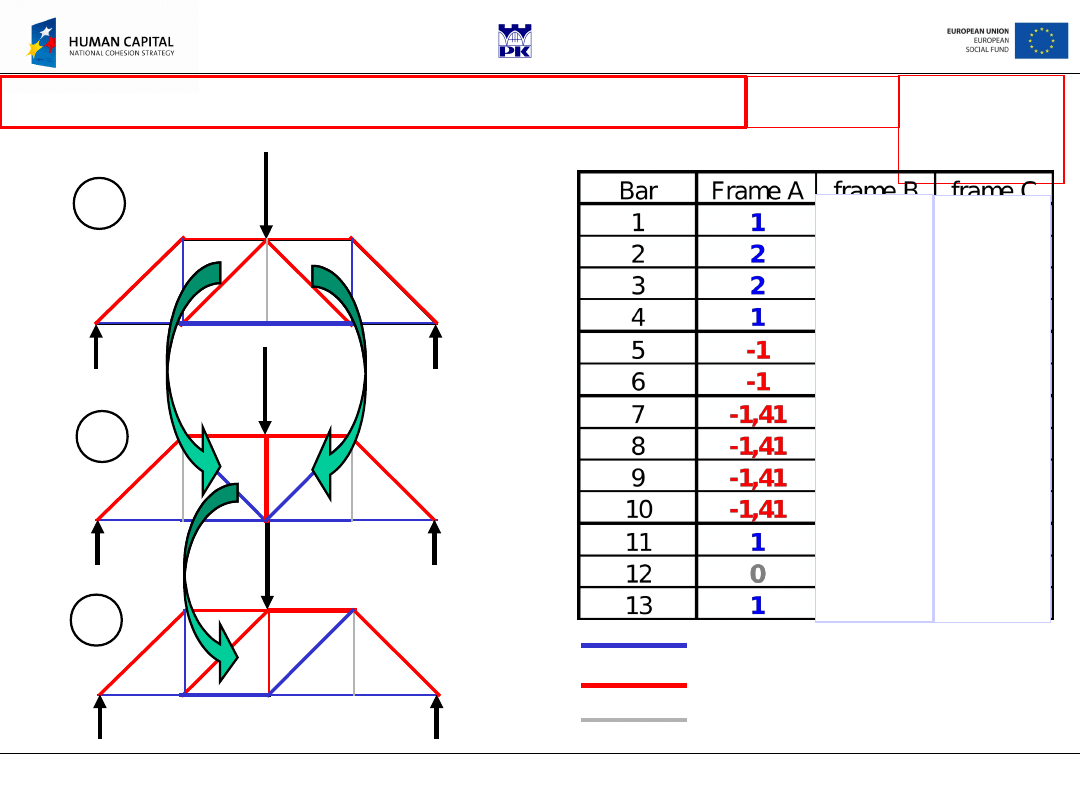
How does

truss

work?

1

2

5

3

4

6

7

8

9

10

11

12

13

A

B

C

Bars under
compression

Bars under
tension

„0-
bars”

P=2

R=1

R=1

All forces in
kN

1

2

5

3

4

6

7

8

9

10

11

12

13

1

2

5

3

4

6

7

8

9

10

11

12

13

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

11/10

M.Chrzanowski: Strength of Materials

SM1-05: Statics 5:Statically determined bar

structures

Trusses



stop