Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

1/6

M.Chrzanowski: Strength of Materials

SM1-08: Continuum Mechanics: Stress

distribution

CONTINUUM MECHANICS

(STRESS DISTRIBUTION)

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

2/6

M.Chrzanowski: Strength of Materials

SM1-08: Continuum Mechanics: Stress

distribution





 

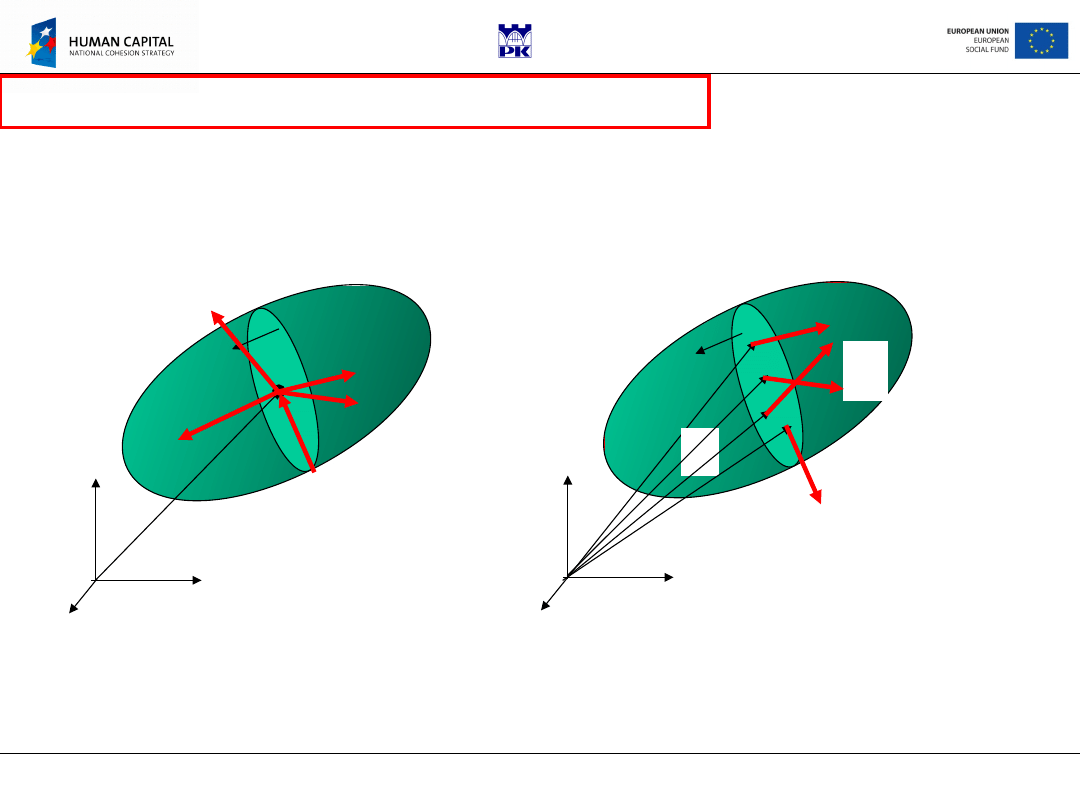
r

p

n

n

r

p

p

const





















;





 

n

p

n

r

r

p

p

const





















;

State of stress

Stress distribution

Stress vector

const

n

r

p



const

r

n



n



r

 

n

r

p

p









,



Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

3/6

M.Chrzanowski: Strength of Materials

SM1-08: Continuum Mechanics: Stress

distribution

x

2

x

1

x

3





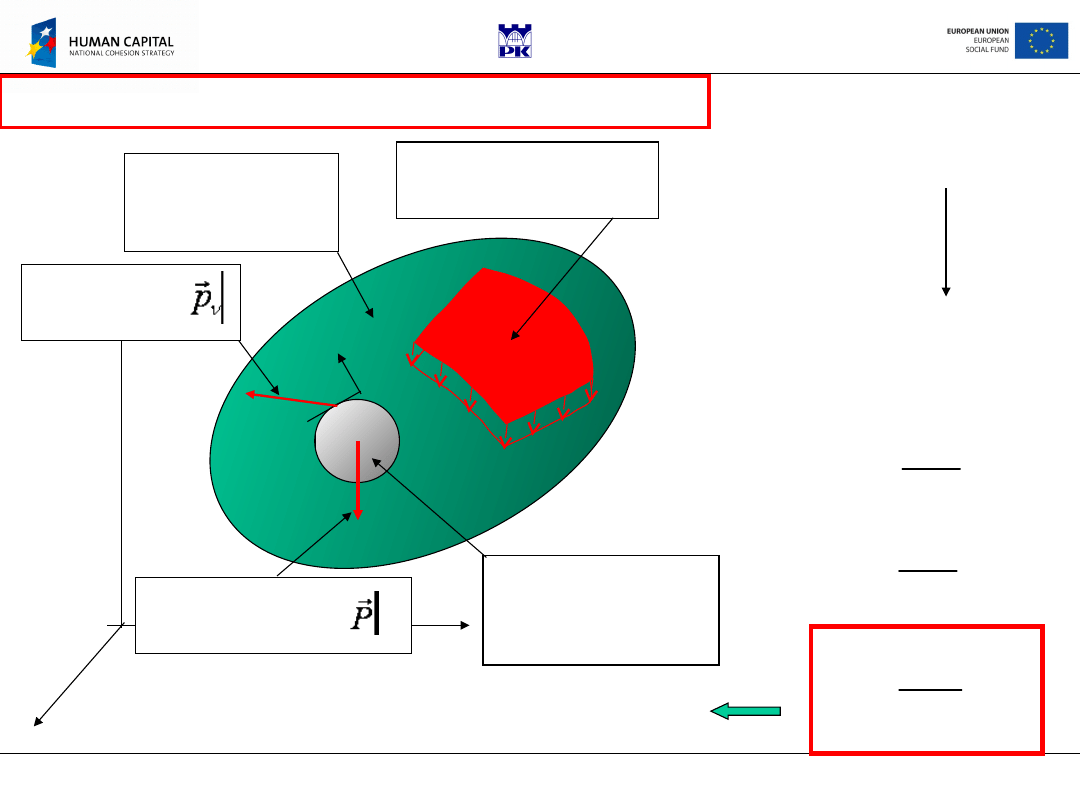
Volume

V

Surface

S

 

i

p









 

i

q

q







Volume

V

0

Surface

S

0

Stress
vector

Volumetric
force

 

i

P

P



dS

p

dV

P

S

V













0

0

0











0

0

0









dS

dV

P

j

S

ij

V

i







0

0

0









dS

dV

P

S

i

V

i





0

0

0













dV

x

dV

P

V

j

ij

V

i



0

0

























dV

x

P

V

j

ij

i



j

ij

i













0























j

ij

i

x

P







,

,

,

3

2

1

x

x

x

ij

ij







GGO
theorem

Surface traction
(loading)



q

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

4/6

M.Chrzanowski: Strength of Materials

SM1-08: Continuum Mechanics: Stress

distribution

0























j

ij

i

x

P







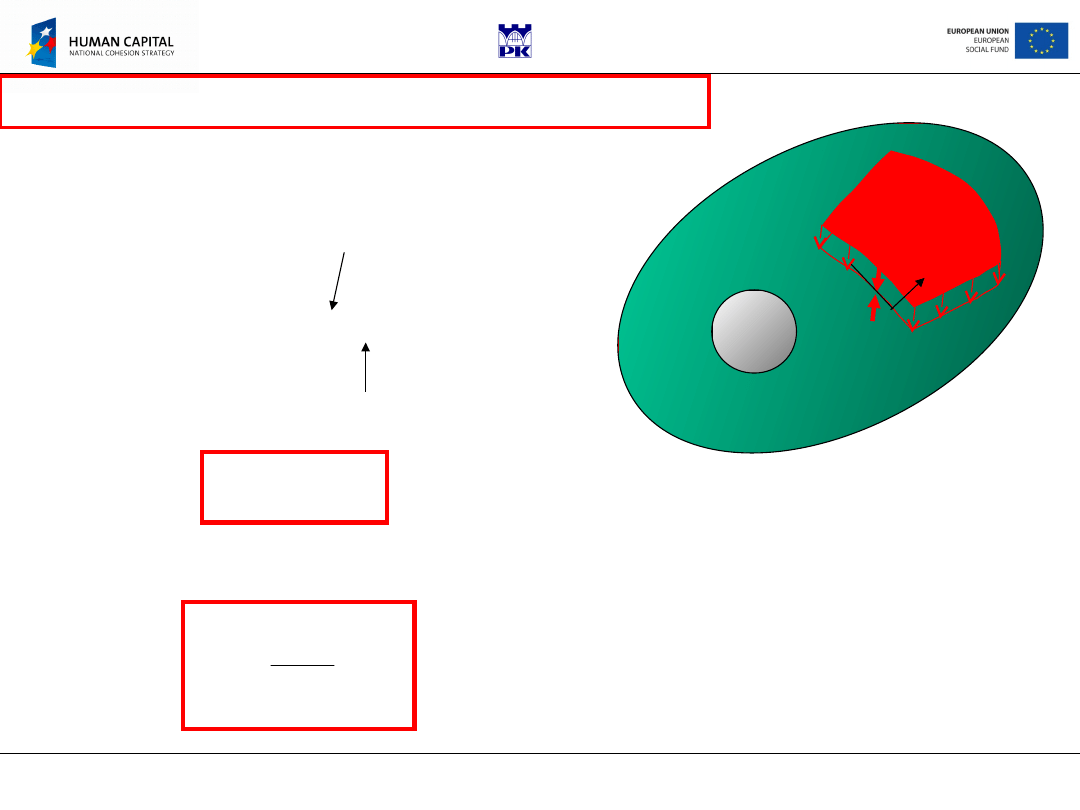
On the body surface stress vector
has to be balanced by the traction
vector



q



p



j

ij

i

i

q

















Stress on the body surface

Coordinates of vector normal to the
surface

j

ij

i

q











This equation states

statics boundary conditions

to comply with the

solution of the equation:

This equation (

Navier equation

)

reflects

internal equilibrium

and has

to be fulfilled in any point of the
body (structure).

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

5/6

M.Chrzanowski: Strength of Materials

SM1-08: Continuum Mechanics: Stress

distribution

0























j

ij

i

x

P



We have to deal with the set of 3 linear
partial differential equations.

Navier equation

in coordintes
reads:

0

0

0

3

33

2

32

1

31

3

3

23

2

22

1

21

2

3

13

2

12

1

11

1





























































x

x

x

P

x

x

x

P

x

x

x

P



















There are 6 unknown functions which
have to fulfil static boundary conditions
(SBC):

j

ij

i

q











We need more equations to determine all 6 functions of stress
distribution. To attain it we have to consider deformation of the
body.

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

6/6

M.Chrzanowski: Strength of Materials

SM1-08: Continuum Mechanics: Stress

distribution

Comments

1. Equation is derived from one of two

equilibrium equations, i.e. that the sum of forces acting over
the body has to vanish.

0























j

ij

i

x

P



2. The other equilibrium equation – sum of the moments equals

zero – yield already assumed symmetry of stress matrix,

σ

ij

=

σ

ji

3. Navier equation is the special case of the motion equation i.e.

uniform motion (no inertia forces involved). The inertia effects
can be included by adding d’Alambert forces to the right hand
side of Navier equation.

Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union

within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education

7/6

M.Chrzanowski: Strength of Materials

SM1-08: Continuum Mechanics: Stress

distribution



stop