Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
1/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
CONTINUUM MECHANICS
(STATE OF DEFORMATION)
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
2/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
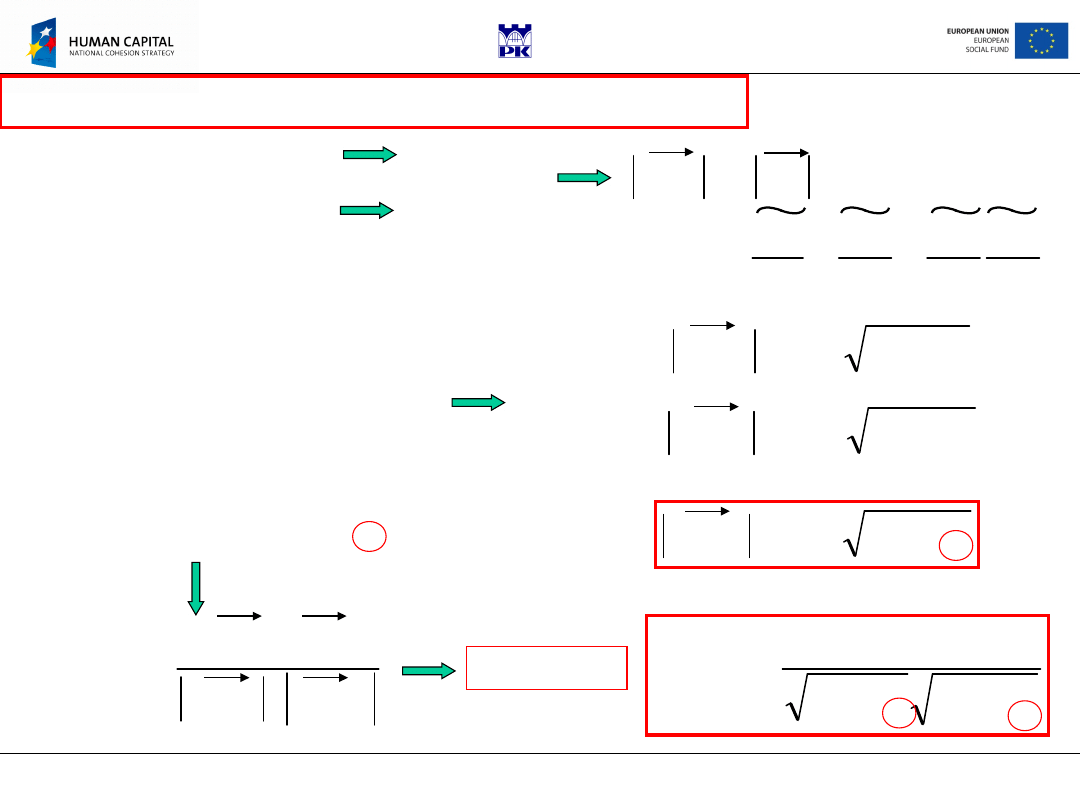
Under action of external loadings the body (structure) changes its
shape. The original positions of material points are shifted to a
new position – this isthe state of deformation.
This change in material point position influences interaction
between body material points resulting in raising internal forces.
If
the whole structure is in equilibrium then any part of it is in
equilibrium, too. This gives rise to the introduction of Navier
equation as shown in preceding chapters
.
This equation cannot be solved without further consideration of
the state of the body being deformed.
Deformation versus internal
forces
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
3/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
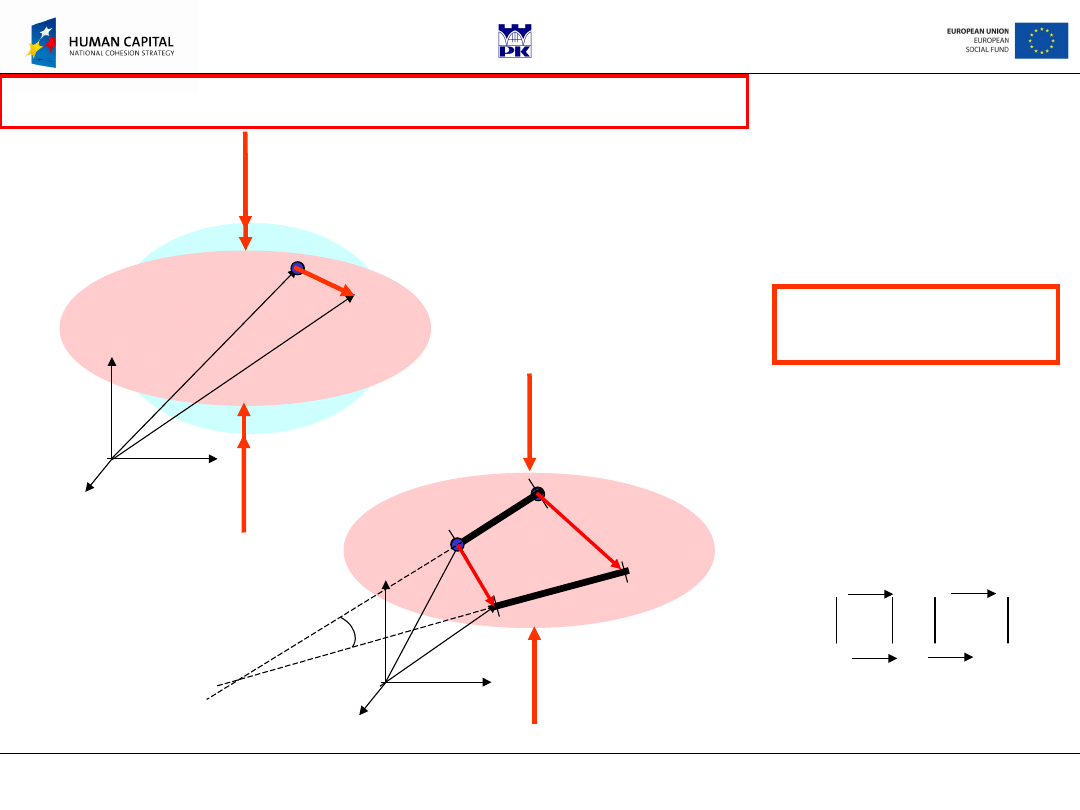
A
A’
'
r
u
r
'
'B
A
AB
Displacement
vector
A
A’
'
r
u
r
B
B’
r
r
u
'
0
'
'
,
B
A
AB
i
u
u
A
u
B
u
3
2
1
,
,
x
x
x
u
u
i
i
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
4/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
'
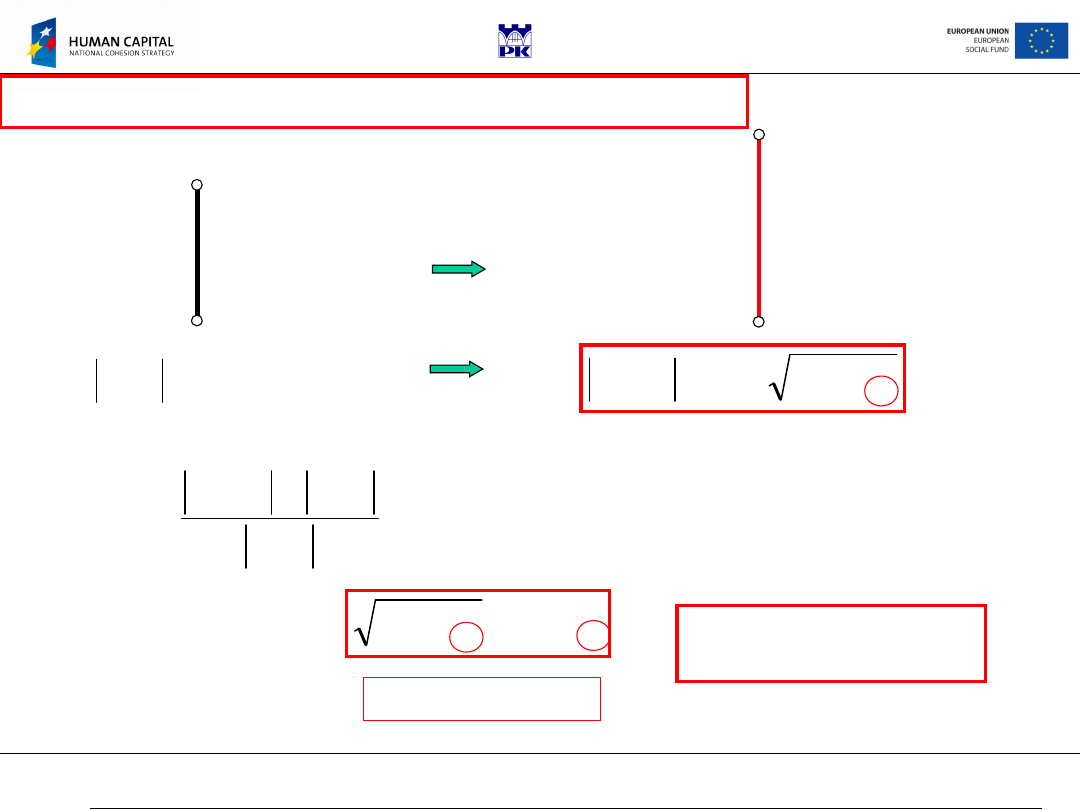
'B
A
AB
j
i
ij
x
x
e
AB
B
A
~
2
...
'
'
2
2
i
x
A
i
i
x
x
B
j
k
i
k
i
j
j
i
ij
x
u
x
u
x
u
x
u
e
~
2
denotes derivative
in an intermediate
point
A’
A
B
2
B
3
23
12
31
B
1
1
x
3
x
2
x
B’
1
B’
2
B’
3
x
1
x
3
x
2
j
i
i
x
u
x
A
'
j
j
i
i
i
x
x
u
x
x
B
'
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
5/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
j
i
ij
x
x
e
AB
B
A
~
2
...
'
'
2
2
ik
k
i
k
x
x
B
j
k
i
k
i
j
j
i
ij
x
u
x
u
x
u
x
u
e
~
2
'
A
k
B'
3
,
2
,
1
i
3
2
1
1
1
,
,
x
x
x
x
B
3
2
2
1
2
,
,
x
x
x
x
B
3
3
2
1
3
,
,
x
x
x
x
B
11
1
1
~
2
1
'
'
e
x
B
A
kk
k
k
e
x
B
A
~
2
1
'
'
22
2
2
~
2
1
'
'
e
x
B
A
i
x
A
………………………..
j
i
j
i
ij
B
A
B
A
B
A
B
A
'
'
'
'
'
'
'
'
cos
jj
ii
ij
ij
e
e
e
~
2
1
~
2
1
~
2
cos
when i
j
no
summation
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
6/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
kk
k
k
e
x
B
A
~
2
1
'
'
k
k
x
AB
1
2
1
~
2
1
lim
'
'
lim
0
0
kk
k
k
kk
k
x
k
k
k
x
e
x
x
e
x
AB
AB
B
A
k
k
kk
kk
e
1
2
1
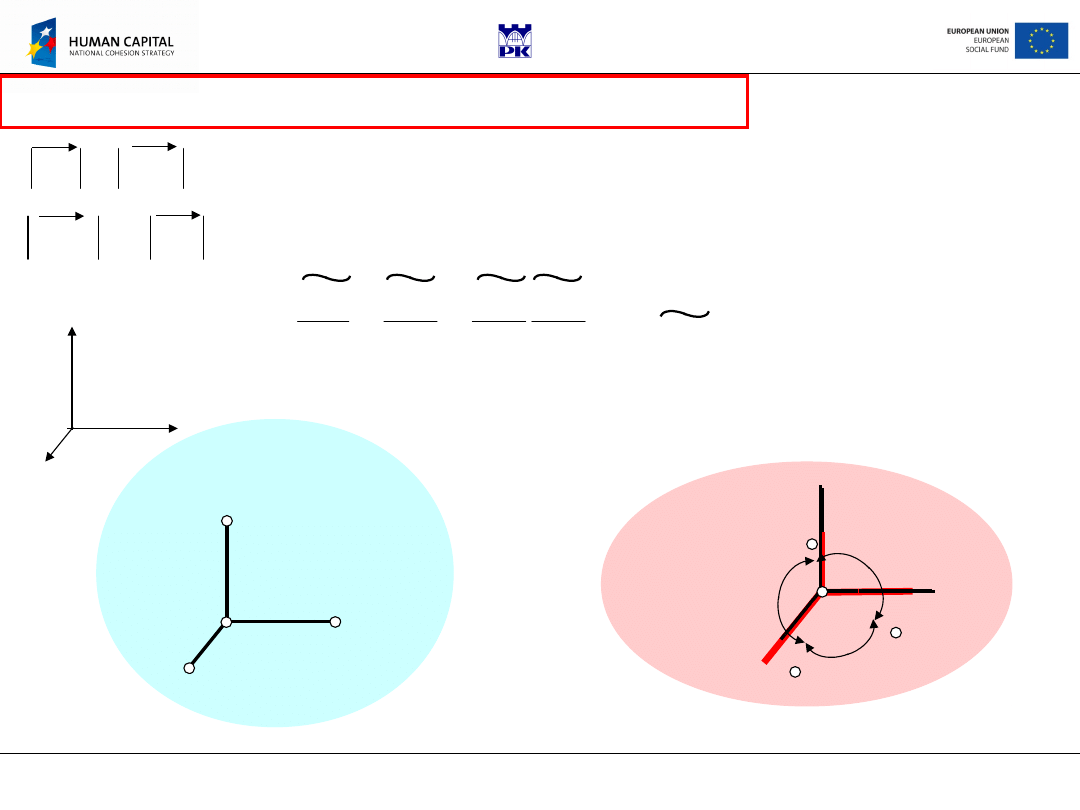
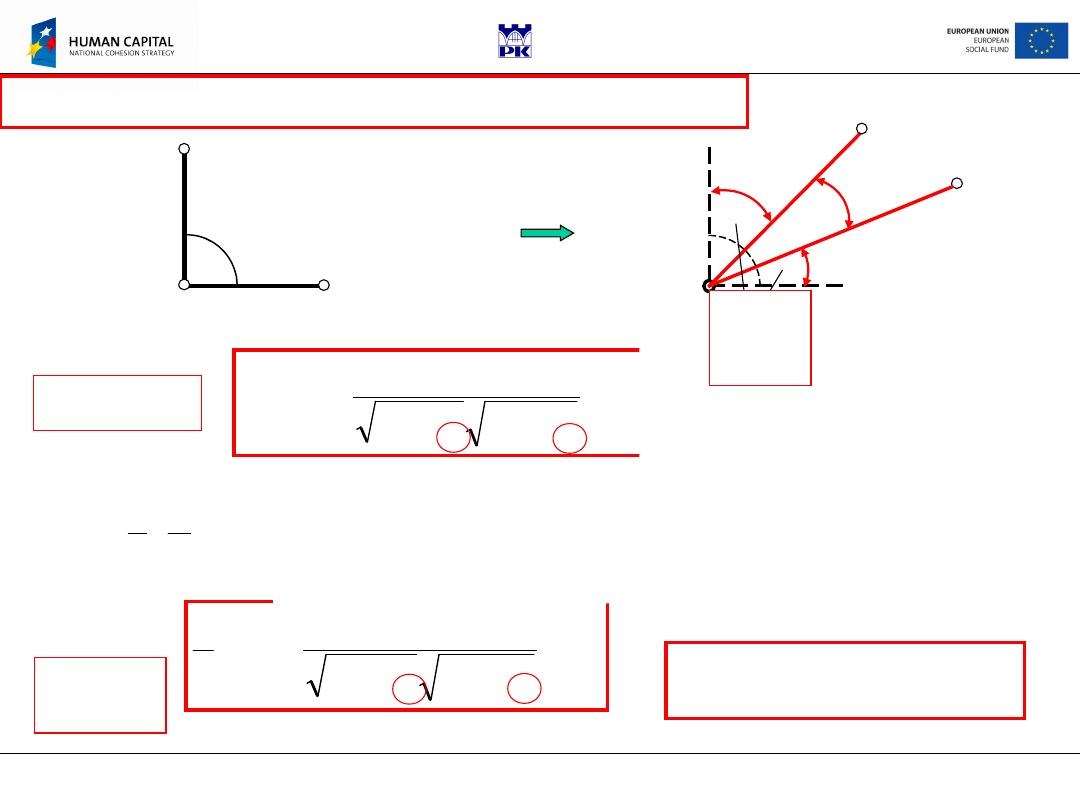
Normal (linear)
strain in point A
A
B
k
A’
B’
k
when i=j=k
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
7/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
ij
jj
ii
ij
ij
e
e
e
2
sin
~
2
1
~
2
1
~
2
cos
when i
j
jj
ii
ij
jj
ii
ij
A
B
A
B
ij
A
B
A
B
e
e
e
e
e
e
j
i
j
i
2
1
2
1
2
arcsin
2
1
~
2
1
~
2
1
~
2
arcsin
2
1
lim
2
2
1
lim
A’
B’
i
B’
j
A
B
i
B
j
/2
ij
ij
jj
ii
ij
e
e
e
2
1
2
1
2
arcsin
2
1
Shear (angular)
strain
when
i
j
/2-
ij
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
8/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
when
i
j
when
i=j=k
ij
1
2
1
kk
e
jj
ii
ij
e
e
e
2
1
2
1
2
arcsin
2
1
1
2
i
j
j
i
ij
x
u
x
u
e
1
j
i
x
u
j
k
i
k
i
j
j
i
ij
x
u
x
u
x
u
x
u
e
2
ij
ij
e
e
2
2
sin
1
2
1
kk
kk
e
1
2
2
1
2
kk
kk
kk
e
2
1
2
1
kk
kk
e
kk
kk
e
ij
ij
ij
e
e
2
2
1
for
i
j
for
i=j=k
i
j
j
i
ij
x
u
x
u
2
1
Cauchy
equation
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
9/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
i
j
j
i
ij
x
u
x
u
2
1
Small
strains
Shear strains
when
i
j
Normal
strains when
i=j
32
2
3
3
2
23
2
1
x
u
x
u
2
2
22
x
u
3
3
33
x
u
1
1
1
1
1
1
11
2
1
x
u
x
u
x
u
21
1
2
2
1
12
2
1
x
u
x
u
31
1
3
3
1
13
2
1
x
u
x
u
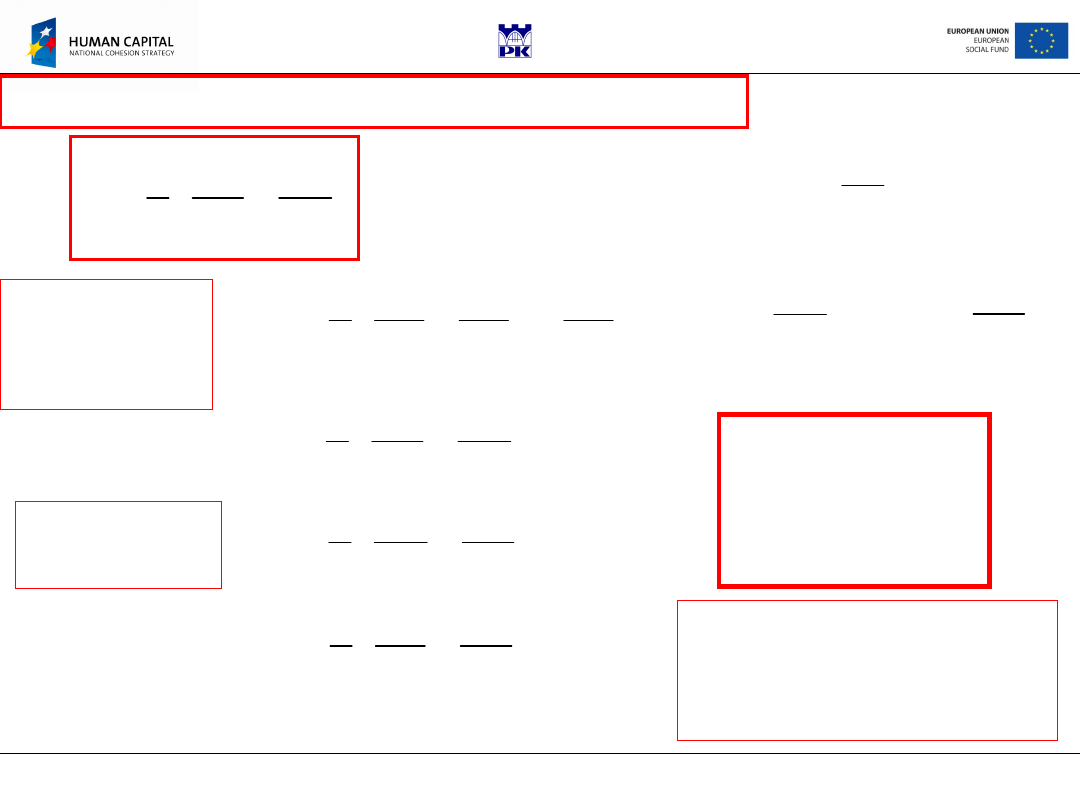
33
32
31
23
22
21
13
12
11
T
Strain matrix
– symmetrical by
definition of angular
strains
1
j
i
x
u
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
10/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
x
1
x
2
x
3
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
11/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
33
32
31
23
22
21
13
12
11
T
Eigenvalues of strain matrix are normal strains
on the planes where there are no shear strains.
Principal strains can be found by solving the
secular equation:
3
2
1
0
0
0
0
0
0
T
0
3
2
2
1
3
I
I
I
where
I
1
, I
2
, I
3
are invariants of strain
matrix
When transfer of strain matrix is made to the
new co-ordinate system then matrix
transformation rule holds:
kl
jl
ik
ij
,
In the co-ordinates
defined by principal
directions of strain
matrix it takes the
diagonal form.
3
2
1
- principal
strains
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
12/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
i
j
j
i
ij
x
u
x
u
2
1
Cauchy equation can be viewed as a the set of 6 linear differential
equations for 3 unknown displacement functions:
3
2
1
,
,
x
x
x
u
i
To solve this set, appropriate kinematic boundary conditions (KBC) for
these functions and/or their derivatives given on the body surface
S
u
have to be formulated
...
u
S
i
u
...
u
S
j
i
x
u
To satisfy the compatibility of deformations only 3 of these 6 six
equations are independent, so the 3 have to be eliminated
If displacement functions are given (or known in advance) then the
Cauchy equation becomes a recipe for determination of all 6
components of the strain matrix.
and/o
r
Comment
s
Project “The development of the didactic potential of Cracow University of Technology in the range of modern construction” is co-financed by the European Union
within the confines of the European Social Fund and realized under surveillance of Ministry of Science and Higher Education
13/12
M.Chrzanowski: Strength of Materials
SM1-09: Continuum Mechanics: State of
deformation
stop