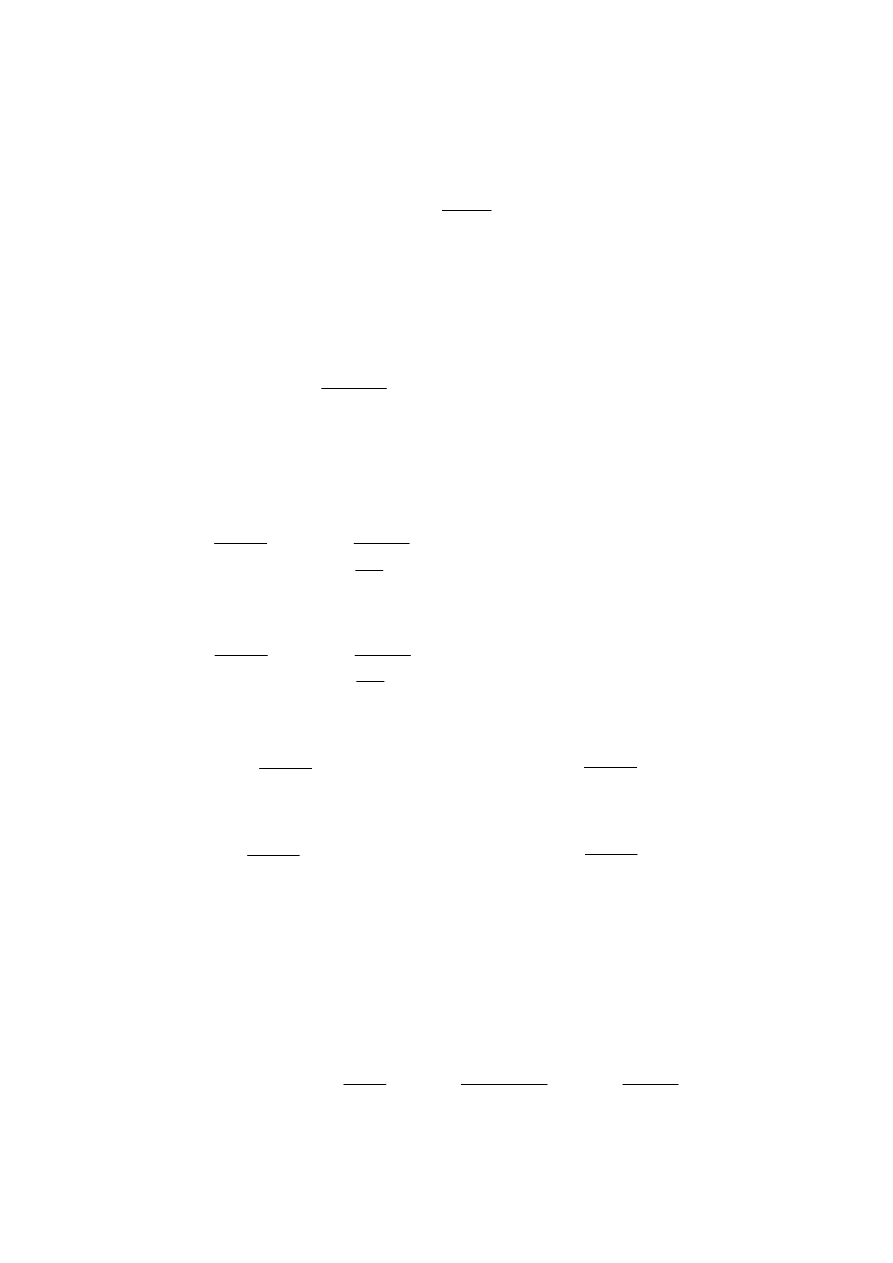
PRZEBIEG ZMIENNOŚCI FUNKCJI
Przykład 1.
f(x)=
2
1
3
x
x
−
1. Dziedzina i miejsca zerowe
Dziedzina :
1-x
2
≠0
x
≠1 ∨
x
≠-1
⇒
Miejsca zerowe:
2
1
3
x
x
−
=0
x=0.
⇒
2. Granice i asymptoty
+∞
=
−
∞
−
→
=
−
∞
−
→
1
2
1
lim
2
1
3
lim
x
x
x
x
x
x
,
−∞
=
−
∞
+
→
=
−
∞
+
→
1
2
1
lim
2
1
3
lim
x
x
x
x
x
x
,
+∞
=
−
−
−
→
2
1
3
1
lim
x
x
x
,
−∞
=
−
+
−
→
2
1
3
1
lim
x
x
x
,
+∞
=
−
−
→
2
1
3
1
lim
x
x
x
,
−∞
=
−
+
→
2
1
3
1
lim
x
x
x
.
Asymptoty poziome:
brak,
Asymptoty pionowe:
x=-1, x=1,
Asymptota ukośna:
a=
1
2
1
2
lim
2
1
3
lim
)
(
lim
−
=
−
∞
→
=
−
∞
→
=
∞
→
x
x
x
x
x
x
x
x
x
f
x
,
Arkadiusz Lisak
1
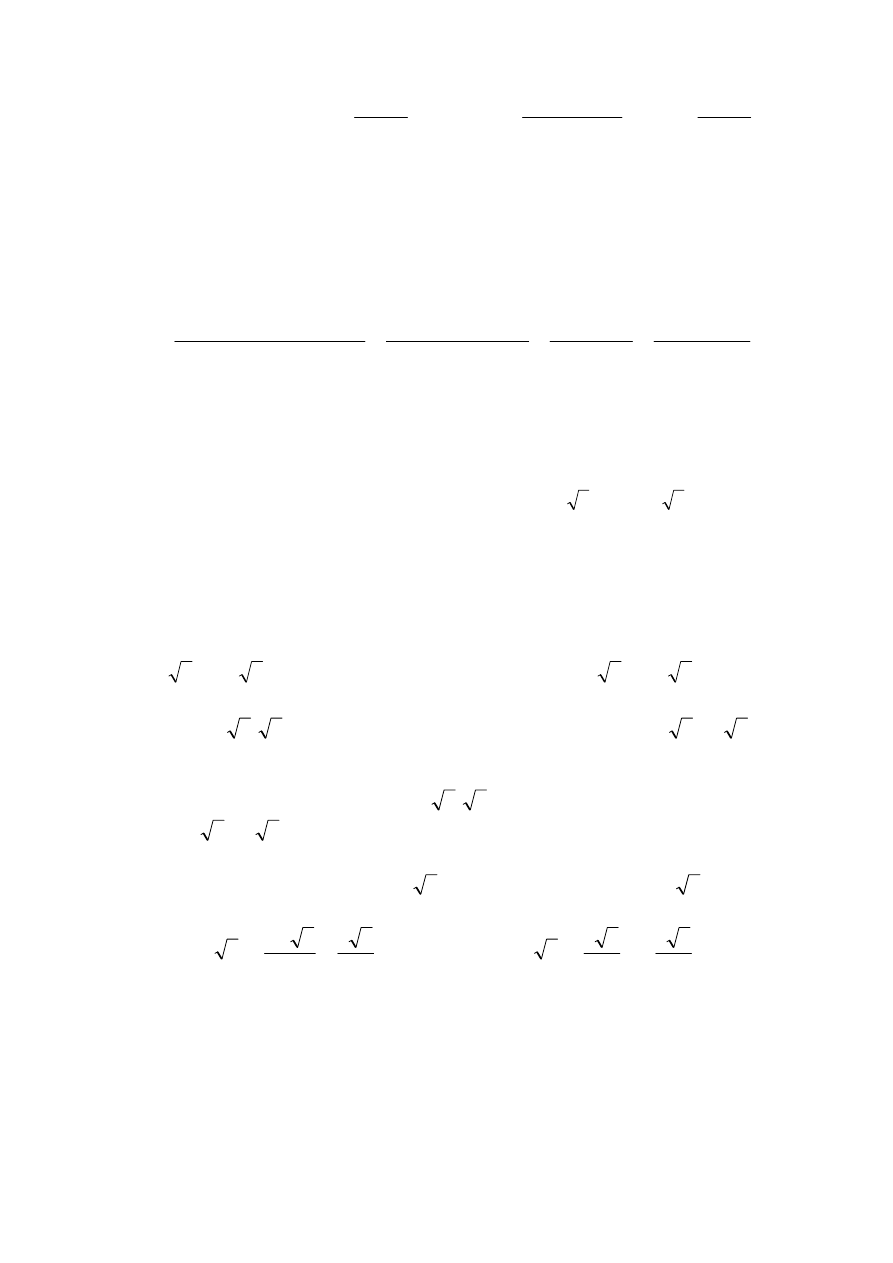
b=
(
)
0
2
1
lim
2
1
3
3
lim
2
1
3
lim
)
(
lim
=
−
∞
→
=
−
−
+
∞
→
=
+
−
∞
→
=
−
∞
→
x
x
x
x
x
x
x
x
x
x
x
x
ax
x
f
x
y=ax+b, więc
y=-x.
3. Pochodna funkcji
(
)
2
2
1
2
3
2
2
2
1
4
2
3
2
2
1
4
2
4
3
2
3
2
2
1
2
3
2
1
2
3
)
(
'
−
−
=
−
−
=
−
+
−
=
−
−
−
−
=
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
f
4. Ekstrema i monotoniczność funkcji
f’(x)=0
⇔ x
2
(3-x
2
)=0
x=0
∨ x=
⇒
3
∨ x= -
3
f’(x)>0
⇔ x
2
(3-x
2
)>0
f’(x)<0
⇔ x
2
(3-x
2
)<0
3-x
2
>0
3-x
2
<0
(
)(
)
x
x
+
−
3
3
>0
(
)(
)
x
x
+
3
−
3
<0
(
)
3
,
3
−
∈
x
(
) (
)
+∞
∪
−
∞
−
∈
,
3
3
,
x
f rośnie w przedziale
(
)
3
,
3
−
, zaś maleje w przedziale
(
) (
)
+∞
∪
−
∞
−
,
3
3
,
.
f osiąga minimum w punkcie
3
−
, zaś maksimum w punkcie 3 .
( )
2
3
3
3
1
3
3
3
min
=
−
−
=
−
f
( )
2
3
3
3
1
3
3
3
max
−
=
−
=
f
.
Arkadiusz Lisak
2
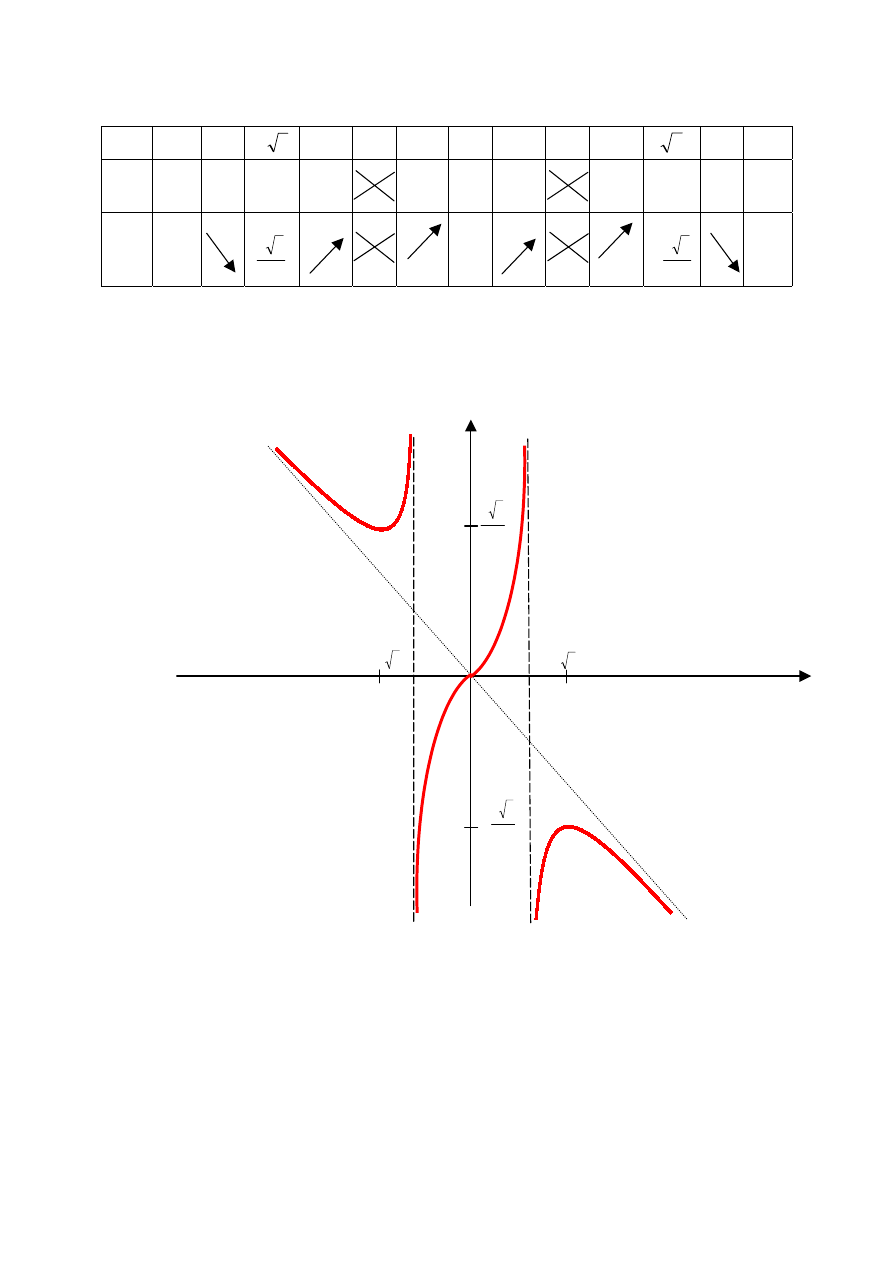
5. Tabelka
-
∞
3
−
-1 0 1
3
+
∞
f’(x)
- 0 +
+ 0 +
+ 0 -
f(x)
+
∞
min
2
3
3
∞
+
∞
−
0
∞
+
∞
−
max
2
3
3
−
-
∞
6. Wykres
2
3
3
3
−
-1
1 3
2
3
3
−
Arkadiusz Lisak
3
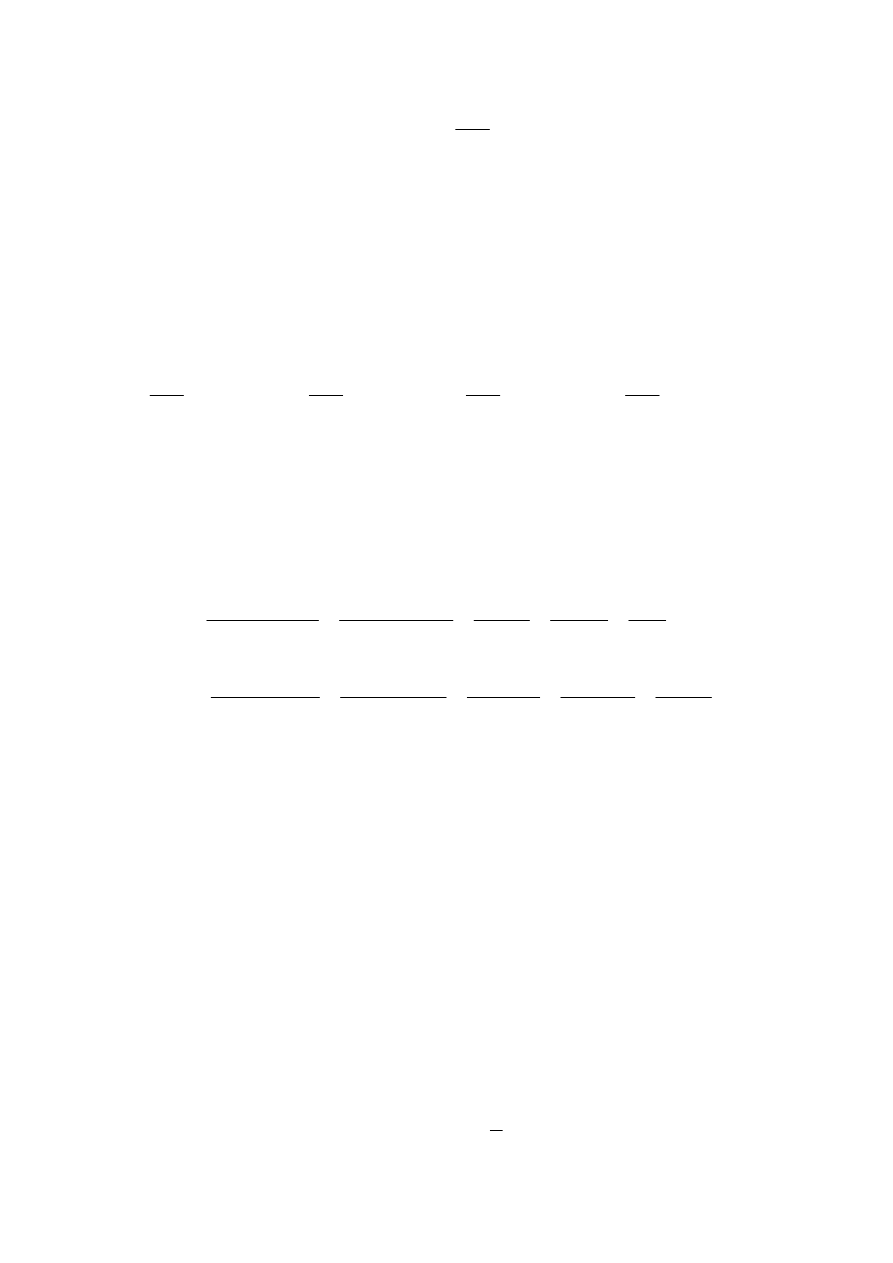
Przykład 2.
( )
2
1
x
x
x
f
−
=
1. Dziedzina i miejsca zerowe
Dziedzina:
0
≠
x
Miejsce zerowe:
1
=
x
2. Granice i asymptoty
0
1
lim
2
=
−
−∞
→
x
x
x
0
1
lim
2
=
−
+∞
→
x
x
x
+∞
=
−
−
→
2
0
1
lim
x
x
x
+∞
=
−
+
→
2
0
1
lim
x
x
x
Asymptota pozioma:
0
=
y
Asymptota pionowa:
0
=
x
3. Pochodne funkcji
( )
(
)
(
)
3
4
4
2
4
2
2
4
2
2
2
2
2
2
1
2
'
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
f
−
=
−
=
−
=
+
−
−
=
−
−
−
=
( )
(
)
(
)
(
)
4
6
2
6
3
2
6
2
3
3
6
2
3
3
2
3
2
2
6
6
3
2
3
''
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
x
f
−
=
−
=
−
=
+
−
=
−
−
=
4. Ekstrema i monotoniczność funkcji
f’(x)=0
⇔ x=2
)
f’(x)>0
⇔ x
3
(x-2)>0
f’(x)<0
⇔ x
3
(x-2)<0
x(x-2)>0
x(x-2)<0
(
) (
+∞
∪
∞
−
∈
,
2
0
,
x
( )
2
,
0
∈
x
f rośnie w przedziale
(
) (
)
+∞
∪
∞
−
,
2
0
,
, zaś maleje w przedziale
( )
2
,
0
.
f osiąga minimum w punkcie 2.
( )
4
1
min
2
−
=
f
Arkadiusz Lisak
4

5. Punkty przegięcia i wypukłość
f’’(x)=0
⇔
x=3
f’’(x)>0
⇔ x<3
f’’(x)<0
⇔
x>3
f jest wypukła w górę (wypukła) w przedziale
, jest wypukła
w dół (wklęsła) w przedziale
(
) {
0
\
3
,
∞
−
}
(
)
+∞
,
3
.
f ma punkt przegięcia dla x=3.
( )
9
2
3
−
=
pp
f
6. Tabelka
-
∞
0
1 2 3 +
∞
f’(x)
+ +
- - - 0 + + + +
f’’(x)
+ +
+ + + + + 0 - -
f(x)
0
∞
+
∞
+
0
min
4
1
−
pp
9
2
−
0
7. Wykres
1 2 3
9
2
−
4
1
−
Arkadiusz Lisak
5
Wyszukiwarka
Podobne podstrony:
przebieg zmienności funkcji
Badanie przebiegu zmienności funkcji
Przebieg zmiennosci funkcji Z Zadanie domowe id 834520
AMI 21.1. Przebieg zmienności funkcji. Zbadać
Przebieg zmiennosci funkcji Z Rozwiazanie zadania domowego id
Elementy przebiegu zmienności funkcji. Twierdzenie de l’Hospitala, Analiza matematyczna
AMI 21 1 Przebieg zmienności funkcji Zbadać
Badanie przebiegu zmiennosci funkcji
Przebieg zmienności funkcji
8 badanie przebiegu zmienności funkcji
Badanie przebiegu zmienności funkcji
Badanie przebiegu zmienności funkcji obejmuje trzy etapy, Badanie przebiegu zmienności funkcji obejm
Badanie przebiegu zmienności funkcji
AMI 21 1 Przebieg zmienności funkcji Zbadać
AMI 21 Przebieg zmienności funkcji
AMI 21 Przebieg zmiennosci fun Nieznany (2)
elementy przebiegu zmienności f , wyklad
więcej podobnych podstron