Wykład 6

Ruch płaski ciała sztywnego

Określenie ruchu płaskiego ciała sztywnego

Ruch płaski ciała sztywnego ruch w którym wszystkie punkty ciała poruszają się w płaszczyznach równoległych

do pewnej płaszczyzny, zwanej płaszczyzną ruchu płaskiego

B B₁

B^'

*

Rys.44 Zmiana położenia pręta AB

A A₁

C środek chwilowego obrotu

Dowolne przemieszczenie figury płaskiej w jej płaszczyźnie

może być dokonane za pomocą przesunięcia równoległego,

równego przesunięciu dowolnie obranego punktu A tej figury, oraz obrotu wokół tego punktu.

Pierwsze twierdzenie Eulera:

Dowolne przemieszczenie figury płaskiej w jej płaszczyźnie

może być dokonane za pomocą obrotu wokół pewnego punktu, zwanego środkiem obrotu

Ruch płaski składa się z chwilowego ruchu postępowego

i chwilowego ruchu obrotowego.

Opis położenia dowolnego punktu ciała sztywnego poruszającego się ruchem płaskim

Y y

Y_B B

* x

r_B r *

Y_A

A

0 X_A X_B X

r_A ω

Z z ε

Rys.45

Ruch dowolnego punktu B figury płaskiej w układzie nieruchomym opisujemy za pomocą promienia wektora r_B

r_B = r_A + r

Prędkość punktu B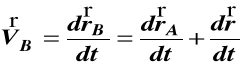
(a)

Ponieważ wektor r nie zmienia długości, zmienia tylko kierunek obracając się z prędkością kątową
i

przyśpieszeniem kątowym
, stąd

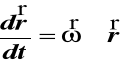
podstawiając to do (a) otrzymujemy

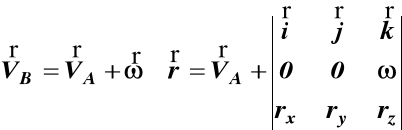
(b)

gdzie r_x = X_B - X_A, r_y = Y_B - Y_A, r_z = Z_B - Z_A

stąd składowe prędkości w układzie nieruchomym są:

,

Przyśpieszenie punktu B

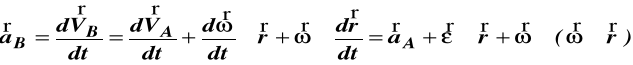
Układ unoszenia xyz z rys.45

y

z

ω y 90⁰

r

x

Rys.46 x

Ostatecznie uwzględniając dane z rys.46 mamy

(62)

Metoda wyznaczania prędkości w ruchu płaskim

Metoda analityczna

Y y B

Y_B *

y

Y_D D r E x

x C *

Y_A A

0 X_A X_B X_C X Rys.47

(c)

gdzie x i y są stałymi, * zmienna w czasie

Składowe prędkości punktu B w układzie 0XYZ

Różniczkując (c) względem czasu

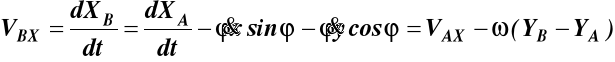
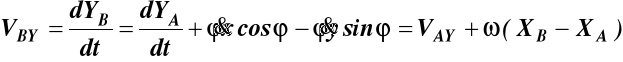
(63)

Przykład 15

Na rys.49 przedstawiony jest schematycznie mechanizm korbowy silnika samochodu, składający się: z wału korbowego OA, który obraca się wokół osi 0, korbowodu AB, oraz tłoka B. Końce korbowodu połączone są przegubowo z tłokiem i wałem. Należy wyznaczyć prędkość tłoka jeśli wał obraca się ze stałą prędkością ω.

Długość wykorbienia równa jest r, a długość korbowodu l.

Y A y

r α l = x

h * B

X

0 X_B

x

Rys.49 α = ωt

Rozwiązanie

;

;

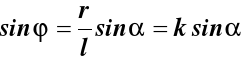
dla
,

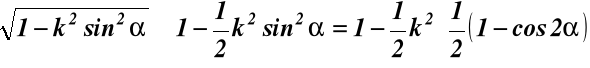
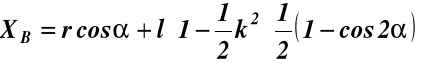
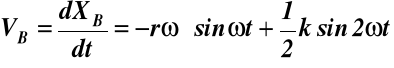
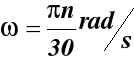
niech: n = 3600obr/min, k = 0.1, r = 2.5cm,

wtedy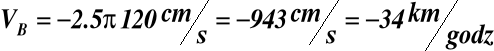

Metoda chwilowego środka obrotu

β

B V_B

V_A α

A ω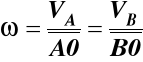
(64)

0

Rys.50 0 środek chwilowego obrotu ciała AB

Przykład 16

Dla konstrukcji i danych z przykładu 15 określić prędkość tłoka metodą środka chwilowego obrotu.

C

ω₁

V_A = ωr

90⁰

A l 90⁰

ω r α β

0 B

V_B Rys.51

z Δ 0AB
;

z Δ 0CB
;
;

;
;

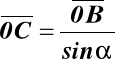
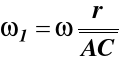
Po kolejnych podstawieniach i przekształceniach

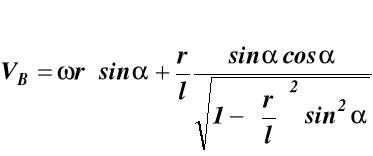
Metoda superpozycji V_AB

90⁰

A ω V_B

α α B V_B = V_A + V_AB (65)

V_A V_A

Rys.52 Wyznaczanie prędkości punktu B metodą

superpozycji
(66)

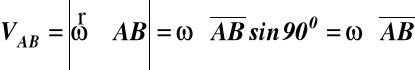
(d)

Przykład 17

Wagon porusza się z prędkością V_w = 72km/h = 20m/s.

określić prędkości punktów A i B (rys.53). R = 35cm,

r = 32cm. A V_A = ω(R+r)

R r

ω V_w = 20m/s, ω = V_w/r = 20/32

szyna Rys.53

V_B B V_B = ω(R-r)

Przyśpieszenia w ruchu płaskim

Metoda analityczna

Różniczkując (63) (rys.47)

(63)

otrzymujemy składowe przyśpieszenia

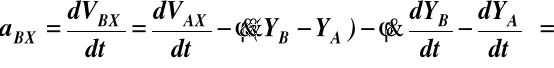
(67a)

=

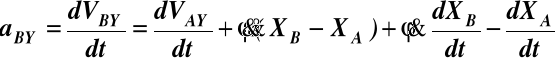
(67b)

Metoda superpozycji

a_BA a_Bt

a_B

ε ω A

α a_A a_BAn B a_A

Rys.53 Wyznaczanie przyśpieszenia metodą superpozycji

a_B = a_A + a_BA a_BA = a_BAn + a_BAt (68)

a_BAn = ω²l; a_BAt = εl; gdzie l = AB

(69)

39kin

40kin

41kin

42kin

43kin

44kin

45kin

---