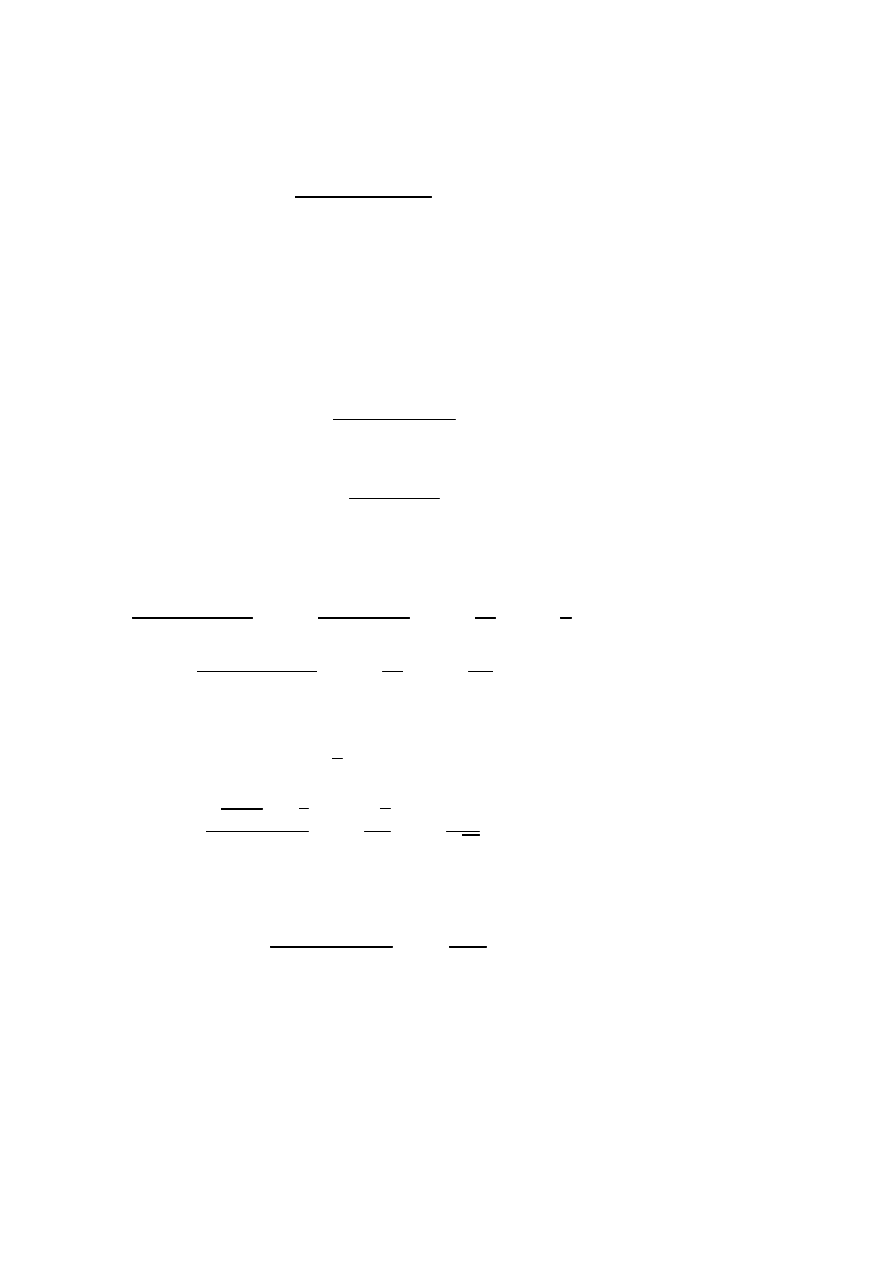
P OCH ODN A FUN KCJI
D E FIN ICJA . Za l´o ˙zm y, ˙ze fu n kc ja f je s t o kr e ´s lo n a w p e wn ym
o t o c z e n iu
p u n kt u x
0
. Je ˙ze li is t n ie je s ko ´n c z o n a g r a n ic a
lim
h→0
f ( x
0
+ h) − f( x
0
)
h
,
t o n a z ywa m y j¸a pochodn¸a funkcji f w punkcie x
0
i o z n a c z a m y f
′
( x
0
) .
D E FIN ICJA . Je ˙ze li fu n kc ja f m a p o c h o d n ¸a w ka ˙zd ym
p u n kc ie p e wn e g o
z b io r u D, t o p r z yp o r z ¸a d ko wa n ie ka ˙zd e m u p u n kt o wi x ∈ D lic z b y
f
′
( x) n a z ywa m y fu n kc j¸a p o c h o d n ¸a . Fu n kc j¸e t ¸e o z n a c z a m y p r z e z f
′
( x) .
M´o wim y t e ˙z, ˙ze f je s t r´o˙zniczkowalna w D.
U W A GA . Za m ia s t p is a ´c
f
′
( x) = lim
h→0
f ( x + h) − f( x)
h
,
m o ˙zn a p is a ´c ( p o d s t a wia j¸a c
z = x + h)
f
′
( x) = lim
z→x
f ( z) − f( x)
z − x
.
P R ZY K L A D . Fu n kc ja f ( x) = |x| n ie m a p o c h o d n e j w p u n kc ie x
0
= 0 .
W ys t a r c z y z a u wa ˙zy´c , ˙ze
lim
h→0
+
f ( 0 + h) − f( 0 )
h
= lim
h→0
+
|0 + h| − |0 |
h
= lim
h→0
+
|h|
h
= lim
h→0
+
h
h
= 1 ,
lim
h→0
−
f ( 0 + h) − f( 0 )
h
= lim
h→0
−
|h|
h
= lim
h→0
−
−h
h
= −1 .
Gr a n ic a le wo s t r o n n a n ie je s t r ´o wn a p r a wo s t r o n n e j, wi¸e c g r a n ic a p r z y
h → 0 n ie is t n ie je .
P R ZY K L A D . Fu n kc ja f ( x) =
3
√
x n ie m a p o c h o d n e j w p u n kc ie x
0
= 0 .
W ys t a r c z y z a u wa ˙zy´c , ˙ze
lim
h→0
3
√
0 + h −
3
√
0
h
= lim
h→0
3
√
h
h
= lim
h→0
1
3
√
h
2
= ∞.
P R ZY K L A D . P o c h o d n a fu n kc ji s t a le j je s t r ´o wn a 0 . N ie c h
f ( x) = c.
W t e d y
f
′
( x) = lim
h→0
f ( x + h) − f( x)
h
= lim
h→0
c − c
h
= 0 .
1

IN TE R P R E TA CJA GE OME TR Y CZN A .
P o c h o d n a
f
′
( x
0
)
je s t r ´o wn a t a n g e n s o wi k¸a t a , ja ki t wo r z y z o s i¸a 0 x
s t yc z n a d o wykr e s u fu n kc ji y = f ( x)
w p u n kc ie
( x
0
, f ( x
0
) ) .
In a c z e j m ´o wi¸a c : is t n ie n ie p o c h o d n e j f
′
( x
0
)
g wa r a n t u je is t n ie n ie
s t yc z n e j ( n ie r ´o wn o le g le j d o o s i 0 y) d o wykr e s u fu n kc ji y = f ( x)
w
p u n kc ie
( x
0
, f ( x
0
) ) . S t yc z n a t a m a r ´o wn a n ie
y − y
0
= f
′
( x
0
) ( x − x
0
) .
IN TE R P R E TA CJA FIZY CZN A .
Je ˙ze li x o z n a c z a c z a s , a f( x) je s t d lu g o ´s c i¸a d r o g i o d p o c z ¸a t ku r u c h u d o
c h wili x, t o
f ( x
0
+ h) − f( x
0
)
je s t d lu g o ´s c i¸a d r o g i p r z e b yt e j w c z a s ie
o d x
0
d o x
0
+ h, ilo r a z r ´o ˙zn ic o wy
f (x
0
+h)−f(x
0
)
h
je s t p r ¸e d ko ´s c i¸a ´s r e d n i¸a
t e g o r u c h u w c z a s ie o d x
0
d o x
0
+ h, a p o c h o d n a f
′
( x
0
) je s t pr¸edko´sci¸a
t e g o r u c h u w c h wili x
0
.
TW IE R D ZE N IE 1 . Fu n kc ja r ´o ˙zn ic z ko wa ln a je s t c i¸a g la .
D ow´
od.
N ie c h x
0
b ¸e d z ie d o wo ln ym
p u n kt e m , w kt ´o r ym
is t n ie je f
′
( x
0
) .
P o ka ˙ze m y, ˙ze f je s t c i¸a g la w t ym
p u n kc ie , t o z n a c z y, ˙ze
lim
x→x
0
f ( x) = f( x
0
) .
lim
x→x
0
f ( x) = lim
x→x
0
f ( x) − f( x
0
)
x − x
0
( x − x
0
) + f ( x
0
)
= lim
x→x
0
f
′
( x
0
) ( x − x
0
) + f ( x
0
) = f ( x
0
) .
TW IE R D ZE N IE 2 . Za l´o ˙zm y, ˙ze fu n kc je f ( x) o r a z g( x) s ¸a r ´o ˙zn ic z ko wa ln e
w p e wn ym
p r z e d z ia le . W t e d y:
1 .
f( x) + g( x)
′
= f
′
( x) + g
′
( x)
2 .
f( x) − g( x)
′
= f
′
( x) − g
′
( x)
3 .
f( x) g( x)
′
= f
′
( x) g( x) + f ( x) g
′
( x)
4 .
cf( x)
′
= cf
′
( x)
5 .
f (x)
g(x)
′
=
f
′
(x)g(x)−f(x)g
′
(x)
g
2
(x)
o ile
g( x) = 0
6 .
f[g( x) ]
′
= f
′
[g( x) ]g
′
( x) .
D ow´
od wzor´
ow 1 i 2.
f( x) ± g( x)
′
= lim
h→0
[f( x + h) ± g( x + h) ] − [f( x) ± g( x) ]
h
= lim
h→0
f ( x + h) − f( x)
h
±
g( x + h) − g( x)
h
= f
′
( x) ± g
′
( x)

D ow´
od wzoru 3.
f( x) g( x)
′
= lim
h→0
f ( x + h) g( x + h) − f( x) g( x)
h
= lim
h→0
f ( x + h) g( x + h) − f( x) g( x + h) + f( x) g( x + h) − f( x) g( x)
h
= lim
h→0
f ( x + h) − f( x)
h
g( x + h) + f ( x)
g( x + h) − g( x)
h
= f
′
( x) g( x) + f ( x) g
′
( x)
D ow´
od wzoru 4.
cf( x)
′
= c
′
f ( x) + cf
′
( x) = 0 + cf
′
( x) = cf
′
( x)
D ow´
od wzoru 5.
f ( x)
g( x)
′
= lim
h→0
f (x+h)
g(x+h)
−
f (x)
g(x)
h
= lim
h→0
1
h
f ( x + h) g( x) − f( x) g( x + h)
g( x + h) g( x)
= lim
h→0
1
h
f ( x + h) g( x) − f( x) g( x) + f( x) g( x) − f( x) g( x + h)
g( x + h) g( x)
= lim
h→0
1
g( x + h) g( x)
f ( x + h) − f( x)
h
g( x) − f( x)
g( x + h) − g( x)
h
=
f
′
( x) g( x) − f( x) g
′
( x)
g
2
( x)
D ow´
od wzoru 6 tylko dla przypadku gdy g
( z) = g( x) dla z nale˙z¸acych
do pewnego s¸asiedztwa punktu x.
f[g( x) ]
′
= lim
z→x
f [g( z) ] − f[g( x) ]
z − x
= lim
z→x
f [g( z) ] − f[g( x) ]
g( z) − g( x)
·
g( z) − g( x)
z − x
= f
′
[g( x) ]g
′
( x) .
TW IE R D ZE N IE 3 . Za l´o ˙zm y, ˙ze fu n kc ja f
−1
je s t fu n kc j¸a o d wr o t n ¸a d o
fu n kc ji c i¸a g le j i m o n o t o n ic z n e j f o r a z ˙ze f m a w p u n kc ie y
0
p o c h o d n ¸a
f
′
( y
0
) = 0 . W t e d y fu n kc ja f
−1
m a w p u n kc ie
x
0
= f ( y
0
)
p o c h o d n ¸a
( f
−1
)
′
( x
0
) =
1
f
′
(y
0
)
.
D ow´
od.
P r z yp o m n ijm y, ˙ze
f
−1
( x) = y ⇔ x = f( y) ,
f
−1
( x
0
) = y
0
⇔ x
0
= f ( y
0
) .
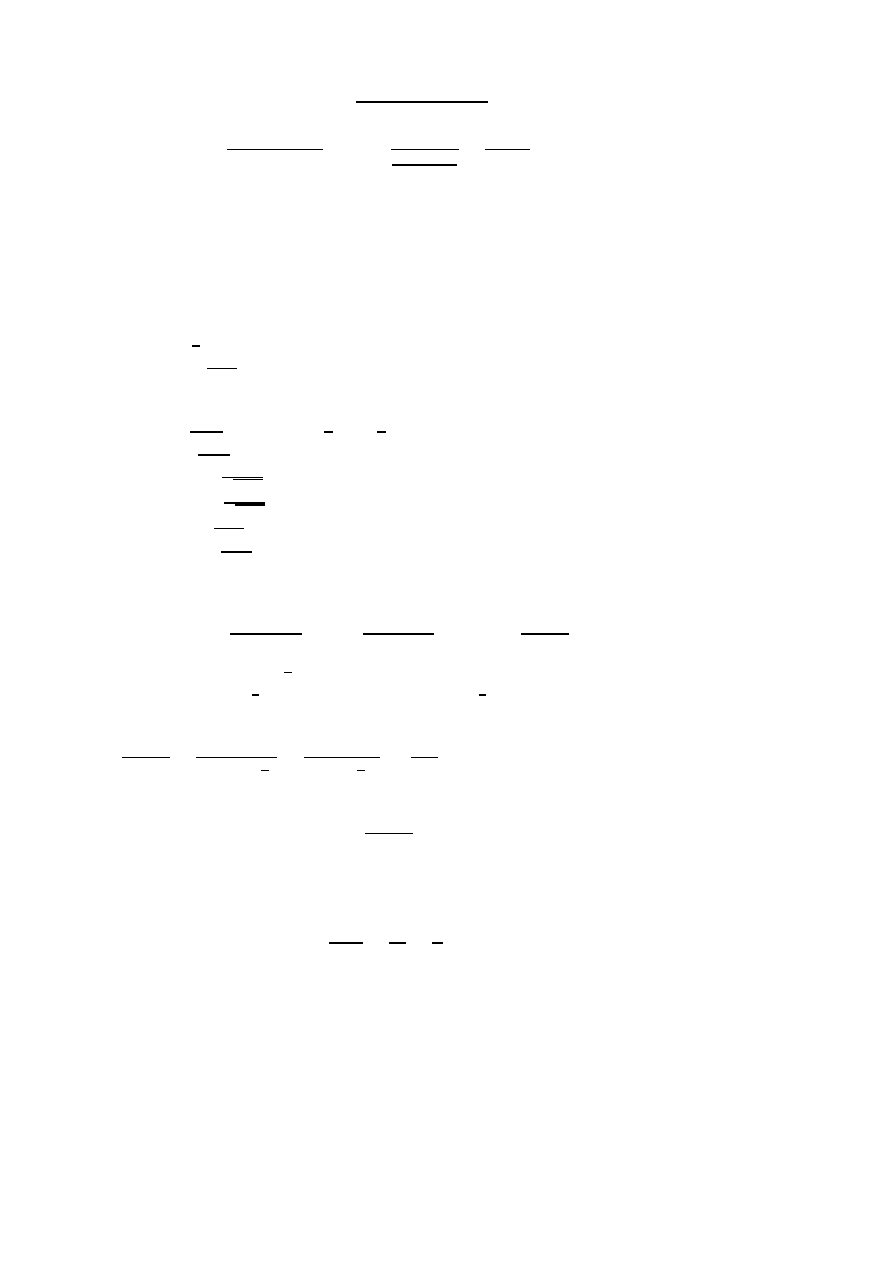
Za t e m
( f
−1
)
′
( x
0
) = lim
x→x
0
f
−1
( x) − f
−1
( x
0
)
x − x
0
= lim
y→y
0
y − y
0
f ( y) − f( y
0
)
= lim
y→y
0
1
f (y)−f(y
0
)
y−y
0
=
1
f
′
( y
0
)
.
P OD S TA W OW E W ZOR Y .
W z o r y t e s ¸a s lu s z n e d la x n a le ˙z ¸a c yc h d o c z ¸e ´s c i ws p ´o ln e j d z ie d z in fu n kc ji
wys t ¸e p u j¸a c yc h p o le we j i p r a we j s t r o n ie wz o r u .
1 . ( x
r
)
′
= rx
r−1
2 . ( e
x
)
′
= e
x
, d la
x ∈ R
3 . ( a
x
)
′
= a
x
ln a, d la
x ∈ R, a > 0
4 . ( ln x)
′
=
1
x
, d la
x > 0
5 . ( lo g
a
x)
′
=
1
x ln a
, d la
x > 0 , a > 0 , a = 1
6 . ( s in x)
′
= c o s x, d la
x ∈ R
7 . ( c o s x)
′
= − s in x, d la x ∈ R
8 . ( t g x)
′
=
1
cos
2
x
, d la x ∈
−
π
2
+kπ,
π
2
+kπ
, g d z ie k = 0 , ±1 , ±2 , . . .
9 . ( c t g x)
′
=
−1
sin
2
x
, d la
x ∈
0 +kπ, π+kπ, g d z ie k = 0 , ±1 , ±2 , . . .
1 0 . ( a r c s in x)
′
=
1
√
1−x
2
, d la
x ∈ ( −1 , 1 )
1 1 . ( a r c c o s x)
′
=
−1
√
1−x
2
, d la
x ∈ ( −1 , 1 )
1 2 . ( a r c t g x)
′
=
1
1+x
2
, d la
x ∈ R
1 3 . ( a r c c t g x)
′
=
−1
1+x
2
, d la
x ∈ R.
D ow´
od wzoru 2.
e
x
′
= lim
h→0
e
x+h
− e
x
h
= lim
h→0
e
x
e
h
− e
x
h
= e
x
lim
h→0
e
h
− 1
h
P o d s t a wia m y e
h
− 1 =
1
z
( o c z ywi´s c ie t u
h = 0 ) . L o g a r yt m u j¸a c
r ´o wn a n ie
e
h
= 1 +
1
z
o t r z ym a m y h = ln ( 1 +
1
z
) . P o n a d t o , g d y
h → 0
+
, t o
z → +∞ o r a z g d y h → 0
−
, t o
z → −∞. Za t e m
e
h
− 1
h
=
1
z ln ( 1 +
1
z
)
=
1
ln ( 1 +
1
z
)
z
→
1
ln e
= 1 , g d y h → 0 .
Os t a t e c z n ie ,
e
x
′
= e
x
lim
h→0
e
h
− 1
h
= e
x
.
D ow´
od wzoru 4.
P r z yp o m n ijm y, ˙ze
y = ln x ⇔ x = e
y
. Za s t o s u je m y
t wie r d z e n ie o p o c h o d n e j fu n kc ji o d wr o t n e j.
( ln x)
′
=
1
( e
y
)
′
=
1
e
y
=
1
x

D ow´
od wzoru 1 tylko dla przypadku, gdy x >
0 . R ´o ˙zn ic z ku j¸a c r ´o wn a n ie
ln x
r
= r ln x o t r z ym a m y
1
x
r
( x
r
)
′
= r
1
x
. S t ¸a d
( x
r
)
′
= rx
−1
x
r
= rx
r−1
.
D ow´
od wzoru 3.
R ´o ˙zn ic z ku j¸a c r ´o wn a n ie
ln a
x
= x ln a o t r z ym a m y
1
a
x
( a
x
)
′
= ln a. S t ¸a d
( a
x
)
′
= a
x
ln a.
D ow´
od wzoru 5.
Z wla s n o ´s c i lo g a r yt m ´o w:
lo g
a
x =
ln x
ln a
, a
wi¸e c
( lo g
a
x)
′
=
(ln x)
′
ln a
=
1
x ln a
.
D ow´
od wzoru 6.
S ko r z ys t a m y z e wz o r ´o w: s in α−s in β = 2 c o s
α+β
2
s in
α−β
2
o r a z
lim
t→0
sin t
t
= 1 .
( s in x)
′
= lim
h→0
s in ( x + h) − s in x
h
= lim
h→0
2 c o s
x+h+x
2
s in
x+h−x
2
h
= lim
h→0
c o s ( x +
h
2
) s in
h
2
h
2
= c o s x.
D ow´
od wzoru 7.
S ko r z ys t a m y z e wz o r u : c o s α−c o s β = −2 s in
α+β
2
s in
α−β
2
.
( c o s x)
′
= lim
h→0
c o s ( x + h) − c o s x
h
= lim
h→0
−2 s in
x+h+x
2
s in
x+h−x
2
h
= lim
h→0
− s in ( x +
h
2
) s in
h
2
h
2
= − s in x.
D ow´
od wzoru 8.
S ko r z ys t a m y z e wz o r u n a p o c h o d n ¸a ilo r a z u .
( t g x)
′
=
s in
x
c o s x
′
=
( s in x)
′
c o s x − s in x( c o s x)
′
( c o s x)
2
=
c o s x c o s x − s in x( − s in x)
( c o s x)
2
=
c o s
2
x + s in
2
x
c o s
2
x
=
1
c o s
2
x
D ow´
od wzoru 9.
P o d o b n ie :
( c t g x)
′
=
c o s x
s in x
′
==
− s in x s in x − c o s x c o s x
( s in x)
2
= −
1
s in
2
x
.
D ow´
od wzoru 10.
P r z yp o m n ijm y, ˙ze
y = a r c s in x ⇔ x = s in y d la
y ∈ [−
π
2
,
π
2
] ( a wi¸e c
c o s y ≥ 0 c o o z n a c z a , ˙ze c o s y = +
1 − s in
2
y) .
Za s t o s u je m y t wie r d z e n ie o p o c h o d n e j fu n kc ji o d wr o t n e j.
( a r c s in x)
′
=
1
( s in y)
′
=
1
c o s y
=
1
1 − s in
2
y
=
1
√
1 − x
2
D ow´
od wzoru 11.
P r z yp o m n ijm y, ˙ze
y = a r c c o s x ⇔ x = c o s y d la
y ∈ [0 , π] ( a wi¸e c s in y ≥ 0 c o o z n a c z a , ˙ze s in y = +
1 − c o s
2
y) .

Za s t o s u je m y t wie r d z e n ie o p o c h o d n e j fu n kc ji o d wr o t n e j.
( a r c c o s x)
′
=
1
( c o s y)
′
=
1
− s in y
= −
1
1 − c o s
2
y
= −
1
√
1 − x
2
D ow´
od wzoru 12.
P r z yp o m n ijm y, ˙ze
y = a r c t g x ⇔ x = t g y.
Z t wie r d z e n ia o p o c h o d n e j fu n kc ji o d wr o t n e j:
( a r c t g x)
′
=
1
( t g y)
′
=
1
1
cos
2
y
=
c o s
2
y
s in
2
y + c o s
2
y
=
cos
2
y
cos
2
y
sin
2
y
cos
2
y
+
cos
2
y
cos
2
y
=
1
t g
2
y + 1
=
1
x
2
+ 1
.
D ow´
od wzoru 13.
P r z yp o m n ijm y, ˙ze
y = a r c c t g x ⇔ x = c t g y.
Z t wie r d z e n ia o p o c h o d n e j fu n kc ji o d wr o t n e j:
( a r c c t g x)
′
=
1
( c t g y)
′
=
1
−
1
sin
2
y
= −
s in
2
y
s in
2
y + c o s
2
y
= −
sin
2
y
sin
2
y
sin
2
y
sin
2
y
+
cos
2
y
sin
2
y
= −
1
1 + c t g
2
y
= −
1
1 + x
2
.
U W A GA . W
p r z yp a d ku , g d y x wys t ¸e p u je z a r ´o wn o w p o d s t a wie ja k i
w wykla d n iku , s t o s u je m y p r z e ks z t a lc e n ie
[f( x) ]
g(x)
= e
g(x) ln f (x)
.
P R ZY K L A D .
x
x
′
=
e
x ln x
′
= e
x ln x
( 1 · ln x + x ·
1
x
) = x
x
( ln x + 1 ) .
D E FIN ICJA . Je ˙ze li fu n kc ja f m a p o c h o d n ¸a i je ˙ze li fu n kc ja f
′
je s t t e ˙z
r ´o ˙zn ic z ko wa ln a , t o ( f
′
)
′
n a z ywa m y pochodn¸a drugiego rz¸edu fu n kc ji f i
o z n a c z a m y f
′′
. P o d o b n ie o kr e ´s la m y p o c h o d ¸a n-t e g o r z ¸e d u :
f
(n)
( x) =
f
(n−1)
( x)
′
.
P R ZY K L A D . Gd y f ( x) = x
3
+ e
2x
, t o d la
x ∈ R:
f
′
( x) = 3 x
2
+ 2 e
2x
, f
′′
( x) = 6 x + 4 e
2x
, f
′′′
( x) = 6 + 8 e
2x
,
f
(4)
( x) = 1 6 e
2x
, f
(5)
( x) = 3 2 e
2x
, ...
Za t e m , f
(n)
( x) = 2
n
e
2x
d la
n ≥ 4 .
P R ZY K L A D . Gd y f ( x) = ln ( 1 + x) , t o d la
x ∈ ( −1 , ∞) :
f
′
( x) =
1
1+x
= ( 1 + x)
−1
, f
′′
( x) = −1 ( 1 + x)
−2
,
f
′′′
( x) = ( −1 ) ( −2 ) ( 1 + x)
−3
= 2 ( 1 + x)
−3
,
f
(4)
( x) = ( −1 ) ( −2 ) ( −3 ) ( 1 + x)
−4
= −6 ( 1 + x)
−4
, ...
Za t e m
f
(n)
( x) = ( −1 )
n−1
( n − 1 ) !( 1 + x)
−n
.
Wyszukiwarka
Podobne podstrony:
janusz w 03 2010d zast poch
02, Pisanie własnych funkcji
02 Pisanie własnych funkcji
02 OGOLNE WLASNOSCI FUNKCJI, szkola technikum, matma, mata, zadania z liceum
Ciągi liczbowe i poch funkcj IŚ
janusz w 04 2010d bad przeb zm funk
02 Określanie warunków funkcjonowania człowieka 2
Budowa i funkcja genomu ludzkiego 02
02 Minimalizacja funkcji logicznyc (2)
02 Wybrane metody numeryczne (aproksymacja funkcji, rozwiazy
04 Rozdział 02 Różniczkowanie funkcji wielu zmiennych
02 Pochodna funkcji o dziedzinie jednowymiarowej (2)
Funkcje intrapersonalne, 02.ROZWÓJ OSOBISTY +.....), 01.Psychologia ; Rozwój osob.;NLP..itp, Psychol
02 pojecie, funkcje, konstrukcje i klasyfikacja podatkow, technika podatkowa, oplatyid 3727 ppt
02 Funkcje, pochodne, calkiid 3 Nieznany (2)
02 Twierdzenie o Funkcji Uwikłanej
Ćw 02 Rysowanie podstawowych obiektów graficznych – funkcje paska „Rysuj”
więcej podobnych podstron