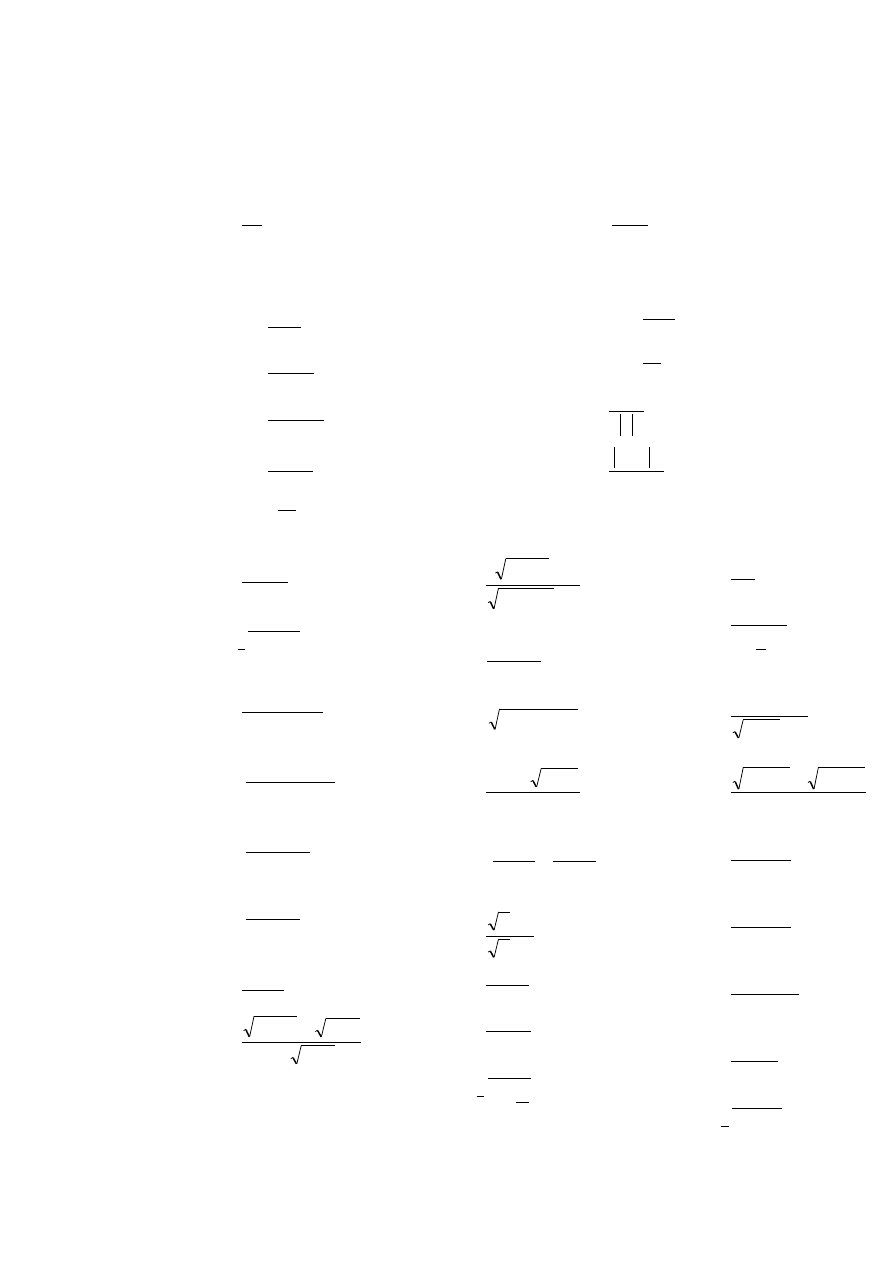
Lista zadań nr 4.
Strona 1 z 2
4.GRANICA FUNKCJI.
4.1. Wykazać, że:
a)
7
1
3
lim
2
x
x
b)
2
0
1
lim
x
x
c)
0
0
lim
a
a
x
x
d)
1
log
lim
a
x
a
x
e)
x
x
3
1
lim
3
4.2. Obliczyć granice jednostronne funkcji w punkcie:
a)
1
,
1
1
x
x
x
f
b)
2
,
4
2
2
x
x
x
x
f
c)
2
,
2
2
2
x
x
x
f
d)
3
,
9
3
2
x
x
x
f
e)
2
,
2
2
1
x
x
f
x
f)
2
,
3
2
2
1
x
x
f
x
g)
3
,
3
2
x
e
x
f
x
h)
x
x
x
sin
lim
0
i)
2
3
3
1
1
lim
x
x
x
x
4.3. Obliczyć:
a)
2
4
lim
2
2
x
x
x
b)
1
2
1
4
lim
2
2
1
x
x
x
c)
6
2
3
4
lim
2
3
x
x
x
x
d)
20
8
8
2
lim
2
2
2
x
x
x
x
x
e)
50
2
125
lim
2
3
5
x
x
x
f)
32
2
lim
5
2
x
x
x
g)
1
1
lim
5
1
x
x
x
h)
1
1
1
1
lim
2
0
x
x
x
x
i)
5
25
1
1
lim
2
2
0
x
x
x
j)
1
3
3
2
lim
x
x
x
x
k)
x
x
x
x
1
2
lim
2
l)
1
2
1
lim
2
1
x
x
x
x
m)
2
2
1
1
2
1
3
lim
x
x
x
n)
1
1
lim
3
4
1
x
x
x
o)
x
x
x
4
3
sin
lim
0
p)
x
x
x
2
sin
4
lim
0
q)
2
cos
lim
2
x
x
x
r)
x
tgx
x
4
lim
0
s)
x
x
x
8
1
sin
8
lim
8
t)
2
4
5
sin
lim
0
x
x
x
u)
x
tgx
tgx
x
2
sin
1
1
lim
v)
2
0
cos
1
lim
x
x
x
w)
x
x
arctg
x
6
2
lim
0
x)
x
x
x
3
arcsin
2
lim
0
y)
2
0
5
sin
lim
x
x
x
x
z)
x
tgx
x
x
2
cos
lim
4

Lista zadań nr 4.
Strona 2 z 2
aa)
2
1
1
1
sin
lim
x
x
x
bb)
x
ctg
x
x
5
3
sin
lim
0
cc)
x
x
x
2
1
lim
0
dd)
x
x
x
3
2
cos
1
sin
lim
ee)
1
4
2
1
arcsin
lim
2
2
1
x
x
x
ff)
x
x
x
x
2
1
3
2
3
lim
gg)
2
sin
2
lim
x
x
x
hh)
x
x
x
x
1
0
4
1
lim
ii)
e
x
x
e
x
3
ln
lim
3
Wyszukiwarka
Podobne podstrony:
Lista zadan nr 1 z matematyki dyskretnej
Lista zadan nr 2
Lista zadan nr 4 z matematyki dyskretnej
Lista zadan nr 2 z matematyki dyskretnej
Lista zadań nr 3
Lista zadan nr 3
lista zadan nr 6
Lista zadań nr 3
Lista zadań nr 4
Lista zadan nr 6 z matematyki dyskretnej
Lista zadań nr 4
SNA3 lista zadań nr 1 H
Lista zadan nr 3
Lista zadan nr 1
Lista zadan nr 1
Lista zadan nr 2
Lista zadań nr 2
lista zadan nr 6
Lista zadan nr 5
więcej podobnych podstron